ABSTRACT We review recent studies of cavity switching induced by the optical injection of free carriers in micropillar cavities containing quantum dots. Using the quantum dots as a broadband

internal light source and a streak camera as detector, we track the resonance frequencies for a large set of modes with picosecond time resolution. We report a record-fast switch-on time

constant (1.5 ps) and observe major transient modifications of the modal structure of the micropillar on the 10 ps time scale: mode crossings are induced by a focused symmetric injection of

free carriers, while a lifting of several mode degeneracies is observed when off-axis injection breaks the rotational symmetry of the micropillar. We show theoretically and experimentally

Moreover, the control of QD-mode coupling on the 10 ps time scale establishes cavity switching as an appealing resource for quantum photonics. SIMILAR CONTENT BEING VIEWED BY OTHERS CAVITY

FLOQUET ENGINEERING Article Open access 06 September 2024 FEW-PHOTON ALL-OPTICAL PHASE ROTATION IN A QUANTUM-WELL MICROPILLAR CAVITY Article 16 June 2022 CAVITY-MEDIATED LONG-RANGE

INTERACTIONS IN LEVITATED OPTOMECHANICS Article Open access 01 March 2024 INTRODUCTION In the context of the blossoming development of nanophotonics, photonic micro and nanostructures have

become powerful tools to tailor the optical properties of semiconductor emitters in solid-state cavity quantum electrodynamics (CQED) experiments1,2,3,4,5. The strong coupling regime has

been observed for quantum wells in planar cavities6, and for a single quantum dot (QD) coupled to a single-cavity mode5. In the weak coupling regime, the enhancement1,3,7 and the

inhibition2,8,9,10 of the spontaneous emission (SpE) rate have been observed for QDs. These drastic modifications of the emitter’s behavior result from the engineering of the modes of the

electromagnetic field and of the emitter-mode coupling. These key fundamental advances have fueled the development of a novel class of optoelectronic devices exploiting CQED effects on a

single QD, including single-mode sources of single photons1,3,4,11,12,13,14,15 or sources of entangled photon pairs16,17. Although solid-state CQED has initially been inspired by pioneering

atomic-CQED, there is still a major difference between these two classes of experiments. In atomic-CQED, one can control the interaction time between a flying atom and a cavity mode by

varying the atom velocity18. By contrast, the vast majority of solid-state CQED experiments to date are “static” experiments, during which the emitter-mode coupling is kept constant for the

entire duration of SpE events1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17. In the quest for “dynamic” solid-state CQED, several groups have recently proposed strategies to rapidly modify the

emitter-field coupling, “rapidly” meaning within a time that is shorter than the relevant time scale for the static coupled system (emitter lifetime in the weak coupling regime, Rabi

oscillation period in the strong coupling regime). One can for instance induce ultrafast modifications of the local density of optical states (LDOS) at the emitter location using short laser

pulses to change the refractive index of the constituent material. It is possible in principle to change the spectral detuning of an emitter with respect to the band-edge of a photonic

crystal19,20,21,22,23,24 or to a discrete cavity mode25. In a long-term perspective, a dynamic control of CQED effects is obviously attractive, e.g., to switch-on and -off Rabi oscillations,

and prepare Fock states in cavity modes26, in a way that would resemble the operation of micromasers based on flying Rydberg atoms18. It could also be used to tune the magnitude of the

Purcell effect and tailor the temporal envelope of single-photon pulses. This capability is highly desirable in view of applications to photonic quantum computing and simulations27 and

quantum state transfer through photon exchange28. Various approaches towards a fast control of emitter-cavity detuning have been explored. The electrostatically induced Stark effect has been

considered for trapped ions29, as well as QDs30,31, for adjusting the emitter frequency with respect to a target cavity mode. In the latter case, its dynamical range happens to be limited

to the sub-GHz range31, which is not sufficient for controlling CQED effects on QDs. One can alternatively tune the frequency of a mode with respect to a target emitter. Transient changes of

the frequency of cavity modes on the few-10 ps time scale have been induced in planar and photonic crystal cavities using pulses of acoustic waves32,33. All-optical cavity switching

provides an even faster and more versatile means. The reversible frequency switching of cavity modes can be achieved on a sub-picosecond time scale using the electronic Kerr effect34,35,36.

The mainstream approach, though, relies on the optical injection of free carriers using a pulsed laser pump to change the refractive index of the semiconductor layer forming the

microcavity37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52. While an ultrafast “switch-on” behavior is observed on the few-ps time scale, the relaxation of the mode towards its original

frequency is usually slower, as it is governed by free carrier recombination. Nevertheless, “switch-off” time constants in the sub-100 ps range can be obtained in small cavities thanks to

fast non-radiative recombination at etched surfaces, fast diffusion out of the cavity mode50, or by engineering the bulk non-radiative recombination rate (e.g., by growing parts of the

semiconductor microcavity at a lower-than-standard substrate temperature49,51). This latter switching scheme is very well suited for the dynamic control of QD–cavity systems in the weak

coupling regime, since the free carrier lifetime ranges between a few tens of ps and a few ns. A reversible change of the SpE rate of a QD in a photonic crystal cavity on the 200 ps time

scale was recently demonstrated using this approach47. The ultrafast injection of free carriers is exploited in various contexts to change cavity mode properties, such as their frequency, in

view of color-change experiments39,40,41,42,43, the cavity Q, to catch and release light pulses44, the coupling and photon exchange between distant cavities45, or the modes field

distributions in coupled cavity systems46,47. We review in this paper recent advances towards dynamic solid-state CQED, which have been obtained by the PHELIQS laboratory in Grenoble, using

GaAs/AlAs pillar microcavities containing InAs QDs—dubbed as quantum dot-micropillars (QDMs)—as main workhorse, and optical injection of free charge carriers as cavity switching method. For

all results presented in this review, we use micropillars containing a one-wavelength-thick GaAs cavity layer, surrounded by two distributed Bragg reflectors (15 periods on the top side, 25

periods on the bottom side). A detailed presentation of the QDM samples used for experiments is given in the Supplementary Information (SI). We first show in section “Probing switching

events with QDs as an internal light source” that QD ensembles can be used as a broadband internal source to light up all cavity modes during switching events48. Using a detection set-up

combining a spectrometer and a streak camera, we track the frequencies of many cavity modes in parallel, with a 2 ps time resolution. This fast and convenient characterization method enables

extensive studies of the switching behavior as a function of the parameters of the laser pump (wavelength, pulse energy, beam shape). For conditions that ensure a spatially uniform

refractive index change, novel insights are obtained on the dynamics of the switching behavior of the fundamental mode after a pump pulse. Under non-uniform carrier injection, using a

focused pump beam, mode-dependent switching behaviors are observed. Amazing transient features are witnessed, noticeably a reordering of the modes of the micropillar under symmetric carrier

injection respective to the pillar axis, and a lifting of the polarization degeneracy of some modes when one uses an off-axis carrier injection to break the circular symmetry of the pillar

microcavity. A model taking into account free carrier diffusion and recombination gives a good quantitative modeling of the markedly different switching behaviors for a large set of cavity

modes. Section “Controlling SpE dynamics using cavity switching: insights from theory” is devoted to the presentation and theoretical modeling of two possible applications of cavity

switching in the field of quantum photonics. By inducing a transient coupling between an emitter and a cavity mode, one can switch on and off the Purcell effect in a fast way. The system

then generates a SpE burst whose duration is much shorter than the lifetime of the uncoupled emitter. On the basis of a rate equation analysis, we introduce and compare possible signatures

of “Purcell switching” in such experiments. However, besides on/off switching, one could also play more smoothly on the detuning between the emitter and a cavity mode. Solving quantum

Langevin equation, we show that single-photon pulses with tailored temporal envelopes can be produced by state-of-the-art QD–cavity systems with a high efficiency and a high fidelity with

respect to the target pulse53. In section “Preparation of ultrashort low-coherence pulses of SpE using switched QDM”, we use cavity switching to tune on and off, and on the few-ps time

scale, the coupling between QDs and the fundamental mode of a pillar microcavity. In these experiments, a set of spectrally selected QDs enters into resonance with the fundamental mode, as

it relaxes towards its original frequency after a switch. This approach is used to generate pulses as short as 6 ps54,55. Unlike laser pulses, these short SpE pulses display a very weak

spectral coherence. Finally, we briefly discuss some perspectives opened by this work in section “Some perspectives related to this work”. PROBING SWITCHING EVENTS WITH QDS AS AN INTERNAL

LIGHT SOURCE Practical applications of cavity switching in the field of quantum photonics require a detailed knowledge of the amplitude and dynamics of the frequency shifts of the modes.

Since the first switching studies, performed on micropillars in the '80s in the context of the development of bistable optical devices37,38, ultrafast pump–probe spectroscopy in

reflection geometry has been the reference technique for probing switching events34,35,36,49,50,51,52. This technique provides excellent time resolution56, but is relatively cumbersome (two

synchronized tunable lasers are needed), and relatively slow due to the pointwise exploration of the 2D frequency-time space, which hinders extensive parametric studies. Furthermore, one is

able to probe by transmission or reflection spectroscopy only modes that have a non-zero overlap with the probe beam. As an example, for a Gaussian beam whose waist is matched to the top

facet of a micropillar, one only detects the fundamental cavity mode in the reflection or transmission curves57. Using a tightly focused beam with a waist smaller than the cavity, alleviates

this constraint to some extent, and permits probing additional modes58,59. It is nowadays well known that one can systematically study all modes of a static cavity in a simple

photoluminescence (PL) experiment, by using a collection of embedded QDs as a broadband internal light source1,60. In the SpE regime, QDs behave as independent point-like emitters and light

up systematically all cavity modes within the broad frequency range covered by their inhomogeneously broadened emission line. Several attempts have been made to adapt this approach to the

characterization of switched single-mode photonic crystal cavities61 and micropillars55. In these experiments, the same laser pulse was used both to excite embedded InAs QDs and to switch

the cavity modes through free carrier injection in GaAs layers. There is in turn some delay (~25 ps) in the onset of the QD PL due to carrier capture and relaxation processes. The PL is too

weak for studying the switch-on behavior of the cavity modes during the first ten to fifteen picoseconds after the pump pulse. We have recently improved this approach by introducing a

two-pulse pumping sequence to ensure that the internal light source is bright enough to probe the entire switching event. As detailed in Sattler et al. 48, we use a fibered system to split

the pulses delivered at 76 MHz by a Ti:sapphire laser into a weak pulse, which lights up the QDs but does not induce significant switching, and a stronger one, which is delayed with respect

to the first one and switches the cavity. We use a detection set-up combining a grating spectrometer and a streak camera to track in parallel the time-dependent frequencies of many resonant

modes, over the entire switching event with a ps time resolution. QD growth conditions are chosen so that the QD ensemble emission is centered at around 1.36 eV, with a 45 meV full-width at

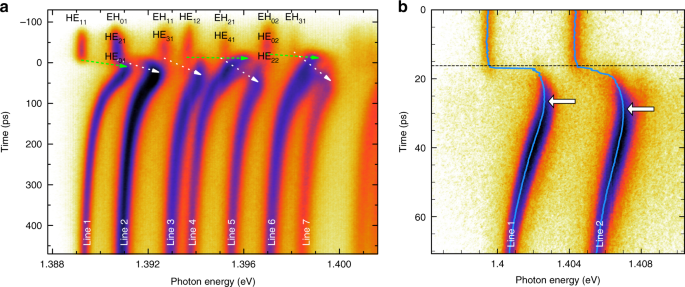
half-maximum (FWHM). This emission range is well suited for an efficient detection by the photocathode of our streak camera (see SI). We display in Fig. 1 two streak camera images, which

illustrate the complementarity of experiments performed over different time ranges (TR). Using a 600 ps-long TR, one can capture the entire event including full relaxation of the modes.

Before the pump pulse at around _t_ = 0, the frequencies of the emission lines do not change, which confirms that possible small switching effects induced by the previous pulse have faded

away. These frequencies correspond therefore to the ones of the modes of the unswitched QDM, as observed in cw PL experiments, and serve as references to evaluate switching effects. Emission

lines are labeled using the standard names of all pillar modes, which contribute to each line60. At around _t_ = 0, the injection of free carriers induces locally a negative change of the

refractive index of GaAs layers. As a consequence, all lines experience a blue shift, within less than 30 ps, albeit with significantly different switching amplitudes and time delays before

reaching the maximum shift. Operating in this TR, the time resolution is not high enough to track precisely the modes during the switch-on. After around 100 ps, all lines relax back towards

their reference frequency and exhibit a common behavior, which can be described by an exponential law, with a 200 ps characteristic time constant that corresponds to the decay time of the

population of free electron–hole pairs. By contrast, our set-up has a time resolution better than 2 ps when using the streak camera in its shortest TR mode, which enables probing the

ultrafast transient effects that occur during and just after the switch, as shown by Fig. 1b and other examples to be presented below. One can easily explain switching effects in a

qualitative way by considering the evolution of the population of free carriers and their impact on the transverse refractive index profile in the micropillar. Since the pump photon energy

_E__p_ is above the GaAs bandgap (~1.52 eV at 4 K), charge carriers are photogenerated with excess energy in the conduction and valence bands of GaAs. They then relax within a few ps62 and

provide a maximal change of the refractive index once relaxation toward the band extrema of GaAs is completed63. Further evolution of the distribution of free carriers is governed by lateral

diffusion on the few-10 ps scale and carrier recombination. While population decay at long times is always dominated by non-radiative recombination at etched sidewall surfaces, radiative

recombination of electrons and holes can contribute to it at shorter delays, when the free carrier density is the largest. Being both fast and precise, this characterization method makes

parametric studies much easier than previous techniques. It allows, for instance, the detailed study of the switch-on dynamics by analyzing images such as that shown in Fig. 1b. By

optimizing the pump power, we could observe a rise-time constant around 1.5 ps48, significantly smaller than previously reported values49,50,51,52. This is obviously interesting in view of

the ultrafast control of the spectral detuning between an artificial atom and one- or few-cavity modes. One can also easily explore the huge impact of the spatial characteristics of the pump

beam (i.e., beam diameter and position with respect to the pillar axis) on switching features. In micropillars, the resonant modes have amazingly different transverse field distributions as

shown in Fig. 2. For instance, the fundamental mode HE11, which gives rise to Line 1 in Fig. 1b has no node and its antinode is on the pillar axis. By contrast, all modes contributing to

Line 2, also shown in Fig. 1, have a donut shape and zero intensity on the pillar axis. These differences, which are inherited from the properties of guided modes in cylindrical

waveguides64, and are well known from theory, have also been clearly confirmed by mode intensity mapping, using reflection spectroscopy with a tightly focused white beam59. Consequently, one

observes drastically different switching behaviors depending on the size and location of the pump beam with respect to the pillar top facet. If one uses a pump beam much broader than the

pillar diameter, uniform carrier injection is realized and all modes display the same switching amplitude and the same switching dynamics48. On the opposite, one can observe mode-specific

switching behaviors for a non-uniform carrier injection. As first shown by pump-probe reflection spectroscopy experiments52, and as confirmed by the time-resolved PL results shown in Fig.

1b, Lines 1 and 2 behave differently when the pump spot matches the pillar top facet. Thanks to a better overlap between the Gaussian initial distribution of free carriers and the mode

profile, Line 1 exhibits a slightly larger switching amplitude and a faster switch-on dynamics. For Line 2, the largest switching amplitude is only obtained after lateral free carrier

redistribution, which induces some delay (see red arrows in Fig. 1b). One can expect more spectacular “differential switching” features when the pump spot is much smaller than the pillar top

facet. For a focused and centered pump beam, we expect to observe (according to mode perturbation theory, see, e.g., Vassalo65) a highly sensitive and selective response of the modes whose

main antinode is located on the pillar axis. Within the set of modes shown in Fig. 2a (giving rise to Lines 1–5) this is only the case for the modes HE1_n_; additionally, the field is more

concentrated around the pillar axis for HE12 than for HE11, which should warrant to HE12 the highest sensitivity to optically induced refractive index changes. This is confirmed by the

numerical simulation of the expected mode blue shifts after excitation by a focused Gaussian beam, which are plotted in Fig. 2b as a function of the maximum index change, induced in GaAs at

the center of the pump spot. HE12 is the most sensitive to such a perturbation and shifts twice as much as the fundamental mode HE11. We assume for these calculations a linear dependence of

the refractive index change as a function of the carrier density, which is reasonable approximation for carrier densities62 above 1017 cm−3 that are typically used in switching experiments.

Since the switch does not break circular symmetry, it does not induce any coupling between modes that have different symmetry degrees (i.e., for instance different azimuthal orders _m_, or

different behaviors with respect to a mirror symmetry). As shown in Fig. 2b, one expects therefore to observe mode crossings (instead of anti-crossings) when the perturbation strength is

increased. This is noticeably the case for the highly sensitive mode HE12 (related to Line 4), which crosses the weakly sensitive donut-shape modes EH11 and HE31 (which give rise to Line 5)

for | Δ_n_ | > 0.1. This theoretical prediction is validated through the observation of a transient re-ordering of resonant pillar modes just after the switch in several experiments. In

Fig. 1a, we see that Lines 4 and 6 (both related to modes with a large field intensity close to the pillar axis) experience a large switch within few ps. For Line 4, the shift is larger than

the separation of Lines 4 and 5 before the switch. This is also the case for the shift of Line 6, when compared to the separation of Lines 6 and 7. By contrast, Lines 5 and 7, related to

donut-shape modes, exhibit a relatively slow shift that reaches its maximum only after several tens of picoseconds, once free carriers have been fully redistributed laterally by diffusion.

So, considering the spectral positions of Lines 4 and 6 before and after the switch, as well as the slow behavior of Lines 5 and 7, we can conclude that Lines 4 and 5, as well as Lines 6 and

7, have to cross twice at short delays. We have recently attempted to study such a behavior over a narrower TR, so as to track precisely the modes during the reordering event. As shown by

Fig. 2c, Lines 4 and 5 overlap about 10 ps after the switch, thanks to the large and fast switch of Line 4 (please see also Fig. S7 in the SI, showing the raw streak camera image). Due to

free carrier diffusion away from the center of the pillar, Line 4 experiences a fast relaxation at longer delays, which restores the standard ordering and spectral separation of the modes.

We do not observe any sign of anti-crossing behavior when Lines 4 and 5 overlap, in agreement with numerical simulations (see, e.g., Fig. 2b). We also show in Fig. 2c the calculated

evolution of the mode frequencies during the switch, taking into account inhomogeneous carrier injection, carrier diffusion and recombination. This modeling, which gives a satisfying

description of the temporal evolution of all modes at all times, suggests that Lines 4 and 5 actually cross and that a reverse ordering is obtained during about 10 ps. In this experiment,

however, the broadening of the lines is similar to their frequency difference, which hinders a clear observation of the mode crossing and reordering. Experiments on higher-_Q_ micropillars

are under way to go beyond this promising but still preliminary result. Additionally, one can use an off-axis focused injection of free carriers to break the circular symmetry of the

micropillar48. The result of such an experiment is shown in Fig. 3 for a 5 µm diameter QDM. Clear transient splittings are observed during around 20 ps after the switch for Lines 2 and 6,

due to the shift from circular symmetry to planar symmetry. This amazing effect vanishes as soon as carrier diffusion restores the circular symmetry. The transient effects observed in Figs.

2c and 3 can be reproduced in detail for all modes by numerical simulations taking into account the carrier injection geometry, as well as carrier diffusion and recombination48,52.

Importantly, such simulations rely on a single fitting parameter, Δ_n_, which is the maximum refractive index change of GaAs layers, induced just after the pulse at the center of the pump

spot. On the basis of this detailed understanding of switching events in in QDM, possible applications of cavity switching in the context of quantum photonics will be introduced in the

following two sections. CONTROLLING SPE DYNAMICS USING CAVITY SWITCHING: INSIGHTS FROM THEORY Solid-state optical microcavities such as micropillars and microdisks are not perfect “photonic

dots”, as, besides the discrete resonant cavity modes, the continuum of non-resonant modes of the environment contributes to the LDOS inside the cavity. In the weak coupling regime,

well-known benefits come from the coupling between an artificial atom such as a QD and a (static) cavity mode whose Purcell factor _F__p_ is large enough (_F__p_ > 1)1,2,3,4,7. The SpE

rate into the cavity mode is selectively enhanced, while SpE into non-resonant modes is basically unchanged. On one side, SpE is accelerated; this is the well-known Purcell effect. On the

other side, photons are preferentially funneled into the cavity modes. SpE becomes single mode for large values of _F__p_. If the cavity mode has a directional emission pattern, as for e.g.,

the fundamental mode of pillar cavities, this improves strongly the collection of emitted photons. These three beneficial effects are noticeably exploited in QD-micropillar sources of

single photons11,12,13,14,15. An artificial atom in a switched optical microcavity is expected to experience the Purcell effect in a transient way, as sketched in Fig. 4. In spite of its

simplicity, a rate equation analysis is very useful to figure out whether, and how strongly, signatures of Purcell enhancement are observable in such experiments25. We introduce here some

important lessons from rate equation modeling that will be used in section “Preparation of ultrashort low-coherence pulses of SpE using switched QDM” to support the analysis of our

experimental data. We consider a single QD that is embedded in a pillar microcavity and located at the antinode of the fundamental cavity mode, hereafter named mode _m_. The mode frequency

at rest is \(\omega _m^0\), the mode linewidth Δ_ω__m_ and the Purcell factor _F__p_. We assume that the collection set-up captures entirely the radiation pattern of mode _m_ in the

far-field (collection efficiency _η__m_ = 1) and a much weaker fraction _η__nr_ of the QD emission into the continuum of non-resonant modes. For sake of simplicity, we consider that mode _m_

is rapidly switched towards the frequency \(\omega _m^0 + S\) at around _t_ = 0 and that the QD is prepared into its excitonic state (for instance using π-pulse resonant pumping) at the

same moment. The QD bandgap is at frequency ωQD which belongs to the frequency interval [\(\omega _m^0,\omega _m^0 + S\)] that is swept by mode _m_ during its relaxation towards its original

frequency \(\omega _m^0\). Finally, we assume that free carrier recombination and mode relaxation follow a common exponential law, defined by the switch-off time constant _τ_off. With these

assumptions, the exciton population, _P__x__(t)_, the time-dependent SpE enhancement factor, _F(t)_, and the collected PL intensity, _I__s__(t)_, satisfy this following set of equations:

$${d}P_x/{d}t = - P_x \cdot \left( {\frac{\gamma }{{\tau _{b}}} + \frac{{F(t)}}{{\tau _{b}}}} \right)$$ (1) $$I_s(t) = \frac{{P_x}}{{\tau _{b}}} \cdot \left( {\gamma \eta _{{nr}} + F(t)\eta

_m} \right)$$ (2) $$F\left( t \right) = F_{p} \cdot \frac{{{{{\mathrm{{\Delta}}}}}\omega _m^2}}{{{{{\mathrm{{\Delta}}}}}\omega _m^2 + \left( {\omega _m\left( t \right) - \omega _{{QD}}}

\right)^2}}$$ (3) where \(\tau _{b}\) is the exciton lifetime for a QD embedded in bulk GaAs and \(\gamma /\tau _{b}\) the SpE of the exciton into the continuum of non-resonant modes. For

small-diameter micropillars in the 1–1.5 µm range, various _Q_’s ranging from 1000 up to 200,000 have been reported1,2,3,37,38,66,67, depending upon the numbers of GaAs/AlAs layer pairs

forming the Bragg reflectors and, more importantly, upon the roughness of the pillar sidewalls, which is induced by the etching step57. We choose for this numerical study experimentally

relevant parameters. We take as QD parameters \(\hbar \omega _{{QD}} = 1.36\;{{{\mathrm{eV}}}}\) and \(\tau _{b} = 1.3\;{{{\mathrm{ns}}}}.\) We fix the pillar diameter as 1.5 µm and its

resonance frequency at rest as \(\hbar \omega _m^0 = 1.358\;{{{\mathrm{eV}}}}\). We consider both moderate_-Q_ (_Q_ = 1000, _F__p_ = 7) and high_-Q_ (_Q_ = 20,000, _F__p_ = 136) micropillars

and take γ = 0.9. As discussed in Gayral et al. 68, a key parameter in the context of the analysis of the PL intensity is the set-up collection efficiency, \(\eta _{{nr}}\), for SpE that is

emitted into the background non-resonant modes. \(\eta _{{nr}}\) is usually very small for pillar microcavities, except for narrow pillar in the 1 µm diameter range, for which values around

0.1 have been reported. Larger values of \(\eta _{{nr}}\) can be obtained for microdisk and photonic crystal cavities, depending on the collection geometry of the experimental set-up. To be

more general, we chose therefore for this study \(\eta _{{nr}}\) equal to 0.1, 0.2, or 0.5. Finally, the switching amplitude is chosen to be _S_ = 6 meV and we assume for sake of simplicity

an exponential relaxation of the mode, with time constant \(\tau _{{off}}\) = 70 ps. With these parameters, one expects the perfect resonance between the QD and the shifting cavity mode to

occur around 80 ps. The most obvious signature of this transient coupling stems in the generation of a few-ps-long pulse of light by the QDM. The duration of this pulse

\({{{\mathrm{{\Delta}}}}}t\) is simply given by the ratio of the mode linewidth and the shifting speed of the mode in the frequency domain. For a single exponential decay, one gets

$${{{\mathrm{{\Delta}}}}}t = \frac{{\tau _{{off}}}}{Q} \cdot \frac{{\omega _m^0}}{{\omega _{{\mathrm{QD}}} - \omega _m^0}}$$ (4) Higher_-Q_’s lead to shorter interaction times, for a given

shifting speed of the mode. Additionally, the detuning between the QD and mode frequency at rest \(\omega _m^0\) appears as a simple tuning parameter for the pulse duration. The relaxation

becomes slower as the mode frequency gets closer to \(\omega _m^0\), which increases the interaction time for a QD close to \(\omega _m^0\). These properties will be exploited in section

“Preparation of ultrashort low-coherence pulses of SpE using switched QDM” to generate ultrashort SpE pulses. Another signature of the transient Purcell effect is the step-like behavior of

the excitonic population, observed at around 80 ps in Fig. 5a. This step results from the accelerated decay of _P__x_ induced by the QD-mode coupling. Its amplitude Δ_P__x_, which quantifies

also the probability for the QD to emit a photon in the mode during the transient coupling event, is approximately given by $${{{\mathrm{{\Delta}}}}}P_x = \frac{{F_{p}}}{{\tau _{b}}} \cdot

{\Delta}t = \frac{{F_{p}}}{Q} \cdot \frac{{\tau _{{off}}}}{{\tau _{b}}} \cdot \frac{{\omega _m^0}}{{\omega _{{QD}} - \omega _m^0}}$$ (5) Interestingly, Eq. 5 predicts that, for a given

pillar radius, Δ_P__x_ does not depend upon _Q_. This is confirmed by the simulation results shown in Fig. 5a, obtained for various _Q_’s (Δ_P__x_ is around 0.25, as predicted using Eq. 5).

If one increases the cavity _Q_, one increases the Purcell factor but one decreases by the same ratio the interaction time between the mode _m_ and the QD. In turn, the total SpE in the mode

is roughly independent of _Q_ (or _F__p_). Measuring the amplitude of the population step can be used to prove the involvement of the Purcell effect in a switching experiment, but is not an

adequate strategy if one aims at evaluating the maximum magnitude of the Purcell effect during the coupling event. In principle, the cleanest way for probing experimentally the population

decay is detecting selectively the emission into the background of non-resonant modes25. For in-plane emitting cavities, such as microdisks or cavities in 2D photonic crystals, one can

relatively easily collect this emission in normal incidence. This is not the case for micropillars, whose emission spectra are usually overwhelmingly dominated by the signal related to

cavity modes. In high-quality micropillars with smooth and straight sidewalls, most of the non-resonant emission is indeed guided in the GaAs/AlAs cylinder and escapes toward the substrate.

As a result, it is generally not possible to detect population changes in a PL experiment in normal incidence on switched QD-micropillars (SQDM) as shown in Fig. 5b, c. For moderate_-Q_

modes (_Q_ = 1000), emission at long delays comprises emission of the QD into the tails of the LDOS of the relaxed mode. In the high-_Q_ case (here 20,000), the observation of a clear drop

of the PL signal after the coupling event would require values of \(\eta _{{nr}}\) \(\left( {\eta _{{nr}}/\eta _m \,>\, 0.1} \right)\) that are obtainable for in-plane emitting cavities

but too large for high-quality micropillars. For these reasons, it is safer to use the enhanced amplitude of the PL signal as a witness of the Purcell effect in experiments on SQDM. When

compared to the decay of a far-detuned QD that would not be coupled to any mode, the PL intensity is typically enhanced by a factor $$1 + \frac{F}{\gamma }\frac{{\eta _m}}{{\eta _{{nr}}}}$$

(6) We recognize in this formula two combined benefits of the Purcell effect: SpE acceleration \(\left( {F \,>\, \gamma } \right)\) and SpE funnelling into the cavity mode enabling better

collection of emitted photons \(\left( {\eta _m \,>\, \eta _{{nr}}} \right)\). A quantitative analysis of experimental data therefore requires prior knowledge of \(\eta _{{nr}}\). The

on–off switching of the Purcell effect could be used, in principle, to trigger the emission of a single photon at a chosen time, from a QD that has been previously prepared in its single

exciton state through resonant pumping. In the context of deterministic sources of indistinguishable single photons, this controlled delay would permit to filter-out the single-photons from

scattered pump light by time gating. This approach looks advantageous compared to present protocols that rely on cross-polarization schemes for the pump and single-photon collection

channels, and induce a strong penalty in terms of source efficiency14,15. Obviously, standard spectral filtering could be used to remove the (far-detuned) parasitic signals induced by the

switching beam such as scattered light or GaAs luminescence. Alternatively, non-local switching could also be implemented to ensure the purity of the single-photon pulses as discussed in

more detail in section “Some perspectives related to this work”. As already mentioned, the efficiency of such a triggered source of single photons is given by Eq. 5. It could be boosted up

to values close to unity by increasing the interaction time between the mode and the QD (while keeping _F__p_ constant), either by increasing _τ_off through improved surface passivation, or

more easily by decreasing the detuning \(\omega _{{QD}} - \omega _m^0\). Apart from micropillars, small volume cavities such as photonic crystal cavities look attractive in this context, as

they enable to get a larger _F__p_ for given values of _Q_ and of the interaction time. Although very useful in practice, a rate equation analysis does not allow to go beyond the study of

average exciton and photon populations. In the context of single-photon pulse shaping, refined modeling using quantum Langevin equation permits both to take into account decoherence effects

and to estimate key figures of merits such as the fidelity with respect to a target pulse or the factor of indistinguishability of the single photons53. Artificial atoms in a static photonic

environment experience an exponential decay. Therefore, single-photon wave packets have a strongly asymmetric temporal envelope, with a step-like rise and an exponential tail whose decay is

defined by the radiative lifetime. In the context of quantum photonics, such a shape is far from being optimal as shown by two emblematic examples. Firstly, it has been shown that Gaussian

pulses are the best suited for linear optics quantum computing, as they enable to minimize the impact of arrival time jitter on the fidelity of two-photon gate operations27. Secondly, the

asymmetric exponential envelope hinders perfect reabsorption by another emitter, even if it is strictly identical to the one that has emitted the single photon28. The success probability for

the teleportation of a quantum state using single photon exchange between two atoms is at most 63%. Perfect reabsorption requires mimicking the time-reversal of a SpE event; in other words,

the single photon should have an inversed exponential envelope as sketched in Fig. 6. A widely used approach toward single-photon time-envelope shaping is post-emission amplitude modulation

using electro-optical modulators69,70,71. In such experiments, pulse shaping is obtained at the price of some absorption, i.e., some reduction of the efficiency of the single-photon source.

We have shown theoretically53 that both Gaussian and inverse-exponential pulses could be emitted with both a high efficiency and high fidelity with respect to the target pulse using a

single QD in a high _F__p_ microcavity. For this, free carrier switching of the cavity mode would be used to tune in real time the QD-mode detuning, hence the magnitude of the Purcell

effect. The ultrafast response of cavity modes to all-optical free carrier injection is a key advantage, especially for the generation of inverse-exponential pulses, which is one of the most

demanding applications in terms of Purcell effect tuning53. One could naively think that changing the emitter frequency or the cavity mode frequency would lead, if both possible in

practice, to strictly equivalent results. As shown in Fig. 7, this is only true for the temporal envelope of the pulse. In the weak coupling regime, the emission of the coupled atom–cavity

system remains at all times close to the emitter frequency _ω_. As a result, emitter tuning leads to chirped single-photon pulses that are unsuitable to efficient reabsorption by another

atom30,53. For this very reason, cavity switching is by far superior to emitter tuning for single-photon pulse shaping. Unlike emitter tuning, cavity switching has a negligible influence on

the photon spectrum. It could allow, for realistic cavity parameters, to create Gaussian envelope single photons with a fidelity to the target photons of 99%, as well as single-photon with

time-reversed envelopes, enabling reabsorption by an atom in a cavity with a probability well above 90%53,72. PREPARATION OF ULTRASHORT LOW-COHERENCE PULSES OF SPE USING SWITCHED QDM As

shown in the previous section, one can use cavity switching to induce a transient coupling between an artificial atom such as a QD and a cavity mode. Due to Purcell enhancement of the SpE

rate into the cavity mode, the QD–cavity system emits a few-ps-long pulse of light. One can extend this approach to cavities containing ensembles of artificial atoms. This is obvious for

emitters such as rare-earth atoms73 or G-center defects in silicon74, whose ensembles exhibit a small inhomogeneous line broadening. By contrast, when emission wavelengths are dispersed, as

for self-assembled QDs75,76,77, one has to rely on the spectral selection of a sub-ensemble of emitters, emitting all within a chosen narrow spectral window. The duration of the SpE pulse is

then simply given by $${\Delta}t = \sqrt {{\Delta}\omega _m^2 + w^2} /\frac{{{d}\omega _m}}{{{d}t}}\left( {t_{{res}}} \right)$$ (7) in the general case, and $${\Delta}t =

^{\tau_{{off}}}/\left( {\omega _{{QD}} - \omega _m^0} \right) \cdot \sqrt {{\Delta}\omega _m^2 + w^2}$$ (8) when the relaxation of the mode is described by an exponential law, where _w_ is

the width of the frequency window, and _t__res_ the time at which the mode frequency _ω__m_ corresponds to the central frequency _ω__w_ of the collection window. We show in Fig. 8 the

results of a proof-of-principle experiment aiming at showing how very short pulses can be generated following this procedure. We study here a 1.6 µm-diameter micropillar, for which a

significant fraction of QD emission into non-resonant modes can be collected (_η__nr_ is around 5%, see SI). On the basis of Eqs. 4 and 6, we also choose switching conditions that provide a

large switching amplitude _S_, and therefore a large shifting speed for mode HE11 in the frequency domain. More precisely, we use a higher pump photon energy _E__p_ (1.7 eV), so as to

increase the density of electron–hole pairs in GaAs at saturation. A switching amplitude _S_ as large as 20 meV is observed (which corresponds to a relative frequency shift Δ_ω__m_/_ω__m_

larger than 1%). Unlike experiments reported in section “Probing switching events with QDs as an internal light source”, a single pump pulse is used in this experiment to prevent emission

from QDs prior to the switching pulse, and the emission behaviors of different collections of frequency-selected QDs, which do not enter (for window W1), or do enter (for windows W2 and W3),

into resonance with the mode during its relaxation towards its frequency at rest, are compared. We obtain the time-resolved PL profile for QDs in each window directly from the streak camera

image, through an integration at all times of the signal collected within that frequency window. As shown in Fig. 8, we observe, for all windows and for time delays between 0 and 15 ps, the

rise of the QD PL that is emitted into the continuum of non-resonant modes of the QDM. For W1, the PL signal decays in a monotonic way at longer time delays. Such a behavior is thus

qualitatively similar to the one of QDs in an unswitched micropillar (see SI). By contrast, the temporal PL profile exhibits a sharp additional peak (5 ps FWHM), at around a 25 ps delay for

window W2, due to the transient coupling of the QDs to the mode. When resonance is reached, the collected signal is increased typically by a factor of 3 with respect to the baseline of the

peak, which is related to emission into non-resonant modes. This specific feature reflects the on–off switching, on the few-picoseconds time scale, of the coupling between QDs emitting at

energies within W2 and the HE11 cavity mode. A similar behavior is observed for QDs emitting in window W3. However, the additional peak is observed at a longer delay after the pump pulse (30

 ps), and its duration is slightly longer (around 9 ps FWHM) than for W2. Since W3 is closer to the unswitched mode frequency \(\omega _m^0\) than W2, the mode enters indeed into resonance

with W3 at a later stage of its relaxation, and crosses window W3 at a reduced shifting speed. At this stage, we have not yet probed the nature of these short pulses of light. Since the QDM

contains many QDs (~ 6000 for a 2 µm diameter), besides SpE, stimulated emission could contribute to the generation of the light pulses when the mode enters into resonance with the QDs in

windows W2 or W3. We have therefore studied the temporal coherence of the pulses produced by the switched QDMs, to test whether SpE or stimulated emission is at play for their production. In

view of this complementary experiment, we use a different scheme for QD frequency-selection. We select here the emission of QDs within a chosen frequency window using bandpass filters. We

expect, according to the results of our proof-of-principle experiment, that a short light pulse escapes from the filter, each time the QDM is pumped. We confirm the successful preparation of

the pulse, and study its properties using the streak camera (see Fig. 9). In this experiment, the window width _w_ is around 3 meV, so that the pulse duration is around 16 ps. _w_ is also

larger than the mode linewidth (0.5 meV); in turn, light pulses display some chirp, because, within the frequency window, QDs at higher energy enter into resonance with the cavity mode

earlier than QDs at a lower energy. If only SpE is at play, all QDs within the spectrally selected sub-ensemble behave as independent emitters, each emitting at different frequencies.

Looking at Fig. 9, we see that the pulses are well above the Fourier limit (_w_ . Δ_t_ ≈ 100 » 1). Assuming they stem from the sum of uncorrelated SpE from numerous QDs spread all over the

collection window, one expects a coherence time \(\tau _{{coh}}^0\) shorter than 1/_w_ ≈ 0.12 ps and a coherence length of about 35 µm. (One has to note that these values give only upper

bounds for these quantities, since the coherence time is additionally reduced by the jitter on QD pumping, as well as dephasing processes that impact single QD emission78,79,80 under

non-resonant pumping conditions). We have probed in a qualitative way the coherence of the pulses emitted by switched QDMs by studying their transmission through a 500 µm Teflon film, used

as scattering medium. At around 1.4 eV, the photon mean free path is around 50 µm81, i.e., much smaller than the thickness of our film, so that light experiences many scattering events

before escaping at the exit side. For a large enough coherence length of the impinging beam (i.e., larger than the optical length difference for paths resulting from different scattering

events), one expects the formation of a 2D speckle pattern at the output facet of the scattering medium82. For this experiment, we use an imaging system based on a Si charge-coupled device

(CCD) camera to probe the appearance of speckles54 (see also SI). As a reference source, we use 3 ps long laser pulses at _ħω_ = 1.4 eV. Since _L_coh is much larger than the film thickness

for such laser pulses, a clear speckle pattern is observed54. This pattern is identical for two independent acquisitions, and does not depend upon pulse energies, as expected82. We perform

this experiment with the QDM light pulses, whose analysis is shown in Fig. 9. For this QDM, the switching amplitude _S_ is larger than 6 meV for pump powers larger than 10 pJ/pulse.

Therefore, light pulses can be prepared using the same frequency window and different pump intensities _P_ (here, ranging between 10 and 140 pJ/pulse). We show in Fig. 10 pairs of images

obtained from independent runs for two different _P_’s. In order to help us detect speckle formation, we use two numerical indicators. Denoting _C__nm_ the number of counts for the CCD pixel

(_n_,_m_) and \(\bar C\) the average number of counts, the standard deviation σ, which reflects intra-image fluctuations of the normalized pixel intensity, is given by $$\sigma ^2 = \mathop

{\sum }\limits_{nm} \left( {C_{nm} - \bar C} \right)^2/\bar C^2$$ We also define an inter-image mathematical distance _D_, to estimate how different two images are from one another: $$D^2 =

\mathop {\sum}\limits_{nm} {\left( {\frac{{C_{nm}}}{{\bar C}} - \frac{{C_{nm}^\prime }}{{\overline {C{^{\prime}} } }}} \right)^2}$$ where _C_ʹ_nm_ is the number of counts for pixel

(_n_,_m_), and \(\overline {C{^{\prime}} }\) the average value of _C_ʹ_nm_ for the second image. Trivially, _D_ = 0 for two identical images; more generally, for highly contrasted and

strongly correlated speckled images (large σ, small _D_), one expects _D/σ_ to become vanishingly small. By contrast, one can easily show that for unstructured images for which deviations of

pixel intensities with respect to the average value are only due to random noise (no inter-pixel correlations), then \(D/\sigma = \sqrt 2\). As shown in Fig. 10, this criterion is very

nicely satisfied for the pair of images obtained for _P_ = 60 pJ/pulse, as well as for smaller _P_’s. Additionally, σ is close to the expected value for inter-image intensity fluctuations

related to the shot noise. We can thus conclude that the light pulses that are prepared for _P_’s in the 10–60 pJ/pulse range are SpE pulses. This is additionally confirmed by the study of

the intensity _I_ of the light pulse, which shows a linear dependence as a function of the pump pulse energy _P_ within this power range. By contrast, we observe for this QDM a strong

non-linear dependence of the intensity of the emitted pulses as a function of _P_ for values above 70 pJ/pulse. We attribute this behavior to amplification by stimulated emission. At high

pump powers, one expects a saturation of the QDs’ ground states and a filling of their excited states as observed for reference QD layers grown under similar conditions (see SI). As the

frequency window W is located at around 1.41 eV, in the high-energy tail of the distribution of our QDs, this filling increases the number of QDs that emit within W and allows reaching the

lasing threshold. The transmission experiment through Teflon reveals a radically different behavior for pulses prepared in this strong pumping regime as shown in Fig. 10 for _P_ = 140 

pJ/pulse. CCD images resulting from different acquisitions look very similar and “hot spots” are clearly visible. Speckle formation is confirmed by considering the two criteria introduced

above. On one hand, the pixel to pixel intensity fluctuation _σ_ is, for both images, 250 times larger than the shot noise level. On the other hand, _D_/σ = 0.12 ≪\(\sqrt 2\), which

highlights the high degree of resemblance between these two highly contrasted images. These results show that the coherence length of SQDM pulses increases drastically when going from low to

high power pumping regimes, due to the onset of stimulated emission. The thresholds for amplification by stimulated emission and for speckle formation are precisely in line: coherence-free

pulses are only generated by SQDMs in the SpE regime, a crucial point for potential applications. In this experiment, we have shown that 16 ps-long pulses of SpE can be produced by

spectrally filtering the emission of a switched QDM. It is interesting to note at this point that short SpE pulses can of course be emitted by QD ensembles in static QDMs in the Purcell

regime. However, their duration cannot be shorter than around 25 ps, even for giant Purcell factors (_F__p_ > 100), because of the time jitter induced by carrier capture and relaxation

times under non-resonant pumping conditions83,84. Cavity switching appears as an utmost new approach to generate short light pulses in a controlled way. As an additional illustration, we

show in the SI that a wide range of pulse durations (from about 500 ps down to 5 ps), can be obtained using switched QDMs in the SpE regime, by simply varying the window detuning _ω__w_ − 

_ω°__m_. SOME PERSPECTIVES RELATED TO THIS WORK The results that have been presented in this paper open interesting perspectives in two main domains: ultrafast imaging and single-photon

pulse shaping for quantum photonics. The interest of low-coherence light pulses for imaging is well recognized, as they enable, e.g., detection of objects hidden by a turbid

environment85,86,87 or obtaining clear images of ultrafast processes in fluids88. Laser pulses are indeed poorly adapted to such applications, as their coherence induces diffraction fringes

and speckle patterns in recorded images. State-of-the-art low-coherence sources exploit few ns long fluorescence pulses emitted by a laser dye88. SQDMs could in principle be used to extend

ultrafast imaging to the sub-ns range, which is of interest for various domains (fluidics, shock wave propagation among others). At first sight, though, SQDMs suffer from their small power.

Their mere operation principle limits the average photon number per pulse to Δ_τ/τ_cav, so as to avoid stimulated emission, e.g., 10 photons/pulse for _Q_ = 1500 and Δ_t_ = 10 ps. By using

longer pulses (Δ_t_ ~ 200 ps) and operating a large array (100 × 100) of very similar SQDMs in parallel, one would reach 2 × 106 photons/pulse. Though challenging, this strategy is likely to

open a route towards the imaging in a stroboscopic mode of highly reproducible processes such as inkjet droplet formation88 on a sub-ns time scale. Thanks to its high repetition rate (76 

MHz here, with potential increase up to 5 GHz), a single SQDM could deliver up to 1011 photons/second. Therefore, the detection of objects hidden in turbid media such as fog or murky water

can be considered a realistic target in the short term. As mentioned in section “Controlling SpE dynamics using cavity switching: insights from theory”, cavity switching could be of interest

in the quest for efficient triggered sources of indistinguishable single photons. Furthermore, the shaping of the temporal envelope of indistinguishable single-photons emitted by single QDs

looks within reach in the mid-term using state-of-the-art optical microcavities. Nevertheless, major additional developments will be necessary to reach these goals. In order to avoid

repumping of the QD by carriers that are used for cavity switching (which would spoil the purity of the single-photon source), as well as dephasing induced by the Coulomb interaction of the

QD exciton with free carriers (which would plague single-photon indistinguishability), some kind of non-local switching has to be performed as shown by several groups on photonic crystal

cavities44,45,46,47. “Photonic molecules” made of coupled micropillars3 or coupled cavities in a photonic crystal membrane46,47 (or other extended cavities such as high-_Q_ ring-resonators)

are well suited for such experiments, where two synchronized lasers would be used to pump resonantly the QD on one side, and switch cavity modes (without pumping the region containing the

QD) on the other side. A local engineering of the non-radiative recombination rate (using for instance ion implantation) could be developed to warrant a fast switch-off dynamics. The method

introduced in section “Probing switching events with QDs as an internal light source” to characterize switching events might prove particularly helpful for the development of these

sophisticated photonic microstructures. REFERENCES * Gérard, J. M. Solid-state cavity-quantum electrodynamics with self-assembled quantum dots. In _Single Quantum Dots: Fundamentals,

Applications, and New Concepts_. _Topics in Applied Physics._ Vol. 90 (ed. Michler, P.) 269 (Springer, 2003)_._ * Noda, S., Fujita, T. & Asano, N. Spontaneous-emission control by

photonic crystals and nanocavities. _Nat. Photonics_ 1, 449–458 (2007). Article  ADS  Google Scholar  * Reitzenstein, S. Semiconductor quantum dot-microcavities for quantum optics in solid

state. _IEEE J. Sel. Top. Quantum Electron._ 18, 1733–1746 (2012). Article  ADS  Google Scholar  * Lodahl, P., Mahmoodian, S. & Stobbe, S. Interfacing single photons and single quantum

dots with photonic nanostructures. _Rev. Mod. Phys._ 87, 347–400 (2015). Article  ADS  MathSciNet  Google Scholar  * Reithmaier, J. P. Strong exciton–photon coupling in semiconductor quantum

dot systems. _Semicond. Sci. Technol._ 23, 123001 (2008). Article  ADS  Google Scholar  * Weisbuch, C. et al. Observation of the coupled exciton-photon mode splitting in a semiconductor

quantum microcavity. _Phys. Rev. Lett._ 69, 3314–3317 (1992). Article  ADS  Google Scholar  * Gérard, J. M. et al. Enhanced spontaneous emission by quantum boxes in a monolithic optical

microcavity. _Phys. Rev. Lett._ 81, 1110–1113 (1998). Article  ADS  Google Scholar  * Lodahl, P. et al. Controlling the dynamics of spontaneous emission from quantum dots by photonic

crystals. _Nature_ 430, 654–657 (2004). Article  ADS  Google Scholar  * Bleuse, J. et al. Inhibition, enhancement, and control of spontaneous emission in photonic nanowires. _Phys. Rev.

Lett._ 106, 103601 (2011). Article  ADS  Google Scholar  * Leistikow, M. D. et al. Inhibited spontaneous emission of quantum dots observed in a 3D photonic band gap. _Phys. Rev. Lett._ 107,

193903 (2011). Article  ADS  Google Scholar  * Gérard, J. M. & Gayral, B. Strong Purcell effect for InAs quantum boxes in three-dimensional solid-state microcavities. _J. Lightwave

Technol._ 17, 2089–2095 (1999). Article  ADS  Google Scholar  * Moreau, E. et al. Single-mode solid-state single photon source based on isolated quantum dots in pillar microcavities. _Appl.

Phys. Lett._ 79, 2865–2867 (2001). Article  ADS  Google Scholar  * Santori, C. et al. Indistinguishable photons from a single-photon device. _Nature_ 419, 594–597 (2002). Article  ADS 

Google Scholar  * Somaschi, N. et al. Near-optimal single-photon sources in the solid state. _Nat. Photonics_ 10, 340–345 (2016). Article  ADS  Google Scholar  * Ding, X. et al. On-demand

single photons with high extraction efficiency and near-unity indistinguishability from a resonantly driven quantum dot in a micropillar. _Phys. Rev. Lett._ 116, 020401 (2016). Article  ADS

  Google Scholar  * Liu, J. et al. A solid-state source of strongly entangled photon pairs with high brightness and indistinguishability. _Nat. Nanotechnol._ 14, 586–593 (2019). Article  ADS

  Google Scholar  * Wang, H. et al. On-demand semiconductor source of entangled photons which simultaneously has high fidelity, efficiency, and indistinguishability. _Phys. Rev. Lett._ 122,

113602 (2019). Article  ADS  Google Scholar  * Raimond, J. M., Brune, M. & Haroche, S. Manipulating quantum entanglement with atoms and photons in a cavity. _Rev. Mod. Phys._ 73, 565–582

(2001). Article  ADS  MathSciNet  MATH  Google Scholar  * Leonard, S. W. et al. Ultrafast band-edge tuning of a two-dimensional silicon photonic crystal via free-carrier injection. _Phys.

Rev. B_ 66, 161102 (2002). Article  ADS  Google Scholar  * Johnson, P. M., Koenderink, A. F. & Vos, W. L. Ultrafast switching of photonic density of states in photonic crystals. _Phys.

Rev. B_ 66, 081102 (2002). Article  ADS  Google Scholar  * Mazurenko, D. A. et al. Ultrafast optical switching in three-dimensional photonic crystals. _Phys. Rev. Lett._ 91, 213903 (2003).

Article  ADS  Google Scholar  * Becker, C. et al. Two-color pump-probe experiments on silicon inverse opals. _Appl. Phys. Lett._ 87, 091111 (2005). Article  ADS  Google Scholar  * Euser, T.

G. et al. Ultrafast optical switching of three-dimensional Si inverse opal photonic band gap crystals. _J. Appl. Phys._ 102, 053111 (2007). Article  ADS  Google Scholar  * Euser, T. G. et

al. All-optical octave-broad ultrafast switching of Si woodpile photonic band gap crystals. _Phys. Rev. B_ 77, 115214 (2008). Article  ADS  Google Scholar  * Thyrrestrup, H. et al.

Non-exponential spontaneous emission dynamics for emitters in a time-dependent optical cavity. _Opt. Express_ 21, 23130–23144 (2013). Article  ADS  Google Scholar  * Cosacchi, M. et al.

On-demand generation of higher-order Fock states in quantum-dot–cavity systems. _Phys. Rev. Res._ 2, 033489 (2020). Article  Google Scholar  * Rohde, P. P., Ralph, T. C. & Nielsen, M. A.

Optimal photons for quantum-information processing. _Phys. Rev. A_ 72, 052332 (2005). Article  ADS  Google Scholar  * Stobińska, M., Alber, G. & Leuchs, G. Perfect excitation of a

matter qubit by a single photon in free space. _Europhys. Lett._ 86, 14007 (2009). Article  ADS  Google Scholar  * Fernée, M. J., Rubinsztein-Dunlop, H. & Milburn, G. J. Improving

single-photon sources with Stark tuning. _Phys. Rev. A_ 75, 043815 (2007). Article  ADS  Google Scholar  * Johne, R. & Fiore, A. Single-photon absorption and dynamic control of the

exciton energy in a coupled quantum-dot–cavity system. _Phys. Rev. A_ 84, 053850 (2011). Article  ADS  Google Scholar  * Faraon, A. et al. Fast electrical control of a quantum dot strongly

coupled to a photonic-crystal cavity. _Phys. Rev. Lett._ 104, 047402 (2010). Article  ADS  Google Scholar  * Berstermann, T. et al. Optical bandpass switching by modulating a microcavity

using ultrafast acoustics. _Phys. Rev. B_ 81, 085316 (2010). Article  ADS  Google Scholar  * Fuhrmann, D. A. et al. Dynamic modulation of photonic crystal nanocavities using gigahertz

acoustic phonons. _Nat. Photonics_ 5, 605–609 (2011). Article  ADS  Google Scholar  * Ctistis, G. et al. Ultimate fast optical switching of a planar microcavity in the telecom wavelength

range. _Appl. Phys. Lett._ 98, 161114 (2011). Article  ADS  Google Scholar  * Eckhouse, V. et al. Kerr-induced all optical switching in a GaInP photonic crystal Fabry-Perot resonator. _Opt.

Express_ 20, 8524–8534 (2012). Article  ADS  Google Scholar  * Yüce, E. et al. All-optical switching of a microcavity repeated at terahertz rates. _Opt. Lett._ 38, 374–376 (2013). Article 

ADS  Google Scholar  * Jewell, J. L. et al. GaAs-AlAs monolithic microresonator arrays. _Appl. Phys. Lett._ 51, 94–96 (1987). Article  ADS  Google Scholar  * Kuszelewicz, R. et al.

Monolithic GaAs/AlAs optical bistable étalons with improved switching characteristics. _Appl. Phys. Lett._ 53, 2138–2140 (1988). Article  ADS  Google Scholar  * Notomi, M. & Mitsugi, S.

Wavelength conversion via dynamic refractive index tuning of a cavity. _Phys. Rev. A_ 73, 051803 (2006). Article  ADS  Google Scholar  * Gaburro, Z. et al. Photon energy lifter. _Opt.

Express_ 14, 7270–7278 (2006). Article  ADS  Google Scholar  * Preble, S. F., Xu, Q. F. & Lipson, M. Changing the colour of light in a silicon resonator. _Nat. Photonics_ 1, 293–296

(2007). Article  ADS  Google Scholar  * Preble, S. et al. Single photon adiabatic wavelength conversion. _Appl. Phys. Lett._ 101, 171110 (2012). Article  ADS  Google Scholar  * McCutcheon,

M. W. et al. Emission spectrum of electromagnetic energy stored in a dynamically perturbed optical microcavity. _Opt. Express_ 15, 11472–11480 (2007). Article  ADS  Google Scholar  * Tanabe,

T. et al. Dynamic release of trapped light from an ultrahigh-_Q_ nanocavity via adiabatic frequency tuning. _Phys. Rev. Lett._ 102, 043907 (2009). Article  ADS  Google Scholar  * Sato, Y.

et al. Strong coupling between distant photonic nanocavities and its dynamic control. _Nat. Photonics_ 6, 56–61 (2012). Article  ADS  Google Scholar  * Pellegrino, D. et al. Deterministic

control of radiative processes by shaping the mode field. _Appl. Phys. Lett._ 112, 161110 (2018). Article  ADS  Google Scholar  * Jin, C. Y. et al. Ultrafast non-local control of spontaneous

emission. _Nat. Nanotechnol._ 9, 886–890 (2014). Article  ADS  Google Scholar  * Sattler, T. et al. Probing microcavity switching events on the picosecond time scale using quantum dots as a

broadband internal fluorescent source. _APL Photonics_ 5, 126104 (2020). Article  ADS  Google Scholar  * Harding, P. J. et al. Dynamical ultrafast all-optical switching of planar GaAs/AlAs

photonic microcavities. _Appl. Phys. Lett._ 91, 111103 (2007). Article  ADS  Google Scholar  * Nozaki, K. et al. Sub-femtojoule all-optical switching using a photonic-crystal nanocavity.

_Nat. Photonics_ 4, 477–483 (2010). Article  ADS  Google Scholar  * Harding, P. J. et al. Observation of a stronger-than-adiabatic change of light trapped in an ultrafast switched GaAs-AlAs

microcavity. _J. Optical Soc. Am. B_ 29, A1–A5 (2012). Article  Google Scholar  * Thyrrestrup, H. et al. Differential ultrafast all-optical switching of the resonances of a micropillar

cavity. _Appl. Phys. Lett._ 105, 111115 (2014). Article  ADS  Google Scholar  * Hornecker, G. et al. Temporal shaping of single-photon pulses. _Proc. SPIE 9505, 9505N, Quantum Optics and

Quantum Information Transfer and Processing 2015_ (eds Banaszek, K. & Silberhorn, C.) (SPIE 2015). * Peinke, E. et al. Generation of ultrashort (~10 ps) spontaneous emission pulses by

quantum dots in a switched optical microcavity. Preprint at: https://arxiv.org/abs/1910.10518 (2019). * Sattler, T. et al. Cavity switching: A novel resource for solid-state quantum optics,

_2017 19th International Conference on Transparent Optical Networks (ICTON)_, 2017, pp. 1-4, https://doi.org/10.1109/ICTON.2017.8025177. * Euser, T. G., Harding, P. J. & Vos, W. L.

Broadband sensitive pump-probe setup for ultrafast optical switching of photonic nanostructures and semiconductors. _Rev. Sci. Instrum._ 80, 073104 (2009). Article  ADS  Google Scholar  *

Rivera, T. et al. Optical losses in plasma-etched AlGaAs microresonators using reflection spectroscopy. _Appl. Phys. Lett._ 74, 911–913 (1999). Article  ADS  Google Scholar  * Jewell, J. L.

et al. Transverse modes, waveguide dispersion, and 30 ps recovery in submicron GaAs/AlAs microresonators. _Appl. Phys. Lett._ 55, 22 (1989). Article  ADS  Google Scholar  * Ctistis, G. et

al. Optical characterization and selective addressing of the resonant modes of a micropillar cavity with a white light beam. _Phys. Rev. B_ 82, 195330 (2010). Article  ADS  Google Scholar  *

Gérard, J. M. et al. Quantum boxes as active probes for photonic microstructures: the pillar microcavity case. _Appl. Phys. Lett._ 69, 449–451 (1996). Article  ADS  Google Scholar  *

Fushman, I. et al. Ultrafast nonlinear optical tuning of photonic crystal cavities. _Appl. Phys. Lett._ 90, 091118 (2007). Article  ADS  Google Scholar  * Oudar, J. L. et al. Subpicosecond

spectral hole burning due to nonthermalized photoexcited carriers in GaAs. _Phys. Rev. Lett._ 55, 2074–2077 (1985). Article  ADS  Google Scholar  * Bennett, B. R., Soref, R. A. & Del

Alamo, J.A. Carrier-induced change in refractive index of InP, GaAs and InGaAsP. _IEEE J. Quantum Electron._ 26, 113–122 (1990). Article  ADS  Google Scholar  * Yariv, A. _Optical

Electronics_ 4th edn (Saunders College Publishing, 1991). * Vassallo, C. _Optical Waveguide Concepts_ (Elsevier, 1991). * Gregersen, N. et al. Numerical and experimental study of the _Q_

factor of high-_Q_ micropillar cavities. _IEEE J. Quantum Electron._ 46, 1470–1483 (2010). Article  ADS  Google Scholar  * Arnold, C. et al. Optical bistability in a quantum dots/micropillar

device with a quality factor exceeding 200 000. _Appl. Phys. Lett._ 100, 111111 (2012). Article  ADS  Google Scholar  * Gayral, B. & Gérard, J. M. Photoluminescence experiment on

quantum dots embedded in a large Purcell-factor microcavity. _Phys. Rev. B_ 78, 235306 (2008). Article  ADS  Google Scholar  * Kolchin, P. et al. Electro-optic modulation of single photons.

_Phys. Rev. Lett._ 101, 103601 (2008). Article  ADS  Google Scholar  * Specht, H. P. et al. Phase shaping of single-photon wave packets. _Nat. Photonics_ 3, 469–472 (2009). Article  ADS 

Google Scholar  * Rakher, M. T. & Srinivasan, K. Subnanosecond electro-optic modulation of triggered single photons from a quantum dot. _Appl. Phys. Lett._ 98, 211103 (2011). Article 

ADS  Google Scholar  * Peinke, E. T. _All-Optical Ultrafast Switching of Semiconductor Micropillar Cavities: Basics and Applications to Quantum Optics_. PhD thesis, Université Grenoble Alpes

(2016). * Weiss, L. et al. Erbium dopants in nanophotonic silicon waveguides. _Optica_ 8, 40–41 (2021). Article  ADS  Google Scholar  * Beaufils, C. et al. Optical properties of an ensemble

of G-centers in silicon. _Phys. Rev. B_ 97, 035303 (2018). Article  ADS  Google Scholar  * Marzin, J. Y. et al. Photoluminescence of single InAs quantum dots obtained by self-organized

growth on GaAs. _Phys. Rev. Lett._ 73, 716–719 (1994). Article  ADS  Google Scholar  * Grundmann, M. et al. Ultranarrow luminescence lines from single quantum dots. _Phys. Rev. Lett._ 74,

4043–4046 (1995). Article  ADS  Google Scholar  * Bimberg, D., Grundmann, M. & Ledentsov, N. N. _Quantum Dot Heterostructures_ (John Wiley, 1999). * Gérard, J. M. et al. Novel prospects

for self-assembled InAs/GaAs quantum boxes. _J. Cryst. Growth_ 201-202, 1109–1116 (1999). Article  ADS  Google Scholar  * Bayer, M. & Forchel, A. Temperature dependence of the exciton

homogeneous linewidth in In0.60Ga0.40As/GaAs self-assembled quantum dots. _Phys. Rev. B_ 65, 041308(R) (2002). Article  ADS  Google Scholar  * Berthelot, A. et al. Unconventional motional

narrowing in the optical spectrum of a semiconductor quantum dot. _Nat. Phys._ 2, 759–764 (2006). Article  Google Scholar  * Li, Q. H. et al. Light scattering of semitransparent sintered

polytetrafluoroethylene films. _J. Biomed. Opt._ 13, 054064 (2008). Article  ADS  Google Scholar  * Goodman, J. W. _Speckle Phenomena in Optics: Theory and Applications_ 2nd edn (SPIE Press,

2020). * Gayral, B. et al. Time-resolved probing of the Purcell effect for InAs quantum boxes in GaAs microdisks. _Appl. Phys. Lett._ 78, 2828–2830 (2001). Article  ADS  Google Scholar  *

Gérard, J. M. Prospects of high-efficiency quantum boxes obtained by direct epitaxial growth. In _Confined Electrons and Photons: New Physics and Applications_ (ed. Burstein, E. &

Weisbuch, C.) (Springer, 1995). * Mosk, A. P. et al. Controlling waves in space and time for imaging and focusing in complex media. _Nat. Photonics_ 6, 283–292 (2012). Article  ADS  Google

Scholar  * Rotter, S. & Gigan, S. Light fields in complex media: mesoscopic scattering meets wave control. _Rev. Mod. Phys._ 89, 015005 (2017). Article  ADS  Google Scholar  * Akhlaghi,

M. I. & Dogariu, A. Tracking hidden objects using stochastic probing. _Optica_ 4, 447–453 (2017). Article  ADS  Google Scholar  * van der Bos, A. et al. iLIF: illumination by

laser-induced fluorescence for single flash imaging on a nanoseconds timescale. _Exp. Fluids_ 51, 1283–1289 (2011). Article  Google Scholar  Download references ACKNOWLEDGEMENTS The authors

acknowledge gratefully the contributions of their coworkers to parts of these studies: A. Auffèves and G. Hornecker53, H. Thyrrestrup25,52, A. Hartsuiker25, E. Yüce and G. Csistis52, and A.

Gérard48. T.S. thanks the Laboratoire d’Excellence LANEF in Grenoble (ANR-10-LABX-51-01) for a PhD grant. G.M.T. and P.L.S. acknowledge funding from CAPES (PDSE 88881.187056 /2018.1) and

CNPq. This work was also partly funded by the FOM “Zap!” project, which is financially supported by NWO, NWO-Nano, and STW, as well as by NOMOS project, which is financed by ANR

(ANR-18CE24-0026). AUTHOR INFORMATION Author notes * These authors contributed equally: Emanuel Peinke, Tobias Sattler, Guilherme M. Torelly. AUTHORS AND AFFILIATIONS * Université Grenoble

Alpes, CEA, IRIG-PHELIQS, “Nanophysique et Semiconducteurs” group, F-38000, Grenoble, France Emanuel Peinke, Tobias Sattler, Guilherme M. Torelly, Sylvain Perret, Joël Bleuse, Julien Claudon

 & Jean-Michel Gérard * LabSem-CETUC, Pontifícia Universidade Católica do Rio de Janeiro, Rio de Janeiro, 22451-900, Brazil Guilherme M. Torelly & Patricia L. Souza * Complex

Photonic Systems (COPS), MESA+ Institute for Nanotechnology, University of Twente, P.O. Box 217, 7500 AE, Enschede, the Netherlands Willem L. Vos Authors * Emanuel Peinke View author

publications You can also search for this author inPubMed Google Scholar * Tobias Sattler View author publications You can also search for this author inPubMed Google Scholar * Guilherme M.

Torelly View author publications You can also search for this author inPubMed Google Scholar * Patricia L. Souza View author publications You can also search for this author inPubMed Google

Scholar * Sylvain Perret View author publications You can also search for this author inPubMed Google Scholar * Joël Bleuse View author publications You can also search for this author

inPubMed Google Scholar * Julien Claudon View author publications You can also search for this author inPubMed Google Scholar * Willem L. Vos View author publications You can also search for

this author inPubMed Google Scholar * Jean-Michel Gérard View author publications You can also search for this author inPubMed Google Scholar CORRESPONDING AUTHOR Correspondence to

Jean-Michel Gérard. ETHICS DECLARATIONS CONFLICT OF INTEREST The authors declare no competing interests. SUPPLEMENTARY INFORMATION 41377_2021_654_MOESM1_ESM.PDF Supplementary information for

tailoring the properties of quantum dot-micropillars by ultrafast optical injection of free charge carriers RIGHTS AND PERMISSIONS OPEN ACCESS This article is licensed under a Creative

Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the

original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in

the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended

use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit

http://creativecommons.org/licenses/by/4.0/. Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Peinke, E., Sattler, T., Torelly, G.M. _et al._ Tailoring the properties of quantum

dot-micropillars by ultrafast optical injection of free charge carriers. _Light Sci Appl_ 10, 215 (2021). https://doi.org/10.1038/s41377-021-00654-y Download citation * Received: 05

February 2021 * Revised: 06 September 2021 * Accepted: 12 September 2021 * Published: 19 October 2021 * DOI: https://doi.org/10.1038/s41377-021-00654-y SHARE THIS ARTICLE Anyone you share

the following link with will be able to read this content: Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer

Nature SharedIt content-sharing initiative