ABSTRACT This study aimed to evaluate the effect of dose reduction, by means of tube exposure reduction, on bone strength prediction from finite-element (FE) analysis. Fresh thoracic

mid-vertebrae specimens (n = 11) were imaged, using multi-detector computed tomography (MDCT), at different intensities of X-ray tube exposures (80, 150, 220 and 500 mAs). Bone mineral

density (BMD) was estimated from the mid-slice of each specimen from MDCT images. Differences in image quality and geometry of each specimen were measured. FE analysis was performed on all

specimens to predict fracture load. Paired t-tests were used to compare the results obtained, using the highest CT dose (500 mAs) as reference. Dose reduction had no significant impact on

with no impact on strength estimates obtained from FE analysis. Reduced CT dose will enable early diagnosis and advanced monitoring of osteoporosis and associated fracture risk. SIMILAR

CONTENT BEING VIEWED BY OTHERS GENDER DIFFERENCES IN L1 VERTEBRAL STRENGTH IN ADULTS 50+ USING AUTOMATED CT-BASED FINITE ELEMENT ANALYSIS Article Open access 28 March 2025 CONICAL SHELL

X-RAY BEAM TOMOSYNTHESIS AND MICRO-COMPUTED TOMOGRAPHY FOR MICROARCHITECTURAL CHARACTERISATION Article Open access 06 December 2023 PRELIMINARY STUDY ON THE EFFECT OF LUMBAR AXIAL ROTATION

ON BONE MINERAL DENSITY MEASURED BY DXA AND QCT Article Open access 03 September 2024 INTRODUCTION Incidence of fragility fractures, particularly in the hip and spine, is one of the most

common skeletal pathologies, due to the increasing rate of growth of elderly population. It is an important public health problem that is predominantly attributed to the occurrence of

osteoporosis. Osteoporosis is an age-related skeletal condition resulting in micro-architectural deterioration and loss of bone mass and density1. The pathological changes induced by

osteoporosis remain often unnoticed until a fracture occurs. While osteoporosis can be a primary bone loss condition due to aging, recent studies have elucidated other underlying conditions

such as multiple myeloma, diabetes, and cancer-treatments may elicit secondary osteoporosis2,3,4. Trauma, due to greater susceptibility to falls, is also a contributing cause to fractures in

the elderly. Fractures lead to poor quality of life, immobility, dependency, morbidity, and significant healthcare costs5. Apart from being a socioeconomic burden, it also significantly

impacts daily living and many patients do not return to their previous functional status6. In order to prevent the many sequelae of bone fragility, it is important to diagnose and treat the

condition early to avoid significant health risk. Dual energy X-ray absorptiometry (DXA) based bone mineral density (BMD) measurements in hip and spine (T- and Z- scores) have been used as

the gold standard for the quantitative evaluation of bone loss. However, the lack of specificity and sensitivity renders BMD a less than reliable predictor of bone loss1. BMD has been

demonstrated as an insufficient variable for bone strength prediction7 because there is a substantial overlap of BMD values of individuals with and without fractures8. Since the clinical

outcome of bone loss is bone fracture, it is increasingly being recognized that the identification of patients at high risk of fracture is more important than the identification of people

with bone loss9,10. Accurate assessment of fracture risk should ideally take into account other proven risk factors that add information to that provided by BMD alone11. The complex

structure of bone tissue highlights the concept that its mechanical strength is derived from the interaction of different structural elements and needs to be accounted for while assessing

fracture risk. According to several studies, BMD is only able to predict fractures with a detection rate of 30–50%12,13. This implies that BMD values often underestimate fracture risk, since

osteoporotic fractures frequently occur in patients with non-pathological BMD values14. Thus, it is necessary to move beyond a singular dependence on BMD since it excludes underlying

information on the local bone mass distribution. Finite-element (FE) modeling has increasingly been used to perform structural analysis of bones to assess strength and understand the

mechanism underlying bone fracture15,16. FE analysis acts as a non-invasive alternative to assess bone strength _in-vivo_, where radiological scan data of patients are translated into

patient-specific three-dimensional (3D) anatomical models. These geometric models are provided with appropriate material properties and boundary value and loading conditions that aims to

approximate the _in-vivo_ fracture conditions as possible, to obtain realistic predictions of the structural strength and other related mechanical properties. Several studies have

demonstrated the superiority of FE analysis based on computed tomography (CT) images to predict vertebral body compressive strength17,18,19. Also, patient-specific analysis and qualitative

visualization of the vertebrae are other unique advantages of FE analysis. Results derived from FE analysis can provide clinicians with valuable information about bone quality for diagnosis,

and targeted treatment planning and monitoring. Although the use of CT for diagnostic imaging has increased dramatically over the years, the fear of high radiation exposure has prompted

cautious use20. There is a general consensus that radiation dose that does not add value to patient care should be avoided. As such, there is little willingness to expose a patient to

ionizing radiation to monitor skeletal conditions such as osteoporosis until it is too late when the patient has already sustained fractures. However, low-dose CT (LDCT) could be a feasible

option for monitoring and evaluating bone health in osteoporotic patients and patients with predisposed skeletal conditions. Hence the need for clinical diagnostic evaluation for skeletal

conditions emphasizes the importance of optimizing radiation dose of each individual CT examination and reducing radiation exposure due to CT21. Recently, a study examined the effect of

radiation dose on image quality and mechanical strength of a single femur bone22. Another study by Musekyo _et al_. investigated the dose effect of CT-based volumetric BMD measurements23. In

this study, we analyzed the dose-dependency of FE analysis, which has been shown to improve fracture risk prediction independent of vertebral volumetric BMD24 and examined the feasibility

of using LDCT scans, instead of standard clinical imaging, to quantify bone loss in the vertebrae. MATERIALS AND METHODS STUDY SPECIMENS Elven fresh thoracic mid-vertebral specimens from

five human donors (two females aged 53 and 74 years and three males aged 46, 51, and 62 years, respectively), who have had no history of any skeletal diseases, were obtained from the

Institute of Pathology and Anatomy, Munich. The mid-vertebral specimens as the middle third of each vertebra were cut out parallel to the endplates by using a band saw (FK 22, Bizerba,

Balingen, Germany). The donors had dedicated their bodies for educational and research purposes to the local Institute prior to death, in compliance with local institutional and legislative

requirements. The study was reviewed and approved by the local institutional review board (Ethikkommission der Fakultaet fuer Medizin der Technischen Universitaet Muenchen, Munich, Germany).

MDCT IMAGING MDCT imaging was performed with a 64-row MDCT scanner (SOMATOM Definition AS, Siemens Healthcare, Erlangen, Germany). The mid-vertebral specimens were scanned in a water bath

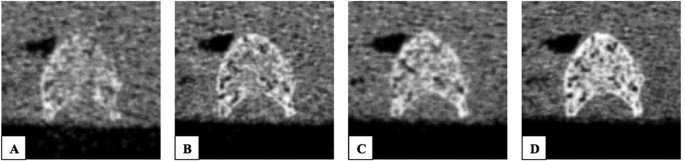
to simulate the soft tissue environment. Four scans with different tube exposures (80, 150, 220, and 500 mAs) were performed (Fig. 1). All other scan parameters were kept constant: tube

voltage of 120 kVp, image matrix of 512 × 512 pixels, pixel spacing of 0.44 × 0.44 mm2, and slice thickness of 0.4 mm. Transverse sections were reconstructed with a sharp reconstruction

kernel (U75u). Mean dose estimate in terms of CTDIvol was 17.5, 32.9, 48.2 and 109.5 mGy for tube exposures 80, 150, 220 and 500 mAs respectively. For calibration purposes, a reference

phantom with a bone-like and a water-like phase (Osteo Phantom, Siemens Healthcare) was placed in the scanner mat beneath patients (Fig. 2). FINITE ELEMENT MODELLING AND ANALYSIS MDCT

dataset of each specimen was imported and bone regions were segmented with commercial software Mimics (Materialise NV, Harislee, Belgium). The segmentation was performed semi-automatically,

by applying HU values threshold as well as manual delineation of choosing the regions and contours (Fig. 3). Linear tetrahedral FE meshes were generated using 3-Matic (Materialise NV,

Harislee, Belgium). Model convergence was investigated by differing the maximum edge lengths of mesh elements. The convergence study showed that a maximum edge length of 5 mm produced

converging FE solutions and was thus used in the construction of the rest of the FE models. Material properties were mapped onto meshed models using Hounsfield unit (HU)-density and

density-elastic modulus (E) relations _ρ__app_ = _47_ + _1.122*HU_ and _E_ = _−349_ + _5.82*ρ__app_ respectively, where E is expressed in MPa and the apparent density (ρapp) is expressed in

g/cm325. Transverse isotropy was assumed and the remaining engineering constants, including the Poisson’s ratio (v) and shear modulus (G) were assigned using the following relations: _E__xx_

 = _E__yy_ = _0.333E__zz__, v__xy_ = _0.381, v__xz_ = _v__yz_ = _0.104, G__xy_ = _0.121E__zz_ and _G__xz_ = _G__yz_ = _0.157E__zz_. The ash density (ρash) was then derived from the ρapp

(_ρ__ash_ = _ρ__app__*0.6_)26. Material strength (S) was defined for each material set based on its mean ρash using correlations, as described previously27,28: _S_ = _137ρ__ash__1.88_ for

ρash < 0.317 g/cm3 and _S_ = _114ρ__ash__1.72_ for ρash > 0.317 g/cm3. Material yield and ultimate failure were assumed to coincide and post-failure material behaviour was defined

based on previously established relations, which have been shown to accurately predict strains27,29,30,31. Any negative modulus obtained was set to 0.0001 MPa18. Boundary conditions were

applied using ABAQUS version 6.10 (Hibbitt, Karlsson, and Sorensen, Inc., Pawtucket, RI, USA) to simulate axial compression, where the inferior surface was constrained in all directions and

a displacement load condition was applied in the normal direction on the superior surface of each specimen. Fracture load was defined as the peak of the force-displacement curve, which was

used as an estimate of bone strength in this study. The above-described FE method has been detailed and experimentally validated in our previous work32. MDCT-GEOMETRY ANALYSIS The segmented

bone regions of each specimen for each individual dose were converted to surface models and then compared in terms of Hausdorff distance, which is a measure of the distance between a point

of one of two sets to a point of the other set33. For each surface model, in addition to the Hausdorff distance, the percentage of points that fall within 1 mm of the calculated distance

were also estimated22, with the high CT dose (500 mAs) scans as the reference. MDCT-BASED BMD ANALYSIS Elliptical regions of interest (ROIs) were placed on an axial mid-slice for each

specimen. These ROIS automatically provide the mean density in terms of HUs and its standard deviation within the region. Reference ROIs were placed within bone density calibration phantoms

composed of hydroxyapatite (HA), where the water-like part of phantom has HA density of 0 mg/cm3 (HAw) and the bone-like part of the phantom has HA density of 200 mg/cm3 (HAb). BMD was

calculated by the following formula: BMD = [HAb/ (HUb – HUw)]*(HU-HUw)34, by assuming linear interpolation between the HUs of the water-like regions (HUw) and bone-like regions (HUb).

STATISTICAL ANALYSIS A paired two-tailed t-test was used to compare the differences in image quality, geometry, and FE-predicted fracture loads between CT doses (80, 150 and 220 mAs).

Regression equations and the coefficients of determination (R2) were used to evaluate the linear correlations between fracture loads obtained for individual doses as well as between

MDCT-determined BMD values and fracture loads. High dose CT (500 mAs) scans were taken as the reference for comparisons between individual doses. The reproducibility of the fracture loads

for each individual dose (80, 150 and 220 mAs), with reference to the fracture loads obtained at 500 mAs, were assessed using the Bland and Altman plots35 and Lin’s concordance correlation

coefficient (rc)36. All analyses were performed using spreadsheet application (Microsoft Office Excel 2010, Redmond, WA), except for Lin’s coefficient, which was calculated using MedCalc

version 16.8.4 (MedCalc Software, Mariakerke, Belgium). A value of p < 0.05 was considered as statistically significant. RESULTS IMAGE QUALITY The differences between each CT dose in

terms of the density and noise were analyzed. The mean density, mean noise and their respective standard deviations were tabulated (Table 1). There were no significant differences in mean

density between CT doses (Fig. 4A). However, image noise was significantly lower in 500 mAs, compared to 80 mAs (25.3%; p < 0.001) (Fig. 4B). There were no significant differences

observed in mean noise with 150 mAs and 220 mAs, as compared to 500 mAs. FE-PREDICTED FRACTURE LOAD Significant correlations were obtained for FE-predicted fracture load values for 80 mAs

(R2 = 0.997, p < 0.001), 150 mAs (R2 = 0.998, p < 0.001) and 220 mAs (R2 = 0.987, p < 0.001), against 500 mAs as the reference (Fig. 5A-C). There were no significant differences in

fracture loads obtained for each individual dose against 500 mAs. Good concordance between each individual dose and 500 mAs was also found for 80 mAs (rc = 0.998), 150 mAs (rc = 0.999) and

220 mAs (rc = 0.993) (Fig. 5D-F). However, there were no significant correlations in the Bland–Altman plots for either dose (80, 150 and 220 mAs), indicating there was a consistent bias.

Also, significant correlations were observed between MDCT-determined BMD and fracture load values for 80 mAs (R2 = 0.879, p < 0.001), 150 mAs (R2 = 0.867, p < 0.001), 220 mAs (R2 = 

0.904, p < 0.001), and 500 mAs (R2 = 0.885, p < 0.001) (Fig. 6). FE-predicted strength estimates for each mid-vertebra specimen across different doses (80, 150, 220 and 500 mAs) are

listed in Supplementary Table 1. GEOMETRY Comparing the surface models of 80, 150, and 220 mAs against 500 mAs, the maximum Hausdorff distance obtained was approximately the same (Table 2)

for all doses and no significant differences were observed. Similarly, the percentage of points that fell within a 1 mm distance was about 90%. In addition, the mean error in volume

decreased with CT dose, where mean error in volume was approximately 7%, 4%, and 2% for the 80 mAs, 150 mAs, and 220 mAs respectively. There were no significant differences between

individual doses for the volumes of models generated for FE analysis. DISCUSSION In this study, FE-predicted fracture load values obtained from MDCT data were not affected by tube exposure

reduction from 500 to 80 mAs, with no significant differences in terms of correlation, with the high CT dose (500 mAs) scans used as reference. Although the noise significantly reduced from

LDCT (80 mAs) to high-dose CT (500 mAs) specimens, there were no differences in outcome, in terms of fracture load values obtained from FE analysis, since the density values remained

unaffected. Accordingly, there were no significant differences observed in volume segmentations between the doses examined in this study. The reliability of the fracture load values were

further emphasized with significant correlations obtained with MDCT-determined BMD values, since several studies have also established a linear correlation37. These findings are consistent

with the only one other published study that the authors are aware of, which compared CT-based FE analysis of a single femur bone with varying radiation doses from 180 mAs to 80 mAs22. In

developed countries, the use of CT is known to account for almost 17% of all radiological examinations, but is attributed to 47% or higher of medical radiation dose38. With such an alarming

contribution, it is necessary to evaluate the efficacy of routine CT imaging. In patients with predisposed skeletal conditions, routine CT imaging to evaluate bone strength is always

performed with caution. In addition, due to the high ionizing radiation exposure of routine imaging, follow-up scans are limited until or unless necessary. As such, the evaluation of bone

strength may valuable in early diagnosis of skeletal conditions such as osteoporosis. Not only does LDCT FE analysis provide the possibility of scanning in patients with predisposed

conditions, but also allows more frequent follow-up scans, which implies that bone strength can be predicted and treated early as well as monitored regularly. Furthermore, risk for bone

fracture can be more accurately diagnosed with the complementation of FE-predicted bone strength information, in addition to BMD. The aim of this study was to examine whether LDCT

examinations (80 mAs and 150 mAs) were as accurate, in comparison to standard routine CT scans (220 mAs) and high dose CT scans (500 mAs) in providing structural information using FE

analysis. The main implication of our findings is that dose reduction, with respects to tube exposure, does not affect structural findings, such as strength estimations, obtained from

engineering analysis. This is critical because a dose reduction from routine clinical imaging dose (220 mAs) to LDCT dose (80 mAs) is a significant reduction by 64% but the highest

percentage error in volume segmentations did not exceed 10%, which certainly justifies its consideration as a clinical tool in the biomechanical quantification of bone strength. Based on

concerns of inferior image quality and diagnostic accuracy, LDCT has still not been clinically appreciated for assessment of patients with bone loss. These findings support that LDCT may be

utilized as the preliminary imaging examination, to accurately evaluate bone strength and the consequent fracture risk of patients. Several studies have demonstrated the usefulness of LDCT

for clinical diagnosis in patients with other pathological skeletal conditions. For instance, Horger _et al_. found low-dose MDCT scans (tube exposure: 40–60 mAs) were capable of diagnosing

lytic bone changes and evaluating fracture risk, even in patients with known or expected reduced bone density, in patients with multiple myeloma39. Bohy _et al_. showed that a 65% reduction

in CT dose does not impact diagnostic findings evaluated in patients with lumbar disk herniation40. Similarly, Mulkens _et al_. demonstrated that a 61–71% dose reduction can be obtained with

negligible reduction in image quality41 in the assessment of cervical spine clearance in patients with blunt force trauma. In line with these findings, our results show that a 64% dose

reduction in CT dose did not affect image quality as well as bone strength predictions. Hence, the first step may be to opt for LDCT scanning protocol, accompanied with structural

engineering analysis, which bears several benefits in terms of assessing bone quality and predicting fracture risk. A key benefit of a combined LDCT and structural engineering analysis

protocol is that the outcome of improved diagnosis of osteoporosis. As aforementioned, BMD alone is not sufficient enough to describe the intricacies involving bone architecture, geometric,

and structural properties. The unique feature of FE analysis is the integration of structure, geometry and density distribution to evaluate bone strength42. As such, bone strength assessment

via FE-analysis using LDCT scans can be a complementing measure to patients suffering from bone loss. Since BMD is insufficient to accurately predict bone strength, LDCT leads the

possibility of diagnosing bone loss with more predictive components of fracture risk. Furthermore, with LDCT scanning, there is a true possibility of increasing frequency of follow up scans.

This means that apart from diagnosing bone loss, monitoring bone loss and effect of treatment at shorter intervals will be plausible. Another key benefit of LDCT is the increased

feasibility of radiological examinations in patients predisposed to other pathological skeletal conditions. It has been shown that patients undergoing cancer treatments, in the form of

radiation therapy, experience insufficiency fractures43. As such, subjecting them to additional CT-based radiation for diagnostic evaluation of bone loss may not be practical, because of a

probable risk of radiation-induced malignancy44 or fear of aggravated bone loss, leading to secondary bone fractures. Given these potential risks, it can be easily argued that radiation

exposure associated with routine CT imaging for the evaluation of bone loss is inapplicable. There are several limitations inherent to this study. The main limitation is the lack of

verification on the use of LDCT in evaluating micro-architectural variables, i.e. trabecular-level changes, which may produce different results. However, the accuracy and reliability of

FE-predicted bone strength have been demonstrated in several studies16,29,45. Also, this study only considered CT dose reduction via the variation of tube current but dose can also be

reduced by reducing tube voltage or increasing pitch38 for instance. Nevertheless, variation of tube current is the most prevalent method for CT dose reduction22. In addition, a snap sample

number of n = 11 were used in this study. The use of a larger sample size will provide statistical weighting to the findings obtained. However, despite these limitations, the merit of these

findings is that LDCT, in combination with FE analysis, may be more valuable than we realize as a clinical diagnostic tool. In conclusion, our results demonstrated that CT dose reduction by

up to 64% does not affect strength estimates obtained from FE analysis. One of the clinical questions that should be answered is: is routine imaging required? If there is a clear indication

for imaging, the option of LDCT scans should first be considered. Clinical diagnostic evaluation, in terms of radiological examinations, has profound impact on the overall radiation dose

delivered to each individual. Apart from diagnosis of bone loss, efforts should focus onto monitoring bone loss to predict fractures early and therefore, CT scanning protocol should be

optimized for the intended clinical evaluation. ADDITIONAL INFORMATION HOW TO CITE THIS ARTICLE: Anitha, D. _et al_. Effects of dose reduction on bone strength prediction using finite

element analysis. _Sci. Rep._ 6, 38441; doi: 10.1038/srep38441 (2016). PUBLISHER'S NOTE: Springer Nature remains neutral with regard to jurisdictional claims in published maps and

institutional affiliations. REFERENCES * Kanis, J. A. Diagnosis of osteoporosis and assessment of fracture risk. Lancet 359, 1929–1936, doi: 10.1016/S0140-6736(02)08761-5 (2002). Article 

PubMed  Google Scholar  * Faiman, B. & Licata, A. A. New tools for detecting occult monoclonal gammopathy, a cause of secondary osteoporosis. Cleve Clin J Med 77, 273–278, doi:

10.3949/ccjm.77a.09091 (2010). Article  PubMed  Google Scholar  * Nicodemus, K. K. & Folsom, A. R. & Iowa Women’s Health, S. Type 1 and type 2 diabetes and incident hip fractures in

postmenopausal women. Diabetes Care 24, 1192–1197 (2001). Article  CAS  Google Scholar  * Pfeilschifter, J. & Diel, I. J. Osteoporosis due to cancer treatment: Pathogenesis and

management. J Clin Oncol 18, 1570–1593 (2000). Article  CAS  Google Scholar  * Teng, G. G., Curtis, J. R. & Saag, K. G. Mortality and osteoporotic fractures: is the link causal, and is

it modifiable? Clin Exp Rheumatol 26, S125–S137 (2008). CAS  PubMed  PubMed Central  Google Scholar  * Magaziner, J. et al. Changes in functional status attributable to hip fracture: a

comparison of hip fracture patients to community-dwelling aged. Am J Epidemiol 157, 1023–1031 (2003). Article  Google Scholar  * Ulrich, D., van Rietbergen, B., Laib, A. & Ruegsegger, P.

The ability of three-dimensional structural indices to reflect mechanical aspects of trabecular bone. Bone 25, 55–60 (1999). Article  CAS  Google Scholar  * Melton, L. J., 3rd et al.

Epidemiology of vertebral fractures in women. Am J Epidemiol 129, 1000–1011 (1989). Article  Google Scholar  * Arabi, A. et al. Discriminative ability of dual-energy X-ray absorptiometry

site selection in identifying patients with osteoporotic fractures. Bone 40, 1060–1065, doi: 10.1016/j.bone.2006.11.017 (2007). Article  PubMed  Google Scholar  * Marshall, D., Johnell, O.

& Wedel, H. Meta-analysis of how well measures of bone mineral density predict occurrence of osteoporotic fractures. BMJ 312, 1254–1259 (1996). Article  CAS  Google Scholar  * Fonseca,

H., Moreira-Goncalves, D., Coriolano, H. J. & Duarte, J. A. Bone quality: the determinants of bone strength and fragility. Sports Med 44, 37–53, doi: 10.1007/s40279-013-0100-7 (2014).

Article  PubMed  Google Scholar  * McCreadi, B. R. & Goldsteim, S. A. Biomechanics of fracture: is bone mineral density sufficient to assess risk? J Bone Miner Res 15, 2305–2308 (2000).

Article  Google Scholar  * Schott, A. M. et al. How hip and whole-body bone mineral density predict hip fracture in elderly women: The EPIDOS prospective study. Osteoporos Int 8, 247–254,

doi: 10.1007/s001980050061 (1998). Article  CAS  PubMed  Google Scholar  * Baum, T. et al. Osteoporosis imaging: effects of bone preservation on MDCT-based trabecular bone microstructure

parameters and finite element models. BMC Med Imaging 15, 22, doi: 10.1186/s12880-015-0066-z (2015). Article  PubMed  PubMed Central  Google Scholar  * Kopperdahl, D. et al. Assessment of

incident spine and hip fractures in women and men using finite element analysis of CT scans J Bone Miner Res 29, 570–580 (2014). Article  Google Scholar  * Anitha, D., Kim, K. J., Lim, S. K.

& Lee, T. Implications of local osteoporosis on the efficacy of anti-resorptive drug treatment: a 3-year follow-up finite element study in risedronate-treated women. Osteoporos Int 24,

3043–3051, doi: 10.1007/s00198-013-2424-4 (2013). Article  CAS  PubMed  Google Scholar  * Black, D. M. et al. An assessment tool for predicting fracture risk in postmenopausal women.

Osteoporos Int 12, 519–528, doi: 10.1007/s001980170072 (2001). Article  CAS  PubMed  Google Scholar  * Crawford, R. P., Cann, C. E. & Keaveny, T. M. Finite element models predict _in

vitro_ vertebral body compressive strength better than quantitative computed tomography. Bone 33, 744–750 (2003). Article  Google Scholar  * Cody, D. D. et al. Femoral strength is better

predicted by finite element models than QCT and DXA. J Biomech 32, 1013–1020 (1999). Article  CAS  Google Scholar  * Harvey, H. B., Brink, J. A. & Frush, D. P. Informed Consent for

Radiation Risk from CT Is Unjustified Based on the Current Scientific Evidence. Radiology 275, 321–325 (2015). Article  Google Scholar  * Higashigaito, K. et al. Automatic radiation dose

monitoring for CT of trauma patients with different protocols: feasibility and accuracy. Clin Radiol, doi: 10.1016/j.crad.2016.04.023 (2016). * Falcinelli, C., Schileo, E., Baruffaldi, F.,

Cristofolini, L. & Taddei, F. The effect of computed tomography current reduction on proximal femur subject-specific finite-element models. J Mech Med Bio 17 (2016). * Museyko, O. et al.

A low-radiation exposure protocol for 3D QCT of the spine. Osteoporos Int 25, 983–992, doi: 10.1007/s00198-013-2544-x (2014). Article  CAS  PubMed  Google Scholar  * Kopperdahl, D. L. et

al. Assessment of incident spine and hip fractures in women and men using finite element analysis of CT scans. J Bone Miner Res 29, 570–580 (2014). Article  Google Scholar  * Rho, J. Y.,

Hobatho, M. C. & Ashman, R. B. Relations of mechanical properties to density and CT numbers in human bone. Med Eng Phys 17, 347–355 (1995). Article  CAS  Google Scholar  * Goulet, R. W.

et al. The relationship between the structural and orthogonal compressive properties of trabecular bone. Journal of Biomechanics 27, 375–389 (1994). Article  CAS  Google Scholar  * Keller,

T. S. Predicting the compressive mechanical behavior of bone. J Biomech 27, 1159–1168 (1994). Article  CAS  Google Scholar  * Keyak, J. H., Lee, I. Y. & Skinner, H. B. Correlations

between orthogonal mechanical properties and density of trabecular bone: use of different densitometric measures. J Biomed Mater Res 28, 1329–1336, doi: 10.1002/jbm.820281111 (1994). Article

  CAS  PubMed  Google Scholar  * Keyak, J. H. Improved prediction of proximal femoral fracture load using nonlinear finite element models. Med Eng Phys 23, 165–173 (2001). Article  CAS 

Google Scholar  * Keyak, J. H. & Falkinstein, Y. Comparison of _in situ_ and _in vitro_ CT scan-based finite element model predictions of proximal femoral fracture load. Med Eng Phys 25,

781–787 (2003). Article  Google Scholar  * Crawford, R. P., Rosenberg, W. S. & Keaveny, T. M. Quantitative computed tomography-based finite element models of the human lumbar vertebral

body: effect of element size on stiffness, damage, and fracture strength predictions. J Biomech Eng 125, 434–438 (2003). Article  Google Scholar  * Anitha, D., Subburaj, K., Baum, T. &

Kirschke, J. S. in _European Orthopaedic Research Society 24th Annual Meeting_ (Bologna, Italy, 2016). * Spyridonos, P., Gaitanis, G., Bassukas, I. D. & Tzaphlidou, M. Gray Hausdorff

distance measure for medical image comparison in dermatology: Evaluation of treatment effectiveness by image similarity. Skin Res Technol 19, e498–506, doi: 10.1111/srt.12001 (2013). Article

  PubMed  Google Scholar  * Huber, M. B. et al. Proximal Femur Specimens: Automated 3D Trabecular Bone Mineral Density Analysis at Multidetector CT - Correlation with Biomechanical Strength

Measurement. Radiology 247, 472–481 (2008). Article  Google Scholar  * Bland, J. M. & Altman, D. G. Measuring agreement in method comparison studies. Statistical Methods in Medical

Research 8, 135–160 (1999). Article  CAS  Google Scholar  * Lin, L. I. A concordance correlation coefficient to evaluate reproducibility. Biometrics 45, 255–268 (1989). Article  CAS  Google

Scholar  * Melton, L. J., 3rd et al. Structural determinants of vertebral fracture risk. J Bone Miner Res 22, 1885–1892, doi: 10.1359/jbmr.070728 (2007). Article  PubMed  Google Scholar  *

Nassiri, M. A., Rouleau, M. & Despres, P. CT dose reduction: approaches, strategies and results from a province-wide program in Quebec. J Radiol Prot 36, 346–362, doi:

10.1088/0952-4746/36/2/346 (2016). Article  PubMed  Google Scholar  * Horger, M. et al. Whole-body low-dose multidetector row-CT in the diagnosis of multiple myeloma: an alternative to

conventional radiography. Eur J Radiol 54, 289–297, doi: 10.1016/j.ejrad.2004.04.015 (2005). Article  PubMed  Google Scholar  * Bohy, P. et al. Multidetector CT in Patients Suspected of

Having Lumbar Disk Herniation: Comparison of Standard-Dose and Simulated Low-Dose Techniques Radiology 244, 524–531 (2007). Article  Google Scholar  * Mulkens, T. H. et al. Comparison of

low-dose with standard-dose multidetector CT in cervical spine trauma. AJNR Am J Neuroradiol 28, 1444–1450, doi: 10.3174/ajnr.A0608 (2007). Article  CAS  PubMed  Google Scholar  * Engelke,

K., Libanati, C., Fuerst, T., Zysset, P. & Genant, H. K. Advanced CT based _in vivo_ methods for the assessment of bone density, structure, and strength. Curr Osteoporos Rep 11, 246–255,

doi: 10.1007/s11914-013-0147-2 (2013). Article  CAS  PubMed  Google Scholar  * Pacheco, R. & Stock, H. Effects of Radiation on Bone. Curr Osteoporos Rep 11, 299–304 (2013). Article 

Google Scholar  * Bluemke, D. A., Fishman, E. K. & Scott, W. W., Jr. Skeletal complications of radiation therapy. Radiographics 14, 111–121, doi: 10.1148/radiographics.14.1.8128043

(1994). Article  CAS  PubMed  Google Scholar  * Imai, K. Computed tomography-based finite element analysis to assess fracture risk and osteoporosis treatment. World J Exp Med 5, 182–187,

doi: 10.5493/wjem.v5.i3.182 (2015). Article  PubMed  PubMed Central  Google Scholar  Download references ACKNOWLEDGEMENTS This work was supported by the following research grants: Deutsche

Forschungsgemeinschaft (DFG) BA 4085/2–1 (JSK) and BA 4906/1–1 (TB); European Research Council (ERC) Starting Grant Stg-2014 637164 iBack (JSK). Singapore University of Technology and Design

(SUTD) Start-up Research Grant SRG EPD 2015 093 (KS). The funding agencies had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.

AUTHOR INFORMATION AUTHORS AND AFFILIATIONS * Engineering Product Development (EPD) Pillar, Singapore University of Technology and Design (SUTD), 8 Somapah Road, 487372, Singapore D. Anitha 

& Karupppasamy Subburaj * Department of Radiology, Klinikum rechts der Isar, Technical University of Munich, Munich, Germany Kai Mei, Felix K. Kopp, Peter B. Noel & Thomas Baum *

Department of Orthopaedics and Sports Orthopaedics, Biomechanical Laboratory, Klinikum rechts der Isar, Technical University of Munich, Munich, Germany Peter Foehr * Department of

Neuroradiology, Klinikum rechts der Isar, Technical University of Munich, Muenchen, Germany Jan S. Kirschke Authors * D. Anitha View author publications You can also search for this author

inPubMed Google Scholar * Karupppasamy Subburaj View author publications You can also search for this author inPubMed Google Scholar * Kai Mei View author publications You can also search

for this author inPubMed Google Scholar * Felix K. Kopp View author publications You can also search for this author inPubMed Google Scholar * Peter Foehr View author publications You can

also search for this author inPubMed Google Scholar * Peter B. Noel View author publications You can also search for this author inPubMed Google Scholar * Jan S. Kirschke View author

publications You can also search for this author inPubMed Google Scholar * Thomas Baum View author publications You can also search for this author inPubMed Google Scholar CONTRIBUTIONS

D.A., K.S., P.B.N., J.S.K. and T.B. designed the study. K.M., F.K.K., P.F., J.S.K. and T.B. coordinated and conducted specimen collection and preparation and image acquisition. D.A., K.S.,

J.S.K. and T.B. wrote the analysis plan. K.S. and T.B. provided data, analysis tools, and coordinated data analysis. D.A. performed FE modeling and data analysis. D.A., K.S., K.M., F.K.K.,

P.F., J.S.K. and T.B. drafted the manuscript. All authors reviewed the manuscript before submission. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing financial

interests. ELECTRONIC SUPPLEMENTARY MATERIAL SUPPLEMENTARY TABLE RIGHTS AND PERMISSIONS This work is licensed under a Creative Commons Attribution 4.0 International License. The images or

other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the

Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit

http://creativecommons.org/licenses/by/4.0/ Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Anitha, D., Subburaj, K., Mei, K. _et al._ Effects of dose reduction on bone

strength prediction using finite element analysis. _Sci Rep_ 6, 38441 (2016). https://doi.org/10.1038/srep38441 Download citation * Received: 12 August 2016 * Accepted: 08 November 2016 *

Published: 09 December 2016 * DOI: https://doi.org/10.1038/srep38441 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry,

a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative