ABSTRACT Mating systems will influence gene spread across the natural distribution of a plant species. Existing theories have not fully explored the role of mating systems on the wave of

advance of an advantageous gene. Here, we develop a theory to account for the rate of spread of both advantageous and neutral genes under different mating systems, based on

migration-selection processes. We show that a complex relationship exists between selfing rate and the speed of gene spread. The interaction of selfing with gametophytic selection shapes the

traveling wave of the advantageous gene. Selfing can impede (or enhance) the spread of an advantageous gene in the presence (or absence) of gametophytic selection. The interaction of

genes. The stochastic process does not alter the pattern of selfing effects except for increasing the uncertainty of the waves of advance of both advantageous and neutral genes. This theory

helps us to explain how mating systems act as a barrier to spread of adaptive and neutral genes, and to interpret species cohesion maintained by a low level of adaptive gene flow. You have

full access to this article via your institution. Download PDF SIMILAR CONTENT BEING VIEWED BY OTHERS LONG-RANGE LINKAGE EFFECTS IN ADAPTING SEXUAL POPULATIONS Article Open access 01 August

2023 TRANSPOSABLE ELEMENTS MAINTAIN GENOME-WIDE HETEROZYGOSITY IN INBRED POPULATIONS Article Open access 17 November 2022 POLYGENIC ADAPTATION: A UNIFYING FRAMEWORK TO UNDERSTAND POSITIVE

SELECTION Article 29 June 2020 INTRODUCTION Gene spread in space is affected by many factors, including the mode of gene inheritance (uni- vs. bi-parental inheritance), physical barriers,

gene neutrality, irregular dispersal of pollen and seeds for plants (or gametes and zygotes for animals), variation in population density, and variation of selection (intrinsic vs.

extrinsic) in different habitats. Fisher (1937) first studied the wave of advance of advantageous nuclear genes in one-dimensional homogeneous space, showing that the velocity is

proportional to the square root of the product of selection advantage and diffusion coefficient. When applied to biological invasion, this conclusion generally holds in theory under the

selection-migration processes but is modified in various situations (Shigesada and Kawasaki 1997), such as biological invasion within heterogeneous habitats or in the presence of competing

species. In flowering plants, both gene spread and biological invasion (or species range expansion) are potentially affected by mating systems (Grossenbacher et al. 2015; Razanajatovo et al.

2016; Pickup et al. 2019). For instance, pollen limitation can lead to Allee effects and slow down species invasion (Groom 1998; Davis et al. 2004). Mating systems may act as a barrier to

gene flow or interact with selection, which naturally influences the wave of advance of advantageous genes, or the species range expansion that could co-evolve with the wave of advance of

advantageous genes (Hu et al. 2019). Nevertheless, the significance of mating systems in regulating the wave of advance of advantageous genes remains to be elaborated. The interaction of

mating systems with selection in shaping gene spread can occur in both the gametophyte and sporophyte stages of the life cycle. We recently showed that selfing as a barrier to adaptive gene

flow can limit a species range under gametophytic selection (Hu et al. 2019). Differences between outcrossing rates could provide a biological basis for pre-mating barriers to both neutral

and selective gene flow between genetically related species (Pickup et al. 2019). Genes may be expressed in either the gametophyte or sporophyte stage, or in both stages (Tanksley et al.

1981; Shaw et al. 2011), and are likely involved in gametophytic and sporophytic selection. Peters and Weis (2018) show the influences of selfing on the spread and maintenance of

pollen-expressed genes that affect pollen competitiveness in the model of a single locus controlling pleiotropic antagonism between pollen competitiveness and diploid fitness. Selfing

interacts with selection in different ways in the gametophyte and sporophyte stages of a flowering plant species (Charlesworth and Charlesworth 1992; Damgaard et al. 1994; Hu and Li 2003).

For instance, when both gametic and zygotic selection are present, the interaction between selfing and zygotic selection increases allele frequency, but the interaction between selfing and

gametic selection reduces allele frequency (Hu 2015, p 1177). Also, mating systems interact with alternation of generations in plant life cycle. Early theories show that selfing enhances the

predominance of the gametophytic generation of the life cycle, while outcrossing favors the sporophyte generation of the life cycle (Otto and Marks 1996). This potentially influences the

wave of advance of advantageous genes among species with different rates of selfing. Thus, it is necessary to bring together the joint effects of selfing with gametophytic and/or sporophytic

selection to examine their influence on the rate of gene spread. The interaction of mating systems with migration is realized through changing pollination, an essential phase in the life

cycle of flowering plants. There are diverse systems of mating ranging from outcrossing to selfing in plants, with many species exhibiting a mixed mating system (Igic and Kohn 2006). At one

extreme, complete self-fertilization assures reproductive success and maintains population growth (Darwin 1876), even in the absence of pollinators. Here the wave of advance of advantageous

genes is carried out by seed flow only. At another extreme, outcrossing or self-incompatible species maximize pollen transfer from other individuals while minimizing inbreeding depression

(Charlesworth and Willis 2009). Here the wave of advance of advantageous genes can be carried out by pollen or seed flow alone, or both. The relative contribution to gene spread is often

associated with the presence or absence of physical barriers and the type of vectors for seed and pollen dispersal. This varies with species, as implied from the estimates of the rates of

pollen to seed flow (_m__p_/_m__s_, Table 1) derived from molecular markers (Ennos 1994). An intermediate system is mixed mating where both selfing and outcrossing modes of transmission of

parental gene copies to progeny occur with certain probabilities. Although the evolutionary stability of this system remains under debate (Charlesworth 2006; Johnston et al. 2009; Barrett

2014), it does add more complexity to analyzing the role of mating systems in regulating gene spread (Hu 2011). Thus, it is important to consider the interaction of mating systems with

pollen flow in addressing the wave of gene advance. In addition, when a nuclear neutral gene is considered, a potential wave generated by seed and pollen flow dissipates with time in

homogeneous habitats, irrespective of presence or absence of a genetic drift process. When the neutral gene is tightly linked with a selective gene, the transient wave of advance of the

neutral gene is maintained by migration and linkage disequilibrium (LD) processes. This is analogous to the transient clines of neutral genes in the cytonuclear system (Hu 2008). LD is

generated by gene flow but eroded by recombination between the two loci. This differs from genetic hitchhiking or background selection effects where the effective population size for the

neutral gene is decreased owing to selection at its linked selective site (Nordborg et al. 1996a, b; Barton 2000; Hu and He 2005). A further effect of the selfing process is strengthening LD

(Nordborg et al. 1996a, b; Nordborg 2000), and the transient wave of neutral gene advance is anticipated to follow the wave of advance of advantageous genes. Selfing alters neutral gene

spread and could produce a wave different in shape from that under a random mating system or in a subdivided population (Slatkin and Charlesworth 1978; Slatkin and Wiehe 1998; Barton 2000).

Here, we bring together the processes of mating systems, selection and migration to examine the wave of advance of both advantageous and neutral genes in flowering plants. We begin by

describing the basic theory for modeling the dynamics of selective and neutral nuclear genes in one-dimensional homogeneous space, followed by both numerical and Monte Carlo (MC) simulations

to examine the effects of mating systems on gene spread. Inferences on the role of mating systems in shaping the wave of advance of advantageous and neutral genes are drawn from both the

analytical model and simulation examples. BASIC THEORY A SINGLE SELECTIVE GENE The theory deals with a hermaphrodite plant species distributed in one-dimensional homogeneous space. Consider

an initial population that is stable in demography and genetic composition. The influence on the initial population from reverse dispersal is assumed to be negligible. A mutant allele has an

advantage over preexisting alleles and has attained a high frequency due to natural selection in the starting population. Adaptive alleles spread from the initial population and move along

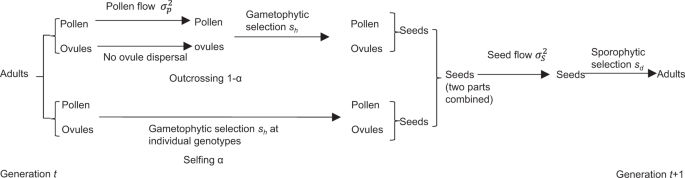
one-dimensional space. Figure 1 shows the life cycle for each local population that follows the sequence of events: generation of pollen and ovules, pollen flow, selection at the gametophyte

stage, mixed mating, seed flow, and selection at the sporophyte stage (Hu et al. 2019). The difference from a previous study (Hu et al. 2019) lies in that we focus here on the spread of

advantageous mutant alleles where traveling waves occur (Fisher 1937). A traveling wave is the term given to the processes in which an allele advances at a constant speed while maintaining a

constant shape of the distribution of allele frequencies (Shigesada and Kawasaki 1997, p 47). Weak selection is considered in both gametophyte and sporophyte stages so that the terms with

selection coefficients of the second or higher orders are neglected in mathematic deduction. For the rigorous theoretical analysis, the life cycle is assumed to occur within a time interval

Δ_t_ so that partial differentiation equations are derived when Δ_t_ becomes infinitely small (Nagylaki 1975). The diffusion process is applied to approximate seed and pollen flow in space.

Dispersal variances of pollen (\(\sigma _P^2\)) and seeds (\(\sigma _S^2\)) are assumed to be of the same order as the selection coefficients so that terms with the product of gene flow and

selection coefficients are neglected. Mutation rate is assumed to be small and its effects are excluded. Genetic drift effects are only considered in MC simulations. In the Supporting

Information (SI) Appendix the derivations of recursive gene and genotype frequencies at two loci are detailed. Here we briefly describe a selective nuclear locus, with alleles _A_ and _a_.

In the gametophyte stage, let _w__A_ = 1 + _s__h_ be the fitness for gamete _A_ in pollen and ovules, where _s__h_ is the selection coefficient, and _w__a_ = 1 for gamete _a_. Allele _A_ is

advantageous over allele _a_ in adaptation to the habitat. The average fitness in pollen and ovules, denoted by _w__p_ and _w__o_, respectively, is calculated using the conventional method

\(w_P = \mathop {\sum}\nolimits_{i = A,a} {w_ip_i^ \ast }\), where \({p_i^ \ast}\) is the allele frequency after pollen flow, and \(w_O = \mathop {\sum}\nolimits_{i = A,a} {w_ip_i}\) where

_p__i_ is the allele frequency in adults before pollen and ovule formation because ovules do not migrate between populations. Selection in pollen increases the frequency of the advantageous

allele, which removes maladaptive alleles from both resident and migrant pollen, resulting in pollen discounting. For simplicity but without neglecting the role of selfing in shaping gene

spread, we consider an additive selective model for genotype fitness in the sporophyte stage. Let _W__AA_ = 1 + 2_s__d_ be the fitness of genotype _AA_, where _s__d_ is the selection

coefficient; _W__Aa_ = 1 + _s__d_ for the fitness of heterozygotes _Aa_; and _W__aa_ = 1 for genotype _aa_. The average fitness in the sporophyte stage is \(\overline W = W_{AA}p_{AA}^ \ast

+ W_{Aa}p_{Aa}^ \ast + W_{aa}p_{aa}^ \ast\), where \(p_{ij}^ \ast\) is the frequency of genotype _ij_ (_i, j_ = _A, a_) after seed dispersal. Let _α_ be the selfing rate in each local

population, _α_ = 1 for complete self-fertilization and _α_ = 0 for outcrossing. Let _n_ be population density at position _x_ and time _t_. For simplicity of mathematical expression, we use

notation \(\dot f\) for ∂_f_/∂_t_, _f_′ for ∂_f/_∂_x_, and _f_′′ for ∂2_f_/∂_x_2 for a function _f_. With these settings, the dynamics for the frequency of the advantageous allele is

obtained from Eq. (A25) in SI Appendix: $$\begin{array}{l}\dot p_A = \widetilde \sigma ^2\left( {{\mathrm{ln}}\left( n \right)} \right)\prime p_A^\prime + \frac{{\widetilde \sigma

^2}}{2}p_A^{\prime\prime} \\+ s_h\left( {\left( {1 - \frac{1}{2}\alpha } \right)p_Ap_a - \frac{1}{2}\alpha D_A} \right) \\+ s_d\left( {\left( {1 + \frac{1}{2}\alpha } \right)p_Ap_a +

\frac{1}{2}\alpha D_A} \right)\end{array},$$ (1) where _D__A_ = (_P__Aa_ − 2_p__A__p__a_)/2 is the Hardy–Weinberg disequilibrium (HWD) coefficient and \(\widetilde \sigma ^2 = \left( {1 -

\alpha } \right)\sigma _P^2/2 + \sigma _S^2\) is a composite rate of seed and pollen flow. The first term on the right side of Eq. (1) represents the effects of asymmetric dispersal that

carries genes from dense populations to less dense populations when (ln(_n_))′ > 0, or vice versa _w_(ln(_n_))′ < 0. The second term represents the contribution from seeds or pollen

flow. The proportion \(\left( {1 - \alpha } \right)\sigma _P^2/2\) in \(\widetilde \sigma ^2\) is involved in pollination, and only _α_\(\sigma _P^2/2\) of cross pollen is not used, which is

one type of pollen discounting (reduced outcross pollen due to selfing). A high selfing rate causes a large proportion of pollen discounting (Kohn and Barrett 1994). The third and fourth

components are effects of selection, showing that selfing differently interacts with gametic and zygotic selection, respectively (Hu and Li 2003; Hu 2015). From Eq. (A27) in SI Appendix, the

dynamics of HWD coefficient _D__A_ with space _x_ and time _t_ is derived as follows: $$\begin{array}{l}\dot D_A = \left( { - 1 + \frac{1}{2}\alpha + \left( {s_d - \frac{1}{2}s_h}

\right)\alpha \left( {p_a - p_A} \right)p_ap_A} \right)D_A\\ + \left( {\frac{1}{2} + \left( {s_d + \frac{1}{2}s_h} \right)\left( {p_a - p_A} \right)} \right)\alpha p_Ap_a + \sigma _S^2\left(

{p_A^\prime } \right)^2 \\+ \frac{1}{2}\alpha \sigma _S^2\left( {\left( {{\mathrm{ln}}(n)} \right)^\prime \left( {p_Ap_a + D_A} \right)^\prime + \frac{1}{2}\left( {p_Ap_a + D_A}

\right)^{\prime\prime}} \right)\end{array}.$$ (2) Note that previous studies address the steady-state distribution of gene frequency using the approximation of _D__A_ = _Fp__A__p__a_ where

inbreeding coefficient _F_ is equal to _α_/(2 − _α_) (Caballero and Hill 1992). This is reasonable because other components in _D__A_ have the second or higher orders of selection

coefficients or the dispersal variances and are neglected. Two scenarios are examined. One is that population density is uniformly distributed, and hence the terms with (ln(_n_))′ in Eqs.

(1) and (2) are equal to 0. The advantageous allele starts from the initial population and spreads by replacing the preexisting alleles (Fig. 2a), like the case addressed by Fisher (1937).

The second scenario is that the initial population colonizes new habitats along one-dimensional space (Fig. 2b). The advantageous gene spreads along with population colonization where

variation in density is present (ln(_n_))′ ≠ 0. In the second scenario, we assume that there is no effect of density-dependent regulation or environmental capacity on the number of pollen

and seeds in the gametophyte stage, but there is in the sporophyte stage. We assume that each population follows a logistical growth model. From Hu and He (2005), the change in population

density with time and space, _n_(_x_, _t_), can be described as follows: $$\dot n = \frac{{\sigma _S^2}}{2}n^{\prime\prime} + {\it{\epsilon }}n\left( {1 - \frac{n}{K}} \right),$$ (3) where

the first term represents the direct contribution from seed dispersal, the second term represents the logistic growth contribution in which _K_ is the environmental capacity and is the

per-capital growth rate derived as \({\it{\epsilon }} = \overline W - 1 = 2s_dp_A\) at position _x_ and time _t_ at a single selective locus. The maximum _∈_ is equal to 2_s__d_ when _p__A_ 

= 1, which is much smaller than 1.0 under weak selection. Oscillation in population size that requires a large _∈_ (say, _∈_ > 1) does not occur in the traveling waves simulated in the

next section. A more complex model is needed when capacities in the sporophyte stage differ among genotypes (Roughgarden 1979; Hu and He 2005). A NEUTRAL GENE LINKED WITH THE SELECTIVE GENE

Now, we describe a neutral locus B linked with the selective locus A, with alleles _B_ and _b_. The recombination rate between the two loci is designated by _r_ (0 ≤ _r_ ≤ 0.5). Let _p__ik_

and _p__ijkl_ be the frequencies of gamete _ik_ (_i_ = _A_,_a_; _k_ = _B_,_b_) and genotype _ijkl_ (_i_,_j_ = _A_,_a_; _k_,_l_ = _B_,_b_) in the current adult population at position _x_ and

time _t_. Let _D__AB_ (= _p__AB_ − _p__A__p__B_) be the LD in current adults between alleles _A_ and _B_ on the same gametes, and _D__A_/_B_ (=_p__A_/_B_ − _p__A__p__B_) be the LD in current

adults between alleles _A_ and _B_ on different gametes. Note that the forward slash “/” stands for alleles from separate gametes. The two digenic frequencies _p__AB_ and _p__A_/_B_ in

current adults are calculated by: $$p_{AB} = p_{AABB} + \left( {p_{AABb} + p_{AaBB} + p_{AB/ab}} \right)/2,$$ (4a) $$p_{A/B} = p_{AABB} + \left( {p_{AABb} + p_{AaBB} + p_{Ab/aB}}

\right)/2,$$ (4b) denote Δ_AB_ = _D__AB_ + _D__A_/_B_, a composite digenic disequilibrium in current adults (Weir 1996, p 126), and _δ__AB_ = 2(_D__AB_ − _D__A_/_B_), which equals twice the

difference between the frequencies of double heterozygotes at two loci in two linkage phases (_p__AB_/_ab_ − _p__Ab_/_aB_). Genotypic frequencies at two loci can be derived based on

Wright's (1969) approach that combines the selfing and random mating parts in the mixed mating system. From Eq. (A36) in SI Appendix, the change of allele frequency at the neutral locus

is given by: $$\begin{array}{l}\dot p_B = \widetilde \sigma ^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime p_B^\prime + \frac{{\widetilde \sigma ^2}}{2}p_B^{\prime\prime} + \left(

{s_h + s_d} \right)\\ \left( {\left( {1 - \alpha } \right)\left( {1 - r} \right)D_{AB} + \frac{{1 - 2r}}{4}\alpha \delta _{AB}} \right) + \alpha s_d\Delta _{AB}\end{array}.$$ (5) Strong

gametic LD (e.g., close linkage) enhances the trend toward concordant spread of selective and neutral genes in traveling waves. Composite LD (Δ_AB_) interacts with selection in the

sporophyte stage and imposes additional effects on neutral gene spread. To evaluate the spread of neutral genes, we need calculations of two digenic LDs (_D__AB_ and _D__A/B_). From Eq.

(A44) in SI Appendix, the change of gametic LD (_D__AB_) per generation is as follows: $$\begin{array}{l}\dot D_{AB} = - rD_{AB} - \frac{1}{2}\alpha r\delta _{AB} + \left( {1 - r}

\right)\widetilde \sigma ^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime D_{AB}^\prime \\ + \frac{{\widetilde \sigma ^2}}{2}\left( {2p_A^\prime p_B^\prime + \left( {1 - r}

\right)D_{AB}^{\prime\prime} } \right) - \frac{1}{2}\alpha r\sigma _S^2\left( {\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime \delta _{AB}^\prime + \frac{1}{2}\delta

_{AB}^{\prime\prime} } \right)\\ + s_h\left( \begin{array}{l}\left( {1 - \alpha } \right)\left( {p_a - p_A} \right)\left( {1 - r} \right)D_{AB}\\ + \frac{1}{4}\alpha \left( {p_{AaBB} +

p_{AB/ab} - \left( {p_A + \left( {p_a - p_A} \right)r} \right)\delta _{AB} - p_Bp_{Aa}} \right)\end{array} \right)\\ + s_d\left( {\left( {p_a - p_A} \right)\left( {1 - r} \right)D_{AB} +

\alpha \left( {\left( {1 - r} \right)D_{AB} - rp_a\delta _{AB} - p_A\Delta _{AB}} \right)} \right.\\ \left. { - \frac{1}{4}\alpha \left( {p_{AaBB} + p_{AB/ab} - r\delta _{AB} + \left( {1 -

2r} \right)p_A\delta _{AB} - p_Bp_{Aa}} \right)} \right)\end{array}.$$ (6) From Eq. (A45), the change of the digenic LD for the two genes on different gametes (_D__A/B_) is derived as

follows: $$\begin{array}{*{20}{l}}\displaystyle{{{\dot D}_{A/B}} = \frac{1}{2}a{\Delta _{AB}}-{D_{A/B}}+\frac{1}{2}\alpha \sigma _s^2{{(\ln (n))}^\prime}{\Delta

^\prime}_{AB}+\sigma_s^2(p_A^\prime p_B^\prime + \frac{1}{4}\alpha \Delta_{AB}^{\prime\prime})}\\\displaystyle{+ \frac{1}{4}\alpha {s_h}({p_{AABb}}+{p_{AB/ab}}-\left.{({p_A} +

({p_a}-{p_A})r){\sigma_{AB}}-{p_B}{p_{Aa}}}\right))}\\\displaystyle{+{s_d}(-2\alpha {p_A}{p_a}{p_B}+\alpha ({p_a}- {p_A}){\Delta _{AB}}-\frac{1}{4}\alpha({p_{A \alpha BB}}+{p_{Ab/\alpha

B}}+({p_A}+({p_a}-{p_A})r){\delta _{AB}}-{p_B}{p_{A\alpha }}))}\end{array}.$$ (7) Pollen flow does not directly change _D__A/B_ because each pollen grain (haploid) only carries one allele at

one locus, but directly affects _D__AB_ because each pollen grain carries one of four types of gametes (_AB_, _Ab_, _aB_, and _ab_). To evaluate two digenic LDs in the mixed mating system,

we need to calculate three genotype frequencies (_p__AaBB_, _p__AABb_, and the double heterozygotes with two linkage phases, _p__AB/ab_ and _p__Ab/aB_). Note that these three frequencies

together with the frequency of any genotype of double homozygotes (e.g., _p__AABB_) are independent among all nine two-locus genotypes. For simplicity, let \(\begin{array}{l}l_{AaBB} =

2\left( {1 - \alpha } \right)p_{AB}p_{aB} + \\ \frac{1}{2}\alpha \left( {p_{AaBB} + r\left( {1 - r} \right)\left( {p_{AB/ab} + p_{Ab/aB}} \right)} \right)\end{array}\) where _p__ik_(_i_ = 

_A,a_; _k_ = _B_,_b_) is the gametic frequency in pollen and ovules (next generation). _l__AaBB_ represents the frequency of genotype _AaBB_ that is derived under both random mating and

selfing but without effects of selection and gene flow. From Eq. (A38) of SI Appendix, the change of genotype frequency _p__AaBB_ is derived as follows: $$\begin{array}{l}\dot p_{AaBB} =

l_{AaBB} - p_{AaBB} + \left( {1 - \alpha } \right)\sigma _P^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime \left( {p_{AB}p_{aB}} \right)^\prime \\+ \frac{{\left( {1 - \alpha }

\right)\sigma _P^2}}{2}\left( {p_{AB}p_{aB}^{\prime\prime} + p_{AB}^{\prime\prime} p_{aB}} \right)\\ + \sigma _S^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime l_{AaBB}^\prime +

\frac{{\sigma _S^2}}{2}l_{AaBB}^{\prime\prime} \\+ 2s_h\left( {1 - \alpha } \right)\left( {p_a - p_A} \right)p_{AB}p_{aB} + s_d\left( {p_a - p_A} \right)l_{AaBB}\end{array}$$ (8) which can

be numerically calculated. Similarly, let \(l_{AABb} = 2\left( {1 - \alpha } \right)p_{AB}p_{Ab} + \frac{1}{2}\alpha \left( {p_{AABb} + r\left( {1 - r} \right)\left( {p_{AB/ab} + p_{Ab/aB}}

\right)} \right)\). From Eq. (A39) of SI Appendix, the recursive equation for genotypic frequency _p__AABb_ is derived as follows: $$\begin{array}{l}\dot p_{AABb} = l_{AABb} - p_{AABb} +

\left( {1 - \alpha } \right)\sigma _P^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime \left( {p_{AB}p_{Ab}} \right)^\prime \\+ \frac{{\left( {1 - \alpha } \right)\sigma

_P^2}}{2}\left( {p_{AB}p_{Ab}^{\prime\prime} + p_{AB}^{\prime\prime} p_{Ab}} \right)\\ + \sigma _S^2({\mathrm{ln}}\left( n \right))^\prime l_{AABb}^\prime + \frac{{\sigma

_S^2}}{2}l_{AABb}^{\prime\prime} \\+ 2s_h\left( {1 - \alpha } \right)\left( {p_a - p_A} \right)p_{AB}p_{Ab} + 2s_dp_al_{AABb}\end{array}.$$ (9) The frequency of genotype _AaBb_ is separately

considered in distinct linkage phases. Let \(l_{AB/ab} = 2\left( {1 - \alpha } \right)p_{AB}p_{ab} + \frac{1}{2}\alpha \left( {\left( {1 - r} \right)^2p_{AB/ab} + r^2p_{Ab/aB}} \right)\).

From Eq. (A40) of SI Appendix, the recursive equation for genotypic frequency _p__AB/ab_ is derived as follows: $$\begin{array}{l}\dot p_{AB/ab} = l_{AB/ab} - p_{AB/ab} + \left( {1 - \alpha

} \right)\sigma _P^2\left( {{\mathrm{ln}}\left(n \right)} \right)^\prime \\ \left( {p_{AB}p_{ab}} \right)^\prime + \frac{{\left( {1 - \alpha } \right)\sigma _P^2}}{2}\left(

{p_{AB}p_{ab}^{\prime\prime} + p_{AB}^{\prime\prime} p_{ab}} \right)\\ + \sigma _S^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime l_{AB/ab}^\prime + \frac{{\sigma

_S^2}}{2}l_{AB/ab}^{\prime\prime} \\+ 2s_h\left( {1 - \alpha } \right)\left( {p_a - p_A} \right)p_{AB}p_{ab} + s_d\left( {p_a - p_A} \right)l_{AB/ab}\end{array}.$$ (10) Let \(l_{Ab/aB} =

2\left( {1 - \alpha } \right)p_{Ab}p_{aB} + \frac{1}{2}\alpha \left( {\left( {1 - r} \right)^2p_{Ab/aB} + r^2p_{AB/ab}} \right)\). From Eq. (A41) of SI Appendix, the recursive equation for

genotypic frequency _p__Ab/aB_ is derived as follows: $$\begin{array}{l}\dot p_{Ab/aB} = l_{Ab/aB} - p_{Ab/aB} + \left( {1 - \alpha } \right)\sigma _P^2\left( {{\mathrm{ln}}\left( n \right)}

\right)^\prime \left( {p_{Ab}p_{aB}} \right)^\prime \\ + \frac{{\left( {1 - \alpha } \right)\sigma _P^2}}{2}\left( {p_{Ab}p_{aB}^{\prime\prime} + p_{Ab}^{\prime\prime} p_{aB}} \right) +

\sigma _S^2\left( {{\mathrm{ln}}\left( n \right)} \right)^\prime l_{Ab/aB}^\prime \\+ \frac{{\sigma _S^2}}{2}l_{Ab/aB}^{\prime\prime} + 2s_h\left( {1 - \alpha } \right)\left( {p_a - p_A}

\right)p_{Ab}p_{aB} + s_d\left( {p_a - p_A} \right)l_{Ab/aB}\end{array}.$$ (11) Thus, the spread of neutral genes can be thoroughly assessed from Eqs. (5) to (11). The dynamics of composite

digenic LD is calculated according to the relationships of \(\dot \Delta _{AB} = \dot D_{A/B} + \dot D_{AB}\) and \(\dot \delta _{AB} = 2\left( {\dot D_{AB} - \dot D_{A/B}} \right)\).

SIMULATIONS METHODS Two approaches are separately applied to assess the wave of advance of both advantageous and neutral genes. The first approach is based on analytical calculations

described above and uses the forward-finite difference method to investigate the waves of gene advance. The initial population is fixed in density (_n_0) and genetic composition. The step

size is set as _dx_ = 1 for the space and _dt_ = 1 for the time (generation). The dispersal variances are set so that the values for \(\left( {\sigma _S^2/2} \right)\partial t/\left(

{\partial x} \right)^2\) and \(\left( {\sigma _P^2/2} \right)\partial t/\left( {\partial x} \right)^2\) are smaller than 1, leading to stable simulations (DuChateau and Zachmann 1989, p

352–365). The second approach is based on MC simulation where a one-dimensional stepping-stone model is used to simulate gene flow among populations (Kimura 1953). The initial population is

stable in density and genetic composition. Each population follows the same life cycle (Fig. 1) together with additional genetic drift after sporophytic selection. In the scenario of uniform

density (Fig. 2a), the MC simulation considers all populations are initially occupied by genotype _aabb_. A mutant allele _A_ of adaptive advantage over allele _a_ and its linked neutral

allele _B_ spread along the one-dimensional space. We assume that allele _A_ has attained a high frequency in the initial population due to selection, and so has the neutral allele _B_

linked with allele _A_ due to genetic hitchhiking effects (Barton 2000). This assumption ensures that the advantageous _A_ allele has a very small probability of going extinct in the initial

population. Fixation of allele _A_ in the initial population is an extreme case. Exchanges of migrants between two nearest-neighbor demes are allowed, each with migration rates of _m__S_/2

and _m__P_/2 for seeds and pollen, respectively. Genotypic frequencies after selection in the sporophyte stage are randomly sampled according to the multinomial distribution of genotypic

frequencies, given the population size. In the scenario of nonuniform density (Fig. 2b), the MC simulation considers that the initial population colonizes new habitats along one-dimensional

homogeneous space. Dispersal of seeds and pollen (_m__S_ and _m__P_) occurs between the nearest-neighbor populations. Joint changes in population density and gene frequency are examined.

Each derived local population originates from immigrant seeds from its nearest-neighbor population that was established earlier. When a derived population starts (for simulation purpose,

say, _n_(_x_,_t_) ≥ 1), we consider both seed and pollen flow between nearest-neighbor populations. The logistical growth model (the second term in Eq. (3)) is used in each population.

Gametic and allelic frequencies in migrants of pollen and seeds are assumed to be the same as in the donor populations. Genotypic frequencies after selection in the sporophyte stage are

randomly sampled according to the multinomial distribution of genotypic frequencies with the obtained population size. One thousand independent data sets are generated for a particular set

of simulation conditions, and each data set is used to estimate mean and standard deviations of different variables within each population. Results are extracted at 50 generation intervals

for analysis. Scripts in _C_ have been submitted to Dryad. SPREAD OF ADVANTAGEOUS GENES In the scenario of uniform population density (Fisher 1937), i.e. (ln(_n_))′ = 0, the advantageous

allele _A_ advances in theory at a constant speed to replace the previous allele _a_ and maintains the same shape of distribution (Shigesada and Kawasaki 1997). Let _c_0 be the rate at which

allele _A_ spreads under outcrossing (_α_ = 0). The speed _c_0 is calculated according to Fisher (1937) and Kolmogorov et al. (1937): $$c_0 = \left( {2\left( {s_h + s_d} \right)\left(

{\sigma _S^2 + \sigma _P^2/2} \right)} \right)^{1/2}.$$ (12) The speed _c_0 can be derived by application of the method provided by Shigesada and Kawasaki (1997, p 57–60). The speed

positively correlates with selection coefficients in both gametophyte and sporophyte stages. When selfing is the major process generating HWD in comparison with gene flow and selection, a

stable HWD coefficient can be achieved and is approximated by \(D_A = \frac{\alpha }{{2 - \alpha }}p_Ap_a\) from Eq. (2). According to Shigesada and Kawasaki (1997, p 57–60), substitution of

_D__A_ into Eq. (1) yields the speed _c__α_ as follows: $$c_\alpha = \left( {2\left( {s_d + s_h + \left( {s_d - s_h} \right)\frac{\alpha }{{2 - \alpha }}} \right)\left( {\sigma _S^2 +

\frac{{1 - \alpha }}{2}\sigma _P^2} \right)} \right)^{1/2}$$ (13) indicating a very complicated relationship between the selfing rate and speed under different levels of pollen and seed

dispersal. To look at the effects of selfing rate on the speed, we take the derivative of _c__α_ with respect to _α_ (suggestion of one anonymous reviewer): $$\frac{{\partial c_\alpha

}}{{\partial \alpha }} = \frac{1}{{\left( {2 - \alpha } \right)^2c_\alpha }}\left( {2\sigma _S^2\left( {s_d - s_h} \right) - \sigma _P^2\left( {s_d + s_h\left( {3 - \alpha } \right)\left( {1

- \alpha } \right)} \right)} \right).$$ (14) It can be inferred that self-fertilization impedes gene flow (\(\frac{{\partial c_\alpha }}{{\partial \alpha }}\,<\,0\)) when the condition

of \(\frac{{\sigma _P^2}}{{\sigma _S^2}} > \frac{{2(1 - s_h/s_d)}}{{1 + \left( {3 - \alpha } \right)\left( {1 - \alpha } \right)s_h/s_d}}\) is met, but enhances gene flow

(\(\frac{{\partial c_\alpha }}{{\partial \alpha }}\,>\, 0\)) when \(\frac{{\sigma _P^2}}{{\sigma _S^2}} < \frac{{2\left( {1 - s_h/s_d} \right)}}{{1 + \left( {3 - \alpha } \right)\left(

{1 - \alpha } \right)s_h/s_d}}\). Self-fertilization always impedes gene flow when _s__d_ < _s__h_. The maximum _c__α_ is related to the relative values of selection coefficients and

pollen/seed dispersal variances. In the scenario of uniform population density, SI Fig. 1a shows the traveling wave of advantageous alleles based on Eqs. (1) and (2). The HWD coefficient

exhibits one unimodal distribution (SI Fig. 1b), with the maximum occurring at around the middle of the wave front. Figure 3a, b shows that gene spread decreases, while the maximum HWD

coefficient increases as the selfing rate increases from _α_ = 0 to 1.0, indicating that selfing impedes the spread of advantageous genes. Alternatively, when selection coefficients are

negative, allele _A_ is maladaptive to the habitat compared with allele _a_. If the initial population is fixed by allele _A_, gene spread is counteracted by selection, eventually resulting

in a limited range of distribution. SI Fig. 2 shows the limitation of gene spread at _t_ = 500 under uniform population density and maladaptive allele _A_ (_s__h_ = −0.01 and _s__d_ = 

−0.02). The rate of gene spread tends to decrease as the selfing rate increases. When only gametophytic selection occurs (_s__h_ ≠ 0, _s__d_ = 0), gene spread decreases as the selfing rate

increases under a given rate of seed flow (Fig. 4a for the case of uniform population density), indicating that a greater proportion of pollen discounting due to the joint effects of sefling

and gametic selection can substantially impede gene spread. This also confirms the relation of ∂_c__α_/∂_α_ < 0 in the presence of gametic selection only. The maximum HWD increases as

the selfing rate increases (Fig. 4b). When only sporophytic selection occurs (_s__h_ = 0, _s__d_ ≠ 0), selfing slightly regulates the wave of gene advance. For instance, Fig. 4c shows that

selfing enhances gene spread to some extent under certain levels of seed flow. The pattern is consistent with ∂_c__α_/∂_α_ > 0 under this parameter setting. The maximum HWD increases as

the selfing rate increases (Fig. 4d). Note allele _A_ does not spread without seed dispersal in the selfing system. In the absence of pollen flow (\(\sigma _P^2 = 0.0\)) but presence of seed

dispersal (e.g., \(\sigma _S^2 = 0.04\) in Fig. 4e, f), selfing discounts outcross pollen from resident populations and facilitates gene spread, which is consistent with the result of

∂_c__α_/∂_α_ > 0 under \(\sigma _P^2 = 0\) and _s__d_ > _s__h_. MC simulations indicate that patterns for selfing effects are qualitatively similar between the stochastic and

deterministic processes except that their traveling waves fluctuate under genetic drift. Selfing impedes the wave of advance of advantageous genes. For instance, the wave of advance of the

advantageous allele has the largest rate of speed under random mating (_α_ = 0) but the smallest rate under selfing (_α_ = 1) (Fig. 5a). The maximum value of standard deviation occurs at

around _p__A_ = 0.5 since the variance of allele frequency is proportional to _p__A_(1 − _p__A_) (Weir 1996, p 42). Also, the position with the largest standard deviations of allele

frequency moves forward along the spread of the advantageous allele as the selfing rate decreases (Fig. 5b). MC simulations indicate that genetic drift effects shape the waves of advance of

the advantageous allele. SI Fig. 3a shows that the wave of gene advance speeds up as population size increases. In addition, as the population size increases, the maximum standard deviation

of the position of gene advance decreases (SI Fig. 3b). In the scenario of nonuniform population density, i.e. (ln(_n_)′ ≠ 0), both population density and gene frequency exhibit traveling

waves (SI Fig. 1a–c). Patterns regarding the effects of selfing rate are qualitatively similar between the two scenarios except that variation in population density enhances the rate of gene

spread. For instance, gene spread decreases as the selfing rate increases (Fig. 3c). The HWD distribution shrinks slightly as the selfing rate increases from 0 to 1.0 (Fig. 3d). This is

because pollen dispersal affects population density and HWD by changing the spatial distribution of the frequencies of adaptive alleles. MC simulations also confirm that selfing impedes the

wave of advance of advantageous alleles. Selfing restricts colonization in the presence of gametophytic selection (Hu et al. 2019). For instance, population density exhibits a traveling wave

with the largest rate of spread under random mating (Fig. 6a), and so does the frequency of advantageous alleles (Fig. 6c). As the selfing rate increases, the standard deviations increase

for the waves of advance of population density and advantageous allele frequency (Fig. 6b, d). This is because the uncertainty increases when genetic drift effects are high (a small

population density) and the allele frequency _p__A_ is low (<<0.5), but is reduced when density is large and _p__A_ is high (>>0.5). SPREAD OF NEUTRAL GENES Numerical simulations

for neutral genes are based on Eqs. (5)–(11). Though accompanying the traveling wave of advantageous alleles, the wave of advance of neutral genes gradually declines under both the scenario

of uniform ((ln(_n_))′ = 0) and nonuniform ((ln(_n_))′ = 0) population density (Fig. 7a, b). This is associated with the wave of advance of the two digenic LDs (_D__AB_ and _D__A/B_) that

are gradually weakened with time owing to recombination (Fig. 7c, d). The genetic hitchhiking effects are broken down by recombination as the advantageous allele spreads. _D__AB_ is greater

in value than _D__A/B_ although both LDs exhibit an unimodal distribution. Like the wave of advance of the advantageous allele, variation in population density promotes wave advance of the

neutral genes through strengthening LDs. The recombination rate between selective and neutral loci is crucial in determining the wave of advance of the neutral gene. For instance, spread of

the neutral gene quickly slows down as the recombination rate increases from 0.01 to 0.5 under both the scenario of uniform and nonuniform population density (SI Fig. 4a, d). The two digenic

LDs also quickly decrease (SI Fig. 4b, c, e, f), and the spread of neutral genes mainly relies on the diffusion process under loose linkage. Thus, only those neutral loci that are tightly

linked with the selective locus tend to follow the wave of advantageous genes due to strong gametic LDs (Fig. 7). The difference between the two scenarios lies in the fact that variation in

population density enhances both digenic LDs and weakens the effects of recombination. Selfing impedes the spread of neutral genes under both the scenario of uniform (Fig. 8b, c) and

nonuniform (Fig. 8e, f) population density. Such effects are substantial for the neutral locus that is tightly linked with a selective locus when pollen dispersal is much stronger than seed

dispersal (\(\sigma _P^2 \ge \sigma _S^2\)). Like the spread of advantageous alleles, random mating (_α_ = 0) maintains the largest rate of spread of neutral genes. In the scenario of

uniform population density, MC simulations confirm that the average traveling wave of neutral genes is similar under genetic drift to that under deterministic processes. Selfing impedes the

rate of neutral gene spread. For instance, the average wave of advance of neutral genes slows down as the selfing rate increases from _α_ = 0 to 1 (Fig. 5c). The maximum values of standard

deviation occur at around _p__B_ = 0.5. Positions with the largest standard deviation of allele frequency move forward along the spread of the advantageous allele as the selfing rate

decreases (Fig. 5d). Also, MC simulations indicate that genetic drift effects shape the average wave of advance of neutral genes. The average wave of neutral genes speeds up as the

population size increases (SI Fig. 3c). The maximum standard deviation of the traveling waves decreases as the population size increases (SI Fig. 3d). In the scenario of nonuniform

population density, MC simulations confirm that selfing impedes the wave of advance of neutral genes. Although selfing initially enhances the spread of neutral genes owing to LD reinforced

by selfing, the spread of neutral gene quickly slows down as the spread of the advantageous allele decreases with the selfing rate (Fig. 6e). As the selfing rate increases from _α_ = 0 to 1,

the standard deviation increases for the wave of advance of neutral genes (Fig. 6f). Similarly, the uncertainty is high when population density is low (large genetic drift effects) and

allele frequency _p__B_ is low, but is reduced when density is large and _p__B_ is high (say, >>0.5). MC simulations indicate that recombination slightly affects the wave of neutral

gene spread when a certain level of seed dispersal is involved in population colonization. This is similar to the results in the scenario of uniform density. For instance, the neutral gene

generally exhibits a pattern similar to that of the advantageous allele under different recombination rates (SI Fig. 6a, c). Nevertheless, neutral genes spread more extensively when they are

more closely linked with the selective locus (SI Fig. 6c). Large fluctuations occur in frontal waves of gene frequencies for both advantageous and neutral genes due to genetic drift effects

(SI Fig. 6b, d). DISCUSSION Since Fisher’s (1937) pioneering work, there have been extensive studies on the rate of spread of advantageous and neutral genes under random mating. The present

study examines the role of mating systems in affecting the spread of advantageous and neutral genes, and provides an addition to the extant theories where effects of selfing are not

considered. Selfing reduces outcross pollen from both immigrants and resident populations. The automatic transmission advantage of selfers over outcrossers (Fisher 1941) ensures that selfing

is more effective than outcrossing in impeding gene dispersal. Two essential processes in our theory remain the same as Fisher (1937) and Haldane (1948), i.e., the migration (seed and

pollen dispersal) and selection (gametophytic and sporophytic selection). An additional stochastic process is considered in MC simulations. Since mating systems play a critical role in

structuring genetic variation within and between populations, it is important in both practice and theory to elucidate how selfing regulates the spread of advantageous and neutral genes

within a species. A few specific conclusions are summarized as follows. One is that a complex relationship exists between selfing rate and the speed of gene spread. The interaction of

selfing with gametophytic selection is crucial in determining the role of mating systems in regulating the wave of advance of advantageous genes. Selfing can impede (or promote) the spread

of advantageous genes in the presence (or absence) of gametophytic selection. This is similar to the role of selfing in regulating a species’ range (Hu et al. 2019). The second conclusion is

that the stochastic process (genetic drift) does not alter the pattern of selfing effects except for increasing the uncertainty of the wave of advance for both advantageous and neutral

genes. The third conclusion is that the interaction of selfing with recombination affects the rate of neutral gene spread, given the migration-selection processes for spreading advantageous

genes. When selfing impedes the spread of advantageous genes, it also restricts the spread of the tightly linked neutral gene due to LD generated by both selfing and pollen/seed dispersal.

The waves of advance of neutral genes gradually go down along the direction of spread of advantageous genes as the genetic hitchhiking effects are broken down by recombination with time. The

fourth conclusion is that, given a particular mating system, variation in population density enhances the spread of advantageous genes and their tightly linked neutral genes. This also

tends to weaken the recombination effects. The above results are applicable to interpreting how selfing impedes or enhances the spread of both advantageous and neutral genes in the natural

distribution of a plant species. Concerned with the spread of advantageous genes, previous studies have examined the effects of different barriers, including population subdivision (Slatkin

1976; Slatkin and Charlesworth 1978), physical obstacles, and random genetic drift effects (Pialek and Barton 1997; Barton 2000). Using the simulation approach, Slatkin and Charlesworth

(1978) showed that the wave of advantageous alleles going to fixation can be quickly attained in a subdivided population, but stochastic effects reduce the wave speed. Pialek and Barton

(1997) also confirmed the effects of random drift on delaying the spread of an advantageous allele in a separate model, as does the effect of selection against heterozygotes. The present

study further expands our understanding of the role of mating systems in impeding the spread of advantageous genes. Although the interaction of selfing with physical barriers (Hu 2015) or

with isolation by distance via reducing neighbor size (Wright 1946) is not examined in this study, this interaction could reinforce the impediment to gene flow. A physical barrier may delay

the spread of neutral genes (Barton 1979). With genetic hitchhiking effects, Slatkin and Charlesworth (1978) showed that the probability (_Pi_) of new alleles occurring in _i_ populations is

similar for detrimental and neutral genes under interaction of gene flow with selection and drift. When migration rate is smaller than selection strength, Slatkin and Wiehe (1998) showed

that genetic hitchhiking enhances population differences at neutral loci that are closely linked to advantageous genes. Under similar condition, Kim and Maruki (2011) showed that population

subdivision diminishes genetic hitchhiking effects because the breakdown by recombination is enhanced in the case of restricted spread of advantageous genes. We here demonstrate new insights

into the spread of neutral genes: (1) selfing impedes the spread of neutral genes; (2) variation in population density enhances neutral gene flow for a given mating system. The difference

between scenarios of uniform vs. nonuniform population density is that the latter has an additional contribution to gene flow due to unequal population sizes. In the scenario of uniform

population density (Fisher 1937), i.e. (ln(_n_))′ = 0, each population is already established in space. Advantageous genes spread through seed and pollen dispersal and replace the

preexisting disadvantageous alleles through natural selection in any local population. In the scenario of nonuniform density, i.e. (ln(_n_))′ ≠ 0, spread of adaptive genes and species

colonization in new habitats co-evolve because fitness at multiple loci is the genetic basis of population growth and adaptation to local environments. Asymmetric dispersal from dense to

less dense populations ((ln(_n_))′ > 0) also tends to increase frequency of adaptive genes (_p__A_′ > 0) or the frequency of linked neutral genes (_p__B_′ > 0), or vice versa (i.e.,

if (ln(_n_))′ < 0, then _p__A_′ < 0 and _p__B_′ < 0) (Kirkpatrick and Barton 1997). High frequencies of advantageous genes enhance population growth. Thus, spread of adaptive

alleles follows or is concordant with the expansion of species range (Hu et al. 2019). Thus, variation in population density produces a larger gradient for adaptive allele frequencies, which

enhances gene spread, than does uniform population density. With our theory, we assume that the mutant allele with advantage over the preexisting allele at a diallelic locus has attained a

high frequency or become fixed before spreading out to neighboring populations (Fisher 1937). This is plausible for a large population where an adaptive mutant allele becomes extinct with a

small probability (Wright 1969). For a small population, achievement of high frequencies of the advantageous allele is conditional on the ultimate fixation probability where the mutant

allele is not lost in the genetic drift process (Haldane 1927; Kimura 1962; Barton 2000). Thus, the assumption that the copies of the advantageous allele exceed a certain level is needed so

that the extinction of this allele is rare. Also, in the presence of the mixed mating system, selfing reduces the efficacy of selection (Glemin 2007) and hence could modify the fixation

probability of the mutant allele in a population. This ultimate fixation probability could change with the selfing rate. Consequently, the waiting time for the mutant allele to reach

fixation should be different between the systems with and without selfing besides the effects due to genetic drift. Several implications can be derived from the present theory. The first

implication concerns the role of selfing in advancing or impeding the spread of an advantageous gene in the distribution of a species. Selfing regulates the rate of spread of advantageous

genes through two approaches. One is through its interaction with gametophytic selection. The antagonistic relationship between two stages of selection in one life cycle leads to their

distinct interactions with selfing rate. This is an indirect form of regulation, like the role of selfing in regulating a species’ range since only selective alleles are associated with

population growth and adaption to habitats (Hu et al. 2019). The second effect is to regulate the proportion of migrant pollen that combines with ovules, which is a form of regulation and

could be more efficient than the indirect way. The two approaches regulate the speed of gene spread in complex ways. A nonlinear relationship exists between the speed and the selfing rate,

as implied from Eq. (13). Given a constant rate of gene spread, a lower level of gene flow can effectively meet the requirement for the spread of alleles with a higher selective advantage

than the existing alleles. Alternatively, a high level of gene flow is needed for the spread of an allele with a lower selective advantages than those already established. Thus, evolution of

mating systems from outcrossing to selfing implicates that different rates of selfing are applied to regulate the rate of spreading adaptive genes in different species (Barrett 2014). This

is crucial to maintain species integrity or cohesion since the spread of selective genes is more effective in preventing reproductive isolation between genetically different pools of

populations (Slatkin 1976). This is different from neutral gene flow that is often estimated and applied to infer population history. If a certain level of exchange of genes responsible for

reproductive isolation is needed to maintain species integrity, the theory could provide a basis for explaining how a low level of such gene flow is sufficient for alleles to spread across

the entire species (Morjan and Rieseberg 2004; Lowe and Allendorf 2010). The second implication concerns a better understanding of the spread of neutral genes. Previous studies imply that a

neutral gene can eventually spread across the whole species, regardless of the presence or absence of physical barriers (Barton 1979; Barton and Bengtsson 1986). Genetic hitchhiking can

generate transient traveling waves or clines of neutral genes in both nuclear and cytonuclear systems (Slatkin and Wiehe 1998; Barton 2000; Hu 2008). The present theory shows that selfing

reinforces the maintenance of the transient traveling waves of neutral genes although the pattern of the frontal waves is reduced as the genetic hitchhiking effects break down with time. It

is predicted that transient waves of neutral genes could last longer than that under random mating where LD is generated by gene flow only. Such a difference could be more substantial for

species with selfing or mixed mating systems than for species with random mating. Practical observations of multilocus waves or clines of gene frequencies among populations at both selective

and neutral marker loci could likely signal the reinforcement of genetic hitchhiking from selfing. The third result to emerge from our models is that they predict potential irregular waves

of advance of advantageous genes under heterogeneous habitats (Shigesada and Kawasaki 1997) or under variation in mating systems. This could arise from variation in diffusion coefficients

(e.g., environment-dependent diffusion for pollen \(\sigma _P^2\left( x \right)\) or seeds \(\sigma _S^2\left( x \right)\) dispersal) and/or selection coefficients (e.g.,

environment-dependent selection in gametophytic _s__h_(_x_) or sporophytic _s__d_(_x_) stage) under heterogeneous habitats. It could also arise from the influence of some factors (e.g.,

population density and environmental factors) that cause variation of mating systems among populations (Whitehead et al. 2018; Zhang et al. 2019). The selfing rate likely changes at

different geographic positions (e.g., _α_(_x_)), and hence could unequally affect the rate of gene spread in different populations. Nevertheless, the function of selfing should remain the

same in interacting with selection and gene flow except that the wave becomes irregular under diverse selfing rates or the wave of gene advance stops in unfavorable environments. The fourth

implication of our models concerns the relationship between population density (_n_) and gene flow that has been investigated in empirical studies and exhibits mixed patterns. Although the

direction of gene flow from less dense to dense populations is recorded under heterogeneous conditions (Kennington et al. 2003; Palstra et al. 2007), more reports are of asymmetrical gene

flow from dense to less dense populations ((ln(_n_))′ > 0 and _p__A_′ > 0). This is supported by studies in both animal (Fraser et al. 2004; Manier and Arnold 2005) and plant species

(Nagamitsu et al. 2014). The pattern is also conceptually related to an extreme case of classical mainland-island model or the source-sink model in metapopulation dynamics (Hanski and

Gaggiotti 2004) where unidirectional gene flow takes place from mainland to island populations. A common phenomenon in tropical forest is the habitat fragmentation that creates many

isolating patches with various population densities of a tree species. Although there are controversial issues about fragmentation and gene flow (Stephanie et al. 1992; White et al. 2002;

Sebbenn 2011), the asymmetric population sizes could be an important factor, given a physical distance or presence/absence of physical barriers between fragments. Our theory implies that

asymmetric population densities enhance gene flow among fragments, provided that long-distance dispersal is neglected. So far, this perspective has rarely been emphasized in the literature

in explaining genetic structure and gene flow among fragments. DATA AVAILABILITY The codes used for simulations have been submitted to Dryad (https://doi.org/10.5061/dryad.83bk3j9p0).

REFERENCES * Barrett SCH (2014) Evolution of mating systems: outcrossing versus selfing. In: Losos J (ed.) The Princeton guide to evolution. Princeton University Press, Princeton, NJ, p

356–362 Google Scholar  * Barton NH (1979) Gene flow past a cline. Heredity 43:333–340 Google Scholar  * Barton NH (2000) Genetic hitchhiking. Philos Trans R Soc Lond Ser B Biol Sci

355:1553–1562 CAS  Google Scholar  * Barton NH, Bengtsson BO (1986) The barrier to genetic exchange between hybridizing populations. Heredity 57:357–376 PubMed  Google Scholar  * Caballero

A, Hill WG (1992) Effective size of non-random mating populations. Genetics 130:909–916 CAS  PubMed  PubMed Central  Google Scholar  * Charlesworth D (2006) Evolution of plant breeding

systems. Curr Biol 16:R726–R735 CAS  PubMed  Google Scholar  * Charlesworth D, Charlesworth B (1992) The effects of selection in the gametophytic stage on mutational load. Evolution

46:703–720 CAS  PubMed  Google Scholar  * Charlesworth D, Willis JH (2009) The genetics of inbreeding depression. Nat Rev Genet 10:783–796 CAS  PubMed  Google Scholar  * Darwin C (1876) The

effects of cross- and self-fertilization in vegetable kingdom. Murray, London Google Scholar  * Damgaard C, Guldbrandtsen B, Christiansen FB (1994) Male gametophytic selection against a

deleterious allele in a mixed mating model. Hereditas 120:13–18 Google Scholar  * Davis HG, Taylor CM, Lambrinos JG, Stong DR (2004) Pollen limitation causes an Allee effect in a

wind-pollinated invasive grass (_Spartina alterniflora_). Proc Natl Acad Sci USA 101:13804–13807 * DuChateau P, Zachmann D (1989) Applied partial differential equations. Harper & Row

Publisher, New York, NY Google Scholar  * Ennos RA (1994) Estimating the relative rates of pollen and seed migration among plant populations. Heredity 72:250–259 Google Scholar  * Fisher RA

(1937) The wave of advance of advantageous genes. Ann Eugen 7:355–369 Google Scholar  * Fisher RA (1941) Average excess and average effect of a gene substitution. Ann Eugen 11:53–63 Google

Scholar  * Fraser DJ, Lippe C, Bernatchez L (2004) Consequences of unequal population size, asymmetric gene flow and sex-biased dispersal on population structure in brook charr (_Salvelinus

fontinalis_). Mol Ecol 13:67–80 CAS  PubMed  Google Scholar  * Glemin S (2007) Mating systems and the efficacy of selection at the molecular level. Genetics 177:905–916 PubMed  PubMed

Central  Google Scholar  * Groom MJ (1998) Allee effects limit population viability of an annual plant. Am Nat 151:487–496 CAS  PubMed  Google Scholar  * Grossenbacher DL, Runquist RB,

Goldberg EE, Brandvain Y (2015) Geographic range size is predicted by plant mating system. Ecol Lett 18:706–713 PubMed  Google Scholar  * Haldane JBS (1927) A mathematical theory of natural

and artificial selection. Part V: selection and mutation. Math Proc Camb Philos Soc 23:838–844 Google Scholar  * Haldane JBS (1948) Theory of a cline. J Genet 28:227–284 Google Scholar  *

Hanski I, Gaggiotti O (2004) Ecology, genetics and evolution of metapopulations. Elsevier Academic Press, London Google Scholar  * Hu XS (2008) Barriers to the spread of neutral alleles in

the cytonuclear system. Evolution 62:2260–2278 PubMed  Google Scholar  * Hu XS (2011) Mating system and the critical migration rate for swamping selection. Genet Res 93:233–254 CAS  Google

Scholar  * Hu XS (2015) Mating system as a barrier to gene flow. Evolution 69:1158–1177 CAS  PubMed  Google Scholar  * Hu XS, He FL (2005) Background selection and population

differentiation. J Theor Biol 235:207–219 PubMed  Google Scholar  * Hu XS, Li B (2003) On migration load of seeds and pollen grains in a local population. Heredity 90:162–168 PubMed  Google

Scholar  * Hu XS, Zhang XX, Zhou W, Hu Y, Wang X, Chen XY (2019) Mating system shifts a species’ range. Evolution 73:158–174 PubMed  Google Scholar  * Igic B, Kohn JR (2006) The distribution

of plant mating systems: study bias against obligately outcrossing species. Evolution 60:1098–1103 PubMed  Google Scholar  * Johnston MO, Porcher E, Cheptou PO, Eckert CG, Elle E, Geber MA

et al. (2009) Correlations among fertility components can maintain mixed mating in plants. Am Nat 173:1–11 PubMed  Google Scholar  * Kennington WJ, Gockel J, Partridge L (2003) Testing for

asymmetrical gene flow in a _Drosophila melanogaster_ body-size cline. Genetics 165:667–673 CAS  PubMed  PubMed Central  Google Scholar  * Kim Y, Maruki T (2011) Hitchhiking effect of a

beneficial mutation spreading in a subdivided population. Genetics 189:213–226 CAS  PubMed  PubMed Central  Google Scholar  * Kimura M (1953) “Stepping-stone” model of population. Annu Rep

Natl Inst Genet 3:62–63 Google Scholar  * Kimura M (1962) On the probability of fixation of mutant genes in a population. Genetics 47:713–719 CAS  PubMed  PubMed Central  Google Scholar  *

Kirkpatrick M, Barton NH (1997) Evolution of a species’ range. Am Nat 150:1–23 CAS  PubMed  Google Scholar  * Kolmogorov A, Petrovsky N, Picoounov NS (1937) A study of the equation of

diffusion with increase in the quantity of matter, and its application to a biological problem. Mosc Univ Bull Math 1:1–25 Google Scholar  * Kohn JR, Barrett SCH (1994) Pollen discounting

and the spread of selfing variant in tristylocus _Eichhornia paniculata_: evidence from experimental populations. Evolution 48:1576–1594 PubMed  Google Scholar  * Lowe WH, Allendorf FW

(2010) What can genetics tell us about connectivity? Mol Ecol 19:30038–30051 Google Scholar  * Manier MK, Arnold SJ (2005) Population genetic analysis identifies source-sink dynamics for two

sympatric garter snake species (_Thamnophis elegans_ and _Thamnophis sirtalis_). Mol Ecol 14:3965–3976 PubMed  Google Scholar  * Morjan CL, Rieseberg LH (2004) How species evolve

collectively: implications of gene flow and selection for the spread of advantageous alleles. Mol Ecol 13:1341–1356 CAS  PubMed  PubMed Central  Google Scholar  * Nagamitsu T, Kikuchi S,

Hotta M, Kenta T, Hiura T (2014) Effects of population size, forest fragmentation, and urbanization on seed production and gene flow in an endangered maple (_Acer miyabei_). Am Naturalist

172:303–316 Google Scholar  * Nagylaki T (1975) Conditions for existence of clines. Genetics 80:595–615 PubMed Central  Google Scholar  * Nordborg M (2000) Linkage disequilibrium, gene trees

and selfing: an ancestral recombination graph with partial self-fertilization. Genetics 154:923–929 CAS  PubMed  PubMed Central  Google Scholar  * Nordborg M, Charlesworth B, Charlesworth D

(1996a) The effect of recombination on background selection. Genet Res 67:159–174 CAS  PubMed  Google Scholar  * Nordborg M, Charlesworth B, Charlesworth D (1996b) Increased levels of

polymorphism surrounding selectively maintained sites in highly selfing species. Proc Biol Sci 263(1373):1033–1039. * Otto SP, Marks JC (1996) Mating systems and the evolutionary transition

between haploidy and diploidy. Biol J Linn Soc 57:197–218 Google Scholar  * Palstra FP, O'Connell MF, Ruzzante DE (2007) Population structure and gene flow reversals in Atlantic salmon

(_Salmo salar_) over contemporary and long-term temporal scales: effects of population size and life history. Mol Ecol 16:4504–4522 CAS  PubMed  Google Scholar  * Peters MAE, Weis AE (2018)

Selection for pollen competitive ability in mixed-mating systems. Evolution 72:2513–2536 PubMed  Google Scholar  * Pialek J, Barton NH (1997) The spread of an advantageous allele across a

barrier: the effects of random drift and selection against heterozygotes. Genetics 145:493–504 CAS  PubMed  PubMed Central  Google Scholar  * Pickup M, Brandvain Y, Fraisse C, Yakimowski S,

Barton NH, Dixit T et al. (2019) Mating system variation in hybrid zones: facilitation, barriers and asymmetries to gene flow. N Phytol 224:1035–1047 Google Scholar  * Razanajatovo M, Maurel

N, Dawson W, Essl F, Kreft H, Pergl J et al. (2016) Plants capable of selfing are more likely to become naturalized. Nat Commun 7:13313 CAS  PubMed  PubMed Central  Google Scholar  *

Roughgarden J (1979) Theory of population genetics and evolutionary ecology: an introduction. Macmillan Publishing Co., Inc, New York, NY Google Scholar  * Sebbenn A (2011) Effects of forest

fragmentation on the effective and realized gene flow of Neotropical tree species: implications for genetic conservation. BMC Proc 5(7):1–2 Google Scholar  * Shaw AJ, Szovenyi P, Shaw B

(2011) Bryophyte diversity and evolution: windows into the early evolution of land plants. Am J Bot 98(2):352–369 PubMed  Google Scholar  * Shigesada N, Kawasaki K (1997) Biological

Invasions: theory and Practice. Oxford University Press, Oxford Google Scholar  * Slatkin M (1976) The rate of spread of an advantageous allele in a subdivided population. In: Karlin S, Nevo

E (eds) Population genetics and ecology. Academic Press, Inc, New York, NY, p 767–780 Google Scholar  * Slatkin M, Charlesworth D (1978) The spatial distribution of transient alleles in a

subdivided population: a simulation study. Genetics 89:793–810 CAS  PubMed  PubMed Central  Google Scholar  * Slatkin M, Wiehe T (1998) Genetic hitch-hiking in a subdivided population. Genet

Res Camb 71:155–160 CAS  Google Scholar  * Stephanie AF, Hickey RJ, Vankat JL, Guttman SI, Schaefer RL (1992) Genetic structure after forest fragmentation: a landscape ecology perspective

on Acer saccharum. Can J Bot 70:1659–1668 Google Scholar  * Tanksley S, Zamir D, Rick CM (1981) Evidence for extensive overlap of sporophytic and gametophytic gene expression in

_Lycopersicon esculentum_. Science 213:453–455 CAS  PubMed  Google Scholar  * Weir BS (1996) Genetic data analysis II: methods for discrete population genetic data. Sinauer Associates, Inc

Publishers, Sunderland, MA Google Scholar  * White GM, Boshier DH, Powell W (2002) Increased pollen flow counteracts fragmentation in a tropical dry forest: an example from _Swietenia

humilis Zuccarini_. Proc Natl Acad Sci USA 99:2038–2042 * Whitehead MR, Lanfear R, Mitchell RJ, Karron JD (2018) Plant mating systems often vary widely among populations. Front Ecol

Evolution 6:38 Google Scholar  * Wright S (1946) Isolation by distance under diverse systems of mating. Genetics 31:39–59 CAS  PubMed  PubMed Central  Google Scholar  * Wright S (1969)

Evolution and the genetics of populations. The theory of gene frequencies, vol. 2. University Chicago Press, Chicago Google Scholar  * Zhang XX, Wang X, Hu Y, Zhou W, Chen XY, Hu XS (2019)

Advances in the study of population genetic diversity at plant species’ margins. Chin J Plant Ecol 43(5):383–395 Google Scholar  Download references ACKNOWLEDGEMENTS We appreciate editors

and three anonymous reviewers for very helpful comments that significantly improved the presentation of this article. We are grateful to Richard A. Ennos for proof reading this manuscript.

This work is supported by forestry reform and development fund of China central finance, forestry science and technology promotion and demonstration (2018-GDTK-08), and funding from the

South China Agricultural University (4400-K16013). AUTHOR INFORMATION AUTHORS AND AFFILIATIONS * College of Forestry and Landscape Architecture, South China Agricultural University,

Guangdong, 510642, China Xin-Xin Zhang, Xiang Cheng, Ling-Ling Li, Xi Wang, Wei Zhou, Xiao-Yang Chen & Xin-Sheng Hu * Guangdong Key Laboratory for Innovative Development and Utilization

of Forest Plant Germplasm, South China Agricultural University, Guangdong, 510642, China Xin-Xin Zhang, Xiang Cheng, Ling-Ling Li, Xi Wang, Wei Zhou, Xiao-Yang Chen & Xin-Sheng Hu

Authors * Xin-Xin Zhang View author publications You can also search for this author inPubMed Google Scholar * Xiang Cheng View author publications You can also search for this author

inPubMed Google Scholar * Ling-Ling Li View author publications You can also search for this author inPubMed Google Scholar * Xi Wang View author publications You can also search for this

author inPubMed Google Scholar * Wei Zhou View author publications You can also search for this author inPubMed Google Scholar * Xiao-Yang Chen View author publications You can also search

for this author inPubMed Google Scholar * Xin-Sheng Hu View author publications You can also search for this author inPubMed Google Scholar CORRESPONDING AUTHOR Correspondence to Xin-Sheng

Hu. ETHICS DECLARATIONS CONFLICT OF INTEREST The authors declare that they have no conflict of interest. ADDITIONAL INFORMATION PUBLISHER’S NOTE Springer Nature remains neutral with regard

to jurisdictional claims in published maps and institutional affiliations. Associate editor: Jinliang Wang SUPPLEMENTARY INFORMATION APPENDIX, SI FIGURES AND SCRIPTS IN C RIGHTS AND

PERMISSIONS Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Zhang, XX., Cheng, X., Li, LL. _et al._ The wave of gene advance under diverse systems of mating. _Heredity_ 125,

253–268 (2020). https://doi.org/10.1038/s41437-020-0333-9 Download citation * Received: 17 December 2019 * Revised: 09 June 2020 * Accepted: 09 June 2020 * Published: 30 June 2020 * Issue

Date: October 2020 * DOI: https://doi.org/10.1038/s41437-020-0333-9 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry, a

shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative