ABSTRACT 2D perovskites hold immense promise in optoelectronics due to their strongly bound electron-hole pairs (i.e., excitons). While exciton polaron from interplay between exciton and

lattice has been established in 2D lead-based perovskites, the exciton nature and behavior in the emerging 2D tin-based perovskites remains unclear. By combining spin-resolved ultrafast

spectroscopy and sophisticated theoretical calculations, we reveal 2D tin-based perovskites as genuine excitonic semiconductors with weak polaronic screening effect and persistent Coulomb

interaction, thanks to weak exciton-phonon coupling. We determine an excited state exciton binding energy of ~0.18 eV in _n_ = 2 tin iodide perovskites, nearly twice of that in lead

BEING VIEWED BY OTHERS MOMENTARILY TRAPPED EXCITON POLARON IN TWO-DIMENSIONAL LEAD HALIDE PEROVSKITES Article Open access 03 March 2021 REGULATION OF THE LUMINESCENCE MECHANISM OF

TWO-DIMENSIONAL TIN HALIDE PEROVSKITES Article Open access 10 January 2022 PEROVSKITE SEMICONDUCTORS FOR ROOM-TEMPERATURE EXCITON-POLARITONICS Article 01 July 2021 INTRODUCTION

Ruddlesden−Popper 2D layered metal halide perovskites, denoted as (LA)2(A)_n_−1B_n_X3_n_+1 where _n_ is inorganic layer thickness, have garnered considerable research interests in past few

years1,2,3,4,5,6,7,8,9,10. Segmenting metal halide inorganic layer by long-chain organic cations (LA+) not only establishes a robust barrier against water vapor erosion and ion migration,

but provides tunable structures, compositions, and properties, in particular, strongly bound electron-hole pairs (excitons) arising from dielectric and quantum confinement. Compared to the

conventional Pb counterparts which has been extensively investigated in past years, 2D tin halide perovskites with environmental hazard Pb substituted by biocompatible Sn has emerged very

recently and exhibited remarkable optoelectronic properties and technological prospects including stable lasing5, high-mobility field-effect transistors11,12 and efficient light-emitting

diodes with excellent external quantum efficiency1. The exciton nature and dynamics at excited state, rather than ground state, lies at the heart of optoelectronic materials and devices. In

2D lead halide perovskites, a general consensus has been reached that photoexcited excitons are dressed by lattice polarization and distortion, forming exciton polarons with screened

electron-hole interaction, due to the interaction between exciton and soft, polarizable lattice13,14,15,16,17,18,19. The formation of exciton polaron has strong impact on the intriguing

properties in 2D lead halide perovskites, including the rich exciton spectral structure, anomalous exciton spin relaxation, exciton dissociation and asymmetric light

emission17,18,19,20,21,22. Compared to the Pb counterparts, the excited state exciton nature and dynamics in the emerging 2D tin halide perovskites have been much less investigated, except

for a few recent photophysical comparison studies of 2D (_n_ = 1) lead and tin halide perovskite polycrystalline films23,24. Recent studies on 2D (_n_ = 1) and 3D tin halide perovskites by

steady-state spectroscopy measurements suggest a lower exciton binding energy in tin halide perovskites than that in their Pb counterparts7,24,25,26,27,28, which potentially would favor

exciton dissociation into free carriers and thus light-to-electricity conversion, rather than the reverse light emission process. However, this seems to contradict a very recent report of

highly efficient light-emitting diodes based on 2D tin halide perovskites1. The ultimate question lies in the exact nature and fate of exciton at excited state, especially how it is

regulated by exciton-lattice coupling in this family of materials, which remains a puzzle and hinders the design of 2D tin halide perovskites and devices. Here, we perform a comparison

photophysical study between _n_ = 2 (PEA)2(MA)Sn2I7 (PEA and MA stand for C6H5CH2CH2NH3+ and CH3NH3+, respectively) and its Pb counterpart (PEA)2(MA)Pb2I7 by combining state-of-the-art

time-resolved spectroscopy and theoretical simulation to reveal the excited state exciton properties in high quality 2D tin halide perovskite single crystals. Although (PEA)2MASn2I7 and the

Pb counterpart exhibit a same large exciton binding energy \({{E}_{{{\rm{b}}}}}^{0}\) ~ 0.2 eV from steady-state exciton Rydberg series measurement, spin-resolved transient absorption (TA)

measurements indicate (PEA)2(MA)Sn2I7 retain the strong bound excitons at excited state with an excited state exciton binding energy \({{E}_{{{\rm{b}}}}}^{*}\,\)~ 0.18 eV, in striking

contrast to the strongly screened excitons with \({{E}_{{{\rm{b}}}}}^{*}\) ~ 0.10 eV in Pb counterpart. An in-depth spectroscopy analysis reveals significantly weaker long- and short-range

exciton-phonon interactions in (PEA)2(MA)Sn2I7, resulting in alleviated excited state polaronic screening effect and robust exciton in 2D tin halide perovskites, which is further confirmed

with atomic level excited state stimulations. The robust excited state excitons in 2D tin halide perovskites manifest excitation-power-independent, high-efficiency and high color-purity

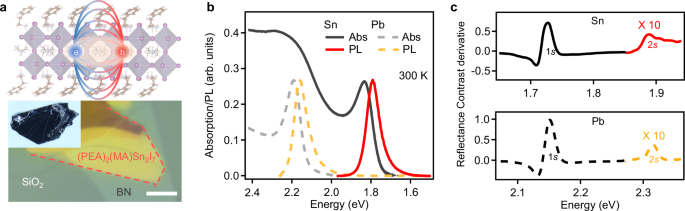
emission, lending them to light emission and lasing applications. RESULTS SAMPLE PREPARATION AND CHARACTERIZATIONS The high-quality (PEA)2(MA)Sn2I7 single crystals (Fig. 1a) were synthesized

under N2 environment by our recently developed method5. We mechanically exfoliated optically thin (PEA)2(MA)Sn2I7 flakes which were further sandwiched by thin-layer h-BN (Fig. 1a) through

van der Waals stacking to protect the 2D perovskite samples from degradation and obtain reliable results29. As a reference, we also prepared (PEA)2(MA)Pb2I7 sample with only different B site

cation using similar method for comparison. The thickness of exfoliated 2D perovskite flakes were characterized by atomical force microscopy which indicates 20–30 layers and thus bulk-like

2D perovskite samples (Supplementary Fig. 1). As shown in Fig. 1b, the absorption spectra of (PEA)2(MA)Sn2I7 and (PEA)2(MA)Pb2I7 exhibit a strong and sharp peak at 1.831 eV and 2.195 eV,

respectively, corresponding to their lowest energy exciton resonance. The photoluminescence (PL) spectra show a corresponding sharp peak with a slight Stokes shift (~0.04 eV). The

temperature-dependent PL spectra (Supplementary Fig. 2) and optical characterizations at 80 K (Supplementary Fig. 3) indicate negligible emission from other n or extrinsic edge states even

at cryogenic temperature and the absence of phase transition in 80–300 K6,30,31, confirming high phase purity and high crystal quality. We first determined the exciton binding energy

\({{E}_{{{\rm{b}}}}}^{0}\) by conventional steady-state exciton Rydberg series measurement, following previous success on 2D transition metal dichalcogenides and 2D lead halide

perovskites32,33. We measured the reflectance contrast spectra δ\(R\) of (PEA)2(MA)Sn2I7 and its Pb counterpart at 4 K and the reflectance contrast derivative \( (d\left({{\rm{\delta

}}}{{\rm{\it R}}}\right)/{dE})\) spectra exhibit clearly resolvable 1s and 2s excitonic peaks with similar energy spacing (\({\Delta }_{12}\) ~ 0.16 eV) (Fig. 1c). Thus, the exciton binding

energy \({{E}_{{{\rm{b}}}}}^{0}\) can be estimated from \({\Delta }_{12}\) by \({{{E}_{{{\rm{b}}}}}^{0}\approx 1.35\Delta }_{12}\)33. We determined a near identical

\({{E}_{{{\rm{b}}}}}^{0}\) for (PEA)2(MA)Pb2I7 and (PEA)2(MA)Sn2I7, \(0.22\pm 0.02\) eV and \(0.21\pm 0.02\) eV, respectively. The obtained \({{E}_{{{\rm{b}}}}}^{0}\) for (PEA)2(MA)Pb2I7

falls into the range of the exciton binding energy in similar materials reported previously (see Supplementary Table 1). We also note the exact value of \({{E}_{{{\rm{b}}}}}^{0}\) in 2D

perovskites are currently still largely dispersed and requires further experimental and theoretical study, which is beyond the scope of this study focusing on the excited state properties.

In the absence of phase transition, \({{E}_{{{\rm{b}}}}}^{0}\) can be regarded as a constant from 4 K to room temperature and is about ten times of thermal energy \({k}_{{{\rm{B}}}}T\)

(\({k}_{{{\rm{B}}}}\) is Boltzmann constant and \(T\) is temperature) at room temperature7,8. According to Saha-Langmuir equation34,35, at room temperature, more than 95% photoexcitation

remain as excitons in both (PEA)2(MA)Pb2I7 and (PEA)2(MA)Sn2I7 at excitation density of ~1011 cm−2 (see details in Supplementary Note 1). The result from steady-state optical measurements

does not necessarily reflect the excited state properties because of possible electronic and structural relaxation at excited state. We first resort to PL measurements to get a sense of the

excited state behavior in these two 2D metal halide perovskites. The PL quantum yields (QYs) of (PEA)2(MA)Sn2I7 and (PEA)2(MA)Pb2I7 were measured as a function of excitation power (see

details in Supplementary Note 2) and shown in Fig. 2a. Interestingly, PLQY of (PEA)2(MA)Sn2I7 remains a near constant of ~60% over three orders of magnitude of excitation power, while that

of the Pb counterpart increases continuously from ~1% to ~20% at same range. This result suggests pure exciton recombination with monomolecular-like recombination behavior in (PEA)2(MA)Sn2I7

where PLQY does not depends on photoexcitation density but the presence of free carrier recombination in the Pb counterpart with bimolecular-like recombination behavior where higher

photoexcitation power increases encounter probability of electron and hole carriers thus PLQY (Fig. 2b and Supplementary Note 3)32,36. The excited state recombination dynamics can be

directly obtained from time-resolved (TR) measurements. Importantly, TR-PL and TA exhibit near-identical kinetics for (PEA)2(MA)Sn2I7 but large deviation for (PEA)2(MA)Pb2I7 (Fig. 2c and

Supplementary Fig. 5), which again suggests dominant excitonic recombination process in (PEA)2(MA)Sn2I7 but substantial fraction of dissociated electron and hole recombination process in

(PEA)2(MA)Pb2I719. Key information about the excited state species is from examining the initial TR-PL intensity at \(t=0\) (denoted as \({{{\rm{TRPL}}}}_{0}\)), which is proportional to the

radiative recombination rate of the initial (~6 ps) photogenerated excited state species since TA kinetics shows no decay in the first 20 ps. Depending on the nature of emitting species,

the radiative recombination rate or \({{{\rm{TRPL}}}}_{0}\) is proportional to photoexcitation densities (\(\propto {n}_{0}\)) for exciton recombination with monomolecular-like behavior or

\({{\propto n}_{0}}^{2}\) for dissociated electron and hole recombination with bimolecular-like behavior (Fig. 2b)36. \({{{\rm{TRPL}}}}_{0}\) at 80 and 300 K for both materials are plotted

as a function of \({n}_{0}\) in Fig. 2d. A power law fitting (\({{{\rm{TRPL}}}}_{0}\propto {n}_{0}^{\beta }\)) of experimental results shows (PEA)2(MA)Sn2I7 increases linearly with a power

factor \(\beta\) of 1.06 and 1.04 at 300 and 80 K, respectively, indicating a robust exciton emission with negligible dissociation at 300 K. In contrast, the Pb counterpart exhibits a

similar linear behavior at 80 K (\(\beta\) = 1.06) but a super-linear relationship (\(\beta\) = 1.31) at 300 K, confirming high concentration of free electron/hole carriers by exciton

thermal dissociation at room-temperature. Similar _β_ values were obtained under near-resonant excitation conditions (Supplementary Fig. 6), demonstrating that the independence of _β_ on

excess energy under our experimental conditions. EXCITON SPIN RELAXATION Robust excitonic recombination in (PEA)2(MA)Sn2I7 and substantial free carrier recombination in its Pb counterpart

indicates their different electron-hole Coulomb interaction strength at excited state, which strongly contradict with their similar \({{E}_{{{\rm{b}}}}}^{0}\) of ~0.2 eV extracted from

steady state measurements, but suggest different polaronic screening effect at excited state considering their polar and dynamic lattice nature16,17,18,35. As demonstrated by our previous

study on 2D CsPbBr3, the excited state polaronic effect and the resulting screened exciton binding energy at excited state (denoted as \({{E}_{{{\rm{b}}}}}^{*}\)) can be inferred from

exciton spin relaxation dynamics35. In excitonic systems, the exciton spin relaxation is governed by excited state electron-hole exchange interaction (\(J\)) through Maialle-Silva-Sham (MSS)

mechanism35,37,38,39,40. Since \(J\) is proportional to \({{E}_{{{\rm{b}}}}}^{*}\), exciton spin relaxation rate \({k}_{{{\rm{s}}}}\) provides a facial way to quantify

\({{E}_{{{\rm{b}}}}}^{*}\) by $${k}_{{{\rm{s}}}} \approx \left\langle {{\varOmega }_{{{\rm{K}}}}}^{2}\right\rangle {\tau }_{{{\rm{p}}}}\propto {J}^{2}T{\tau }_{{{\rm{p}}}}\propto

({{{E}_{{{\rm{b}}}}}^{*}})^{2}T/\varGamma$$ (1) where \({\varOmega }_{{{\rm{K}}}}\) is momentum-dependent Larmor frequency under an effective magnetic field, _T_ denotes temperature and

\({\tau }_{{{\rm{p}}}}\) is momentum scattering time which is inversely proportional to PL linewidth \(\varGamma\) (see details in Supplementary Note 4)38,39. The MSS model has described the

_T_-dependent exciton spin relaxation in 2D transition metal dichalcogenides and quantum wells in a quantitative manner38,39,40,41. We performed spin-resolved femtosecond TA spectroscopy

measurements on the exciton spin relaxation dynamics in (PEA)2(MA)Sn2I7 at 80–300 K. As shown in Fig. 3a, spin-polarized excitons |+1> (|−1>) can be selectively excited by right (left)

circularly polarized pump pulse \({{{\rm{\sigma }}}}^{+}\) (\({{{\rm{\sigma }}}}^{-}\)) and probed by another circular polarized probe pulse with either same (SCP) or opposite (OCP)

circular polarization conditions (see Methods for details)35,42,43,44,45,46. Spin-resolved TA results of (PEA)2(MA)Sn2I7 at 1.93 eV near-resonant excitation under SCP and OCP conditions are

shown in Fig. 3b, with a few representative spectra at indicated delay times (0.4, 4, and 20 ps) in Fig. 3c for better view. The pump fluence was kept low of 3–8 μJ cm−2 to ensure TA signal

in linear regime. Generally speaking, TA signal in 2D semiconductors stems from combined band filling effect and Coulombic effect including band renormalization, biexciton effect, and

exciton screening35,42,43. While band filling and biexciton effect are sensitive to photoexcited exciton occupation in a specific spin state, other effects would apply to both spin states,

leading to TA spectral difference. At early time (0.4 ps) after photoexcitation, SCP spectra of (PEA)2(MA)Sn2I7 show a dominant photoinduced bleach (PB) feature at exciton resonance due to

band filling and band renormalization effect, and a photoinduced absorption (PA2) at higher energy side due to biexciton repulsion effect (interaction between pump-generated preexisted

exciton and probe-induced excitonic transition) from excitons with same spin. On the other hand, OCP spectra show a derivative line shape with PA1 feature around exciton resonance which can

be ascribed to spin-independent band renormalization effect35,42. Therefore, the difference on TA spectra between SCP and OCP (i.e. \({{\rm{\delta }}}{{\mbox{T}}}_{{\mbox{SCP}}}-{{\rm{\delta

}}}{{\mbox{T}}}_{{\mbox{OCP}}}\)) indicates the imbalance of exciton population in +1 and −1 spin states and the creation of spin polarization, which vanishes in ~20 ps (Fig. 3c). To

extract the exciton spin relaxation dynamics, we spectrally integrated SCP and OCP spectra at low energy side, where the difference between them is mainly ascribed to the band filling of

spin-polarized excitons. The degree of exciton spin polarization (Pol) is calculated by \({\mbox{Pol}}=({{\rm{\delta }}}{{\mbox{T}}}_{{\mbox{SCP}}}-{{\rm{\delta

}}}{{\mbox{T}}}_{{\mbox{OCP}}})/({{\rm{\delta }}}{{\mbox{T}}}_{{\mbox{SCP}}}+{{\rm{\delta }}}{{\mbox{T}}}_{{\mbox{OCP}}})\). As shown in Fig. 3d, the spin polarization shows a fast

relaxation with a lifetime of \(2.79\pm 0.06\) ps in (PEA)2(MA)Sn2I7. This lifetime is two orders of magnitude shorter than exciton population lifetime, indicating a pure spin relaxation

process. This exciton spin relaxation rate is 1–2 orders of magnitude faster that than in 3D bulk Sn-based materials, confirming the dominant electron-hole exchange interaction (MSS)

mechanism in 2D perovskites47. The spin relaxation rates are also found to depend on pump fluences linearly in (PEA)2(MA)Sn2I7 (Supplementary Fig. 7), which further proves the dominant MSS

spin relaxation mechanism rather than the Bir−Aronov−Pikus (BAP) or the D’yakonov-Perel (DP) mechanism35,40,48. In addition, we performed the same spin relaxation measurement with larger

(0.15 eV) or smaller (0.05 eV) excess energies (relative to bandgap) and found that the excess energy mostly affects the initial spin polarization degree and has little effect on the spin

relaxation dynamics (Supplementary Fig. 8), which is consistent with previous studies35,49. According to Eq. (1), information about excited state electron-hole interaction comes from

examining _T_-dependent exciton spin relaxation kinetics. As shown in Fig. 4a, b, interestingly, by increasing temperature from 80 K to 300 K, exciton spin relaxation is accelerated in

(PEA)2(MA)Sn2I7 but decelerated in the Pb counterpart. The _k_s by single exponential fitting on exciton spin relaxation kinetics are plotted (symbols) in Fig. 4c, d for (PEA)2(MA)Sn2I7 and

(PEA)2(MA)Pb2I7, respectively, showing interestingly opposite _T_-dependence. The _T_-dependent _k_s in excitonic system can be described by Eq. (1) and the modeled results assuming a

_T_-independent constant \({{E}_{{{\rm{b}}}}}^{*}\) are plotted in Fig. 4c, d (line) for (PEA)2(MA)Sn2I7 and the Pb counterpart, respectively. The modeled curve has been scaled to match

experimental value at 80 K where lattice is mostly frozen and excited state polaronic screening effect can be approximated to be negligible (i.e. \({{E}_{{{\rm{b}}}}}^{*}\) = 

\({{E}_{{{\rm{b}}}}}^{0}\))50. Interestingly, the measured _k_s for (PEA)2(MA)Sn2I7 shows similar trend as the modeled curve but diverges slightly at high temperature while the measured and

modeled _k_s for (PEA)2(MA)Pb2I7 show completely opposite trend. The negative deviation of experimental _k__s_ compared to modeled value assuming constant \({{E}_{{{\rm{b}}}}}^{*}\) = 

\({{E}_{{{\rm{b}}}}}^{0}\) indicates that \({{E}_{{{\rm{b}}}}}^{*}\) is temperature dependent and significantly screened at elevated temperature. The extent of deviation between experimental

and model values allows a quantitative calculation of \({{E}_{{{\rm{b}}}}}^{*}\) since \({k}_{{{\rm{s}}}}\propto {({{E}_{{{\rm{b}}}}}^{*})}^{2}\)18,35. For example, at room temperature,

modeled \({k}_{{{\rm{s}}}}\) of (PEA)2(MA)Pb2I7 is 4.54 times the measured value which yields an excited state exciton binding energy \({{E}_{{{\rm{b}}}}}^{*}\) of ~ 0.10 \(\pm\) 0.01 eV. A

similar analysis on (PEA)2(MA)Sn2I7 provides a \({{E}_{{{\rm{b}}}}}^{*}\) of ~ 0.18 \(\pm\) 0.02 eV, almost twice of that in Pb counterpart. EXCITON-PHONON INTERACTION The screened

\({{E}_{{{\rm{b}}}}}^{*}\) well explains different light emission behaviors in (PEA)2(MA)Sn2I7 and the Pb counterpart where robust excitonic behavior in the former but partial free carrier

behavior in latter have been observed, despite of near identical \({{E}_{{{\rm{b}}}}}^{0}\). Because of their polar and dynamic lattice, photoexcited excitons in 2D metal halide perovskites

can polarize lattice through exciton-phonon interaction, forming exciton polarons16,18,19,35. Depending on the nature and range of exciton-lattice interaction, the excited state polaronic

effect can arise from both long-range electrostatic polarization response (Fröhlich interaction) and short-range deformation potential (Holstein interaction)17,18,20. The long-range Fröhlich

coupling strength is proportional to the lattice polarization response and can be captured by _T_-dependent PL linewidth20,51, while the short-range Holstein interaction manifests itself as

a Urbach tail on absorption/PL spectra52,53,54,55. The PL spectra of (PEA)2(MA)Sn2I7 and its Pb counterpart at different temperatures (80–300 K) are shown in Fig. 5a, b, respectively, which

are broader and more asymmetrical with a prominent low energy tail (Urbach tail) at higher temperature. The full-width-at-half-maximum (FWHM) of PL spectra at different temperatures can be

described by a phenomenological equation31,50,51 $${{\rm{FWHM}}}={\varGamma }_{0}+\frac{{\gamma }_{{{\rm{LO}}}}}{\left[\exp \left({E}_{{{\rm{LO}}}}/{k}_{{{\rm{B}}}}T-1\right)\right]}$$ (2)

where the first term \({\varGamma }_{0}\) is _T_-independent and the second term is contributed by longitudinal optical (LO) phonon scattering with an effective phonon energy

\({E}_{{{\rm{LO}}}}\) and a coupling strength \({\gamma }_{{{\rm{LO}}}}\). By extracting and fitting the FWHM of PL spectra with Eq. (2) (Fig. 5c), we obtain a coupling strength \({\gamma

}_{{{\rm{LO}}}}\) of 0.072 ± 0.004 eV for (PEA)2(MA)Sn2I7, which is smaller than \({\gamma }_{{{\rm{LO}}}}\) of 0.095 ± 0.008 eV for its Pb counterpart. We also analyzed the Urbach tail of

PL to quantify the short-range Holstein exciton-phonon coupling strength. The absorption coefficient \(\alpha \left(E,T\right)\) of Urbach tail below optical band gap can be described by

refs. 52,53,54 $$\alpha \left(E,T\right)={\alpha }_{0}\exp \left(\frac{\sigma \left(T\right)\left(E-{E}_{0}\right)}{{k}_{{{\rm{B}}}}T}\right)$$ (3) where \({\alpha }_{0}\) and \({E}_{0}\)

are constants, \(\sigma \left(T\right)\) is a _T_-dependent steepness parameter which contains exciton-phonon coupling information. With van Roosbroeck-Schockley relation, the Urbach tail of

PL can be described by refs. 53,54 $${I}_{{{\rm{PL}}}}\left(E,T\right)\propto {E}^{2}\exp \left(\frac{\sigma \left(T\right)-1}{{k}_{{{\rm{B}}}}T}\left(E-{E}_{0}\right)\right)$$ (4)

Generally, \(\sigma \left(T\right)\) increases with _T_ and approaches a limit of steepness constant \({\sigma }_{0}\) at high temperature (e.g., 300 K) by $$\frac{\sigma

\left(T\right)}{{k}_{{{\rm{B}}}}T}=\frac{2{\sigma }_{0}}{{{\hslash }}{\omega }_{{{\rm{ph}}}}}\tanh \left(\frac{{{\hslash }}{\omega }_{{{\rm{ph}}}}}{2{k}_{{{\rm{B}}}}T}\right)$$ (5) where

\(\hslash {\omega }_{{{\rm{ph}}}}\) is the average energy of interaction phonon modes. Importantly, the steepness constant \({\sigma }_{0}\) is an inherent property for a certain material

and inversely proportional to the Holstein exciton-phonon coupling strength, i.e., smaller \({\sigma }_{0}\) indicates a stronger short-range exciton-phonon coupling. In high-quality

semiconductors with weak electron-phonon coupling strength, \({\sigma }_{0}\) from PL measurement (corresponding to excited-state configuration, denoted as \({{\sigma }_{0}}^{*}\)) and that

from absorption measurement (corresponding to ground-state configuration, \({{\sigma }_{0}}^{0}\)) have near same value54,55. We determined the steepness parameters \(\sigma \left(T\right)\)

for (PEA)2(MA)Sn2I7 and its Pb counterpart by fitting PL tails with Eq. (4) (Fig. 5a, b) and the obtained \(\sigma \left(T\right)\) are plotted in Fig. 5d as a function of temperature. For

(PEA)2(MA)Sn2I7, \(\sigma \left(T\right)\) increases with temperature and approaches a limit of \(1.58\pm 0.01\) (\({{\sigma }_{0}}^{*}\)). This value is smaller than that (2.28) in its 3D

counterpart54, which can be ascribed to the increased exciton-phonon coupling at reduced dimensionality. As a comparison, \(\sigma \left(T\right)\) of (PEA)2(MA)Pb2I7 initially increases

with temperature at cryogenic region (80–120 K) and then decreases monotonically to a plateau of \({{\sigma }_{0}}^{*} \sim 1.46\pm 0.01\) at 300 K, which is much smaller than that of

(PEA)2(MA)Sn2I7. \(\sigma \left(T\right)\) of (PEA)2(MA)Pb2I7 at low temperature (<120 K) where lattice is frozen and polaronic effect is negligible50 can be well fitted by Eq. (5)

(dashed line in Fig. 5d) and the deviation at high temperature indicates the excited state electronic and structural relaxation due to exciton-lattice coupling. As shown in Fig. 5d, the

measured \(\sigma \left(T\right)\) of (PEA)2(MA)Sn2I7 exhibits slight deviation from the fitted curve at high temperature, indicating the presence but rather weak excited state polaronic

effect in (PEA)2(MA)Sn2I7. In contrast, the measured \(\sigma \left(T\right)\) deviates significantly from the fitted curve at high temperatures for (PEA)2(MA)Pb2I7, revealing prominent

_T_-dependent excited state polaronic effect. To further confirm the fitted curve from low temperature \({{\sigma }_{0}}^{*}\) in (PEA)2(MA)Pb2I7, we also determined \({{\sigma }_{0}}^{0}\)

from absorption tail by PL excitation (PLE) measurement at 300 K (see details in Supplementary Note 5) and the obtained \({{\sigma }_{0}}^{0} \sim 1.58\pm\)0.02 (blue circle) falls on the

fitted curve. DISCUSSION The determined photophysical parameters for (PEA)2(MA)Sn2I7 and its Pb counterpart are summarized in Table 1. We also extracted the high-frequency dielectric

constant \({\varepsilon }_{\infty }\) along _c_ axis and exciton effective mass from first-principles calculation for discussions (see Supplementary Note 6). (PEA)2(MA)Sn2I7 and its Pb

counterpart have same crystal structure with only different B site cations, which results in near identical \({\varepsilon }_{\infty }\) and justifies similar \({{E}_{{{\rm{b}}}}}^{0}\) of

~0.21 eV for (PEA)2(MA)Sn2I7 and 0.22 eV for (PEA)2(MA)Pb2I7 by steady-state optical measurement56. However, in-depth PL analysis reveals very different long- and short-range exciton-lattice

coupling strength, as evidenced by \({\gamma }_{{{\rm{LO}}}}\) and \({{\sigma }_{0}}^{*}\). \({\gamma }_{{{\rm{LO}}}}\), which is proportional to the long-range polarization

response20,31,51, is much smaller in (PEA)2(MA)Sn2I7 than the Pb counterpart. Smaller \({\gamma }_{{{\rm{LO}}}}\) suggests less excited state structural relaxation in (PEA)2(MA)Sn2I7, i.e.,

less displacement of excited-state potential energy surface (PES) relative to ground-state PES21,57. Meanwhile, \({{\sigma }_{0}}^{*}\), inversely proportional to short-range deformation

potential, is much larger in (PEA)2(MA)Sn2I7 (1.58 ± 0.01) than its Pb counterpart (1.46 ± 0.01), which also suggests much less excited state structural deformation in the former. A more

intuitive way to understand \({{\sigma }_{0}}^{*}\) is to compare it with the critical value \({\sigma }_{{{\rm{c}}}}\) of 1.42 for 2D materials, which determines the relative energy

position and thus stability between band edge delocalized exciton and deformed Urbach exciton (UE)17,52,58. As \({\sigma }_{{{\rm{c}}}}\) is much smaller than \({{\sigma }_{0}}^{*}\) of

(PEA)2(MA)Sn2I7 but similar to that of the Pb counterpart, UE state lies much higher than the band edge state in (PEA)2(MA)Sn2I7 but close to that in (PEA)2(MA)Pb2I7 (Fig. 6a, b)17,52.

Therefore, compared to Pb counterpart with dynamic interconversion between band edge state and UE state, (PEA)2(MA)Sn2I7 retains its band edge exciton with much weaker excited state

polaronic effect (Fig. 6a). In the context of exciton polaron, electrons and holes with oppososing lattice deformation are mutually separated in real space. Specifically, the hole induces a

compression of bonds, while the electron causes an elongation of bonds in metal halides17,18,21. Hence, weaker excited state polaronic effect in (PEA)2(MA)Sn2I7 results in less polaronic

screening effect and larger binding energy \({{E}_{{{\rm{b}}}}}^{*}\), and vice versa for (PEA)2(MA)Pb2I7. With \({{E}_{{{\rm{b}}}}}^{*}\) of 0.18 eV for (PEA)2(MA)Sn2I7, Saha-Langmuir

equation (Supplementary Note 1) yields little exciton dissociation (7% at \({n}_{0}\) = 1011 cm-2) at room temperature, which well explains the exciton recombination behavior. On the other

hand, a \({{E}_{{{\rm{b}}}}}^{*}\) of 0.10 eV for (PEA)2(MA)Pb2I7 implies 35% exciton dissociation at \({n}_{0}\) = 1011 cm-2 and a power factor \(\beta\) of 1.35 (Supplementary Note 1),

which also agrees well with experimental value (1.31) from TRPL0 results. This result aligns with earlier observations of elevated free-carrier densities in 2D lead halide perovskites. The

measured densities surpass the values predicted by the Saha-Langmuir equation when using the exciton binding energy from the ground state34,59,60. This discrepancy highlights the significant

polaronic effect in the excited states of these materials. Meanwhile, Moser et al. have demonstrated exciton-exciton Auger heating contributes to the formation of long-lived free

carriers59, which might be the primary reason for the observation of non-negligible free carrier THz signals even at 4 K34. To substantiate the excited state polaronic effect and the

screening of electron-hole interactions at atomic level, we conducted theoretical atomistic simulations employing density functional theory (DFT) and time-dependent density functional theory

(TDDFT). These approaches allowed for the directly visualization of photo-induced structural and electronic relaxation at excited state61. The details of computational methods are provided

in Supplementary Note 6. Briefly speaking, the primitive cell of (PEA)2(MA)Sn2I7 or its Pb counterpart with experimental lattice parameters (Supplementary Table 2) was used to generate a 2 ×

 2 × 1 supercell. With Perdew-Burke-Ernzerhof functional, the ground-state configurations were optimized by the DFT approach, followed by relaxation of the lowest-energy excited-state

geometries through TDDFT approach. Given that the dielectric screening is weak along _c_ axis, the density of states (DOS) and photogenerated electron-hole distributions were calculated by

DFT and TDDFT, respectively, with optimally tuned and range-separated hybrid functional62. From the calculation, the iodine atoms in PbI2 layers displace at excited state, with the concave

and protruding iodine atoms moving towards straightening the Pb-I-Pb bonds (Fig. 6c). As a result, the distances between the concave (protruding) iodine atoms and the end -NH3 of organic

ligands, which are highlighted by red (green) lines in Fig. 6c, are increased (decreased). As distances guided by the red lines are 0.1–0.2 Å smaller than those guided by the green lines,

the hydrogen bonds are overall weakened under photoexcitation. This agrees with the partial DOS where the valence and conduction band are predominantly contributed by the 5p orbital of

iodine and the 5p (6p) orbital of Sn (Pb) cation (see details in Supplementary Note 6), respectively. Thereby, a fraction of electron densities transfers from iodine atoms to B site atoms

under photoexcitation, attenuating hydrogen bonding. We quantify the overall light-induced structural changes in (PEA)2(MA)Sn2I7 and its Pb counterpart by a set of inter-octahedron geometry

descriptors including length displacement \({L}_{{{\rm{d}}}}\) and angle deviation \({\theta }_{{{\rm{d}}}}\)63 $${L}_{{{\rm{d}}}}=\sum _{i=1}^{N}\left|{L}_{i}-L\right|$$ (6) $${\theta

}_{{{\rm{d}}}}=\sum _{i=1}^{N}\left|90-{\theta }_{i}\right|$$ (7) where \({L}_{i}\) is the distance between two adjacent B site atom (B-B), \({\theta }_{i}\) is the right angle of B-B-B and

_L_ denotes the average value of all \({L}_{i}\) at a-b plane (Fig. 6c). The \({L}_{{{\rm{d}}}}\) and \({\theta }_{{{\rm{d}}}}\) for (PEA)2(MA)Sn2I7 and the Pb counterpart at both

ground-state and relaxed excited-state configurations are calculated and plotted in Fig. 6d, e, respectively. Interestingly, both materials exhibit reductions of \({L}_{{{\rm{d}}}}\) and

\({\theta }_{{{\rm{d}}}}\) at excited-state relative to ground state, which is consistent with very recent experimental results showing photoexcitation-induced lattice straightening in lead

halide perovskites64. Importantly, (PEA)2(MA)Sn2I7 shows less change of \({L}_{{{\rm{d}}}}\) and \({\theta }_{{{\rm{d}}}}\) at excited state compared to the Pb counterpart. On the other

hand, the electronic structure change can be captured by the change of DOS in Fig. 6f, g. The conduction band edge at excited-state shifts down compared to ground-state in both materials and

the shift is less in (PEA)2(MA)Sn2I7 than the Pb counterpart. These calculations on ground state and excited state nuclear and electronic structures confirm less excited state relaxation in

(PEA)2(MA)Sn2I7. The excited state polaronic effect on the screening of electron-hole interaction can be scrutinized by calculating the distance between electron and hole,

\(\left|d\left(e\right)-d\left(h\right)\right|\). The effective positions of electron and hole along c direction can be defined as $$d=\frac{{\sum }_{i}z{\rho }_{i}\left(z\right)}{{\sum

}_{i}{\rho }_{i}\left(z\right)}$$ (8) where _z_ denotes the coordinate along c direction and the \({\rho }_{i}\left(z\right)\) represents the summed electron (hole) densities in a-b plane at

_z_ coordinate (Fig. 6h). As shown in Fig. 6i where we compare electron-hole separation at ground and excited state configurations, the distance between electron and hole are increased at

excited state in both 2D tin- and lead-halide perovskites and the separation is significantly smaller in (PEA)2(MA)Sn2I7. This electron-hole separation at excited state, together with

geometric and electronic structure above, directly confirms excited state polaronic effect screens electron-hole interaction in both materials, forming exciton polaron and the polaronic

screening effect is much weaker in (PEA)2(MA)Sn2I7 than in the Pb counterpart. To unveil the ultimate structural origin for different excited state polaronic effect in (PEA)2(MA)Sn2I7 and

the Pb counterpart, we calculated the orientation-dependent Young’s modulus in a-b plane, which reflects the material stiffness (see Supplementary Note 6 for details)65. Notably, Young’s

modulus along a and b axis in (PEA)2(MA)Sn2I7 is ~90% and ~65% larger than that in Pb counterpart. The much larger Young’s modulus in (PEA)2(MA)Sn2I7 indicates a more stiffened and less

deformable lattice, which can be attributed to the shorter Sn-I bond. Furthermore, the exciton effective mass of (PEA)2(MA)Sn2I7 is about half of that in the Pb counterpart. A larger

effective mass implies a narrower electronic structural bandwidth and stronger exciton-phonon coupling strength, which tends to drive polaron formation17,21,57. To preclude external factors,

we have performed additional TRPL0 (Supplementary Fig. 9) and temperature dependent spin relaxation (Supplementary Fig. 10) measurements and analysis on different sets of (PEA)2(MA)Sn2I7

flakes and its Pb counterparts and observed similar excited state recombination and spin relaxation behaviors as observed above. We have also compared 2D tin- and lead- halide perovskites

with different _n_ (_n_ = 1 and 3, Supplementary Figs. 11, 12, 13) and different long-chain ligands (BA+: butylammonium, 2T+: bithiophenylethylammonium and 3T+:

2-([2,2′:5′,2″-terthiophen]-5-yl)ethan-1-aminium, _n_ = 2) (Supplementary Fig. 14). For _n_ = 2 and _n_ = 3 perovskites, we have observed consistently excitonic behavior in tin halide

perovskites but partial free carrier behavior due to much weakened electron-hole interaction in Pb counterparts, indicating a fairly robust exciton in 2D tin halide perovskites rather than

their Pb counterparts. Interestingly, we note both _n_ = 1 tin- and lead- halide perovskites exhibit a linear TRPL0 dependence on photoexcitation density at room temperature, indicating

persistently bound electron-hole pair and strong excitonic effect in both _n_ = 1 perovskites at room temperature. This is reasonable since the _n_ = 1 inorganic layer is too thin to screen

electron-hole binding energy sufficiently to be comparable to _k_B_T_ at room temperature. As a contrast, previous studies on 2D and 3D tin halide perovskites have suggested a lower

\({E}_{{{\rm{b}}}}\) than their Pb counterparts7,24,25,26,27,28. In 3D tin halide perovskites, the more stiffened Sn-I lattice and lighter Sn element should lead to higher optical phonon

frequencies \({\upsilon }_{{{\rm{Sn}}}-{{\rm{I}}}}\) which can reach the exciton characteristic frequency \({\upsilon }_{{{\rm{b}}}}={E}_{{{\rm{b}}}}/{{\hslash }}\) and thus excitons in 3D

tin halide perovskites experience more effective screening by the ionic metal-halide lattice26,27,28. Although \({\upsilon }_{{{\rm{b}}}}\) increases dramatically and gets out of optical

phonon frequencies from 3D to 2D, the smaller exciton effective mass and band gap still imply a smaller exciton binding energy in 2D tin halide perovskites than the Pb counterparts7,24,25.

These results highlight excited state polaronic screening effect, rather than ground state properties, govern the photogenerated exciton nature and dynamics. In contrast to Pb counterpart

with free carrier characteristics, the robust exciton with weak exciton-phonon coupling defines 2D tin halide perovskites as genuine excitonic semiconductors, which greatly benefits their

optoelectronic applications, especially for light emission purposes. The robust excitonic effect facilitates the encounter and radiative recombination of photo- or electric- injected

electron and hole carriers and inhibits the reverse exciton thermal dissociation. This, together with higher carrier mobility11,12 and color purity due to weak exciton-phonon coupling, is

ideal for light emission and lasing applications. In conclusion, we have performed a combined spectroscopy and theoretical study on the excited state exciton nature and dynamics in

high-quality 2D tin halide perovskites by comparing to the Pb counterpart. Despite both exhibiting a same large \({{E}_{{{\rm{b}}}}}^{0}\) ~ 0.2 eV from steady state exciton Rydberg series

measurement, we reveal a much weaker long- and short-range exciton-phonon coupling in 2D tin halide perovskites than the Pb counterpart, resulting in weak excited state polaronic screening

effect and the persistence of robust excitons in 2D tin halide perovskites. The excited state exciton binding energy in _n_ = 2 2D tin halide perovskites has been determined to be ~0.18 eV,

about twice of that in the Pb counterpart with same structure. This finding challenges the conventional view of exciton behavior and highlights the critical role of excited state polaronic

effect on exciton nature and dynamics in these materials. The robust excitons at excited state in 2D tin halide perovskites exhibits excitation power-insensitive, high-efficiency and high

color-purity light emission, rendering them superior for light-emitting diodes and lasers than their Pb counterparts. METHODS PREPARATION OF PEROVSKITE SINGLE CRYSTALS All single crystals

were grown using the slow cooling method5. For (PEA)2(MA)Sn2I7, 0.17 mmol phenethylammonium iodide (PEAI, Greatcell Solar Ltd.), 0.4 mmol methylammonium iodide (MAI, Greatcell Solar Ltd.)

and 0.4 mmol SnI2 (Shanghai Macklin Biochemical Co., Ltd.) were added into 1 mL hydriodic acid (HI, 57wt.%, TCI) and 0.1 mL hypophosphorous acid (H3PO2, J&K Scientific). For

(PEA)2(MA)2Sn3I10, 0.17 mmol PEAI, 0.67 mmol MAI and 0.4 mmol SnI2 were added into 1 mL HI and 0.1 mL H3PO2. For (PEA)2(MA)Pb2I7, 0.43 mmol PEAI, 0.60 mmol MAI and 0.59 mmol PbI2 (TCI

(Shanghai) Development Co., Ltd.) were added into 0.9 mL HI and 0.1 mL H3PO2. For (PEA)2(MA)2Pb3I10, 0.15 mmol PEAI, 0.53 mmol MAI and 0.59 mmol PbI2 were added into 0.8 mL HI and 0.1 mL

H3PO2. As for (BA)2(MA)Sn2I7, (2T)2(MA)Sn2I7 and (3T)2(MA)Sn2I7 single crystals, they were synthesized using the previously reported recipes5. After heating and complete dissolution, they

were put into a muffle furnace for cooling down from 383 K to 293 K at a cooling rate of 1 °C/h, and finally high purity halide perovskite single crystals were obtained. It is worth noting

that all the processes, starting from the weighing of precursors and ending with the drying of single crystals, are conducted within N2 glove box. The CCDC numbers for (PEA)2(MA)Sn2I7 and

(PEA)2(MA)Pb2I7 are 2240837 and 2299252, respectively. 2D perovskites and h-BN (Onway Technology) thin flakes were mechanically exfoliated onto gel films (Gel-Pak PF-X4) from bulk single

crystals. Under an optical microscope, we fabricated BN/perovskites/BN heterostructures to preserve 2D perovskites from the damage caused by water, oxygen and vacuum by transferring the

exfoliated thin flakes from gel film to SiO2 substrate in sequence29. The whole processes are operated in N2 glovebox. STEADY STATE OPTICAL MEASUREMENTS We used a home-built microscope setup

integrated with liquid-nitrogen-cooled cryostat (custom-made STC-W30, LANHAI Science Instrument) for optical characterizations and the samples are consistently in controlled environment of

vacuum or N2. The TeslatronPT VTI cryostat (Oxford Instruments Inc., temperature range from 4 to 300 K) was utilized for reflection spectra at Ultra-low temperature (4 K) with a home-made

optical probe inserts with a 50X objective lens (MPlan 50X, Olympus, NA = 0.75) seated on the probe for the optical excitation and collection. A broadband halogen lamp (OSLR, Thorlabs, Inc.)

is used as the light source. In steady-state absorption measurement, the fundamental 1030 nm femtosecond-pulsed laser (CARBIDE, Light Conversion Ltd) was focused onto a YAG (Yttrium

Aluminum Garnet) crystal for generating a continuum white light. The steady-state transmission and reflection differential spectra were obtained by normalizing the transmitted

(\({{\rm{\delta }}}T=({T}_{{{\rm{sample}}}}-{T}_{{{\rm{sub}}}})/{T}_{{{\rm{sub}}}}\)) and reflected (\({{\rm{\delta }}}R=({R}_{{{\rm{sample}}}}-{R}_{{{\rm{sub}}}})/{R}_{{{\rm{sub}}}}\))

light from the sample on transparent SiO2 substrate to that from the bare substrate, respectively. And The collection and analysis of transmitted and reflected light were conducted by liquid

nitrogen cooled detectors (PyLon 100B, Princeton Instruments). The absorption coefficient of sample was calculated by

\(A=1-({T}_{{{\rm{sample}}}}+{R}_{{{\rm{sample}}}})/({T}_{{{\rm{sub}}}}+{R}_{{{\rm{sub}}}})\). In PL measurement, PL spectra were obtained with a 532 nm continuous-wave (CW) laser

excitation. A supercontinuum laser (SC-OEM, YSL Photonics) coupled with a monochromator was used as excitation light source for PL excitation measurements. TIME-RESOLVED PHOTOLUMINESCENCE

MEASUREMENTS The fundamental 1030 nm femtosecond-pulsed laser (pulse width ~200 fs and frequency of 100–1000 KHz, YactoFiber-FL-20, Yacto-Tech) was focused onto a barium metaborate (BBO)

crystal to generate a 515 nm excitation light for TRPL measurements. The TRPL decay kinetics were collected using a TCSPC module (SPC-130INX) and ultrafast detector (Hybrid PMT, HPM-100-07)

with an instrument response function ~30 ps. SPIN-RESOLVED TRANSIENT ABSORPTION SPECTROSCOPY For TA measurements, two 1030 nm femtosecond-pulsed lasers (~150 fs pulse duration, supplied by

CARBIDE, Light Conversion Ltd) were focused onto YAG (Yttrium Aluminum Garnet) crystals, producing a continuum white light. One served as the probe light, and the other was filtered to

selected central wavelength with narrow FWHM (i.e., 640–660 nm) and served as the pump light. To accurately regulate the time delay between the pump and probe beams, a high-precision

motorized delay stage from Newport was employed. Both beams were converged and focused by a microscope equipped with a 50X transmissive lens, resulting in spot size smaller than 2 μm. The

transmitted probe light, with the pump beam efficiently filtered out, was detected by liquid nitrogen cooled detectors (PyLon 100B, Princeton Instrument). The TA signal (\(\Delta T/T\)) was

derived by normalizing the spectral intensity from the pumped to the unpumped state, yielding \({{\rm{\delta }}}T=\Delta T/T=({T}_{{{\rm{pump}}}\; {{\rm{on}}}}-{T}_{{{\rm{pump}}}\;

{{\rm{off}}}})/{T}_{{{\rm{pump}}}\; {{\rm{off}}}}\). For the spin-resolved transient absorption measurement, circularly polarized lights were generated by passing the linear polarized pump

and probe beams through a quarter wave plate (1/4_λ_). By manipulating the pump beam’s polarization direction using a half wave plate, either same (SCP) or opposite (OCP) circular

polarization conditions could be achieved relative to the probe beam. Throughout all optical measurements, the laser beam was focused to a spot size of less than 2 μm. DATA AVAILABILITY All

data to evaluate the conclusions are present in the manuscript and the Supplementary Material. Raw data are available from the corresponding authors on request. REFERENCES * Han, D. et al.

Tautomeric mixture coordination enables efficient lead-free perovskite LEDs. _Nature_ 622, 493–498 (2023). Article  ADS  CAS  PubMed  Google Scholar  * Morteza Najarian, A. et al. Homomeric

chains of intermolecular bonds scaffold octahedral germanium perovskites. _Nature_ 620, 328–335 (2023). Article  ADS  CAS  PubMed  Google Scholar  * Park, J. Y. et al. Thickness control of

organic semiconductor-incorporated perovskites. _Nat. Chem._ 15, 1745–1753 (2023). Article  CAS  PubMed  Google Scholar  * Lei, Y. et al. Perovskite superlattices with efficient carrier

dynamics. _Nature_ 608, 317–323 (2022). Article  ADS  CAS  PubMed  Google Scholar  * Li, Y. et al. Phase-pure 2D tin halide perovskite thin flakes for stable lasing. _Sci. Adv._ 9, eadh0517

(2023). Article  ADS  CAS  PubMed  Google Scholar  * Gu, H. et al. Phase-pure two-dimensional layered perovskite thin films. _Nat. Rev. Mater._ 8, 533–551 (2023). Article  ADS  CAS  Google

Scholar  * Hansen, K. R. et al. Mechanistic origins of excitonic properties in 2D perovskites: Implications for exciton engineering. _Matter_ 6, 3463–3482 (2023). Article  CAS  Google

Scholar  * Passarelli, J. V. et al. Tunable exciton binding energy in 2D hybrid layered perovskites through donor-acceptor interactions within the organic layer. _Nat. Chem._ 12, 672–682

(2020). Article  CAS  PubMed  Google Scholar  * Metcalf, I. et al. Synergy of 3D and 2D perovskites for durable, efficient solar cells and beyond. _Chem. Rev._ 123, 9565–9652 (2023). Article

  CAS  PubMed  Google Scholar  * Mihalyi-Koch, W. et al. Tuning structure and excitonic properties of 2D Ruddlesden–Popper germanium, tin, and lead iodide perovskites via interplay between

cations. _J. Am. Chem. Soc._ 145, 28111–28123 (2023). Article  CAS  PubMed  Google Scholar  * Liu, A. et al. High-performance metal halide perovskite transistors. _Nat. Electron._ 6, 559–571

(2023). Article  CAS  Google Scholar  * Liang, A. et al. Ligand-driven grain engineering of high mobility two-dimensional perovskite thin-film transistors. _J. Am. Chem. Soc._ 143,

15215–15223 (2021). Article  CAS  PubMed  Google Scholar  * Quan, L. N. et al. Vibrational relaxation dynamics in layered perovskite quantum wells. _Proc. Natl Acad. Sci. USA_ 118,

e2104425118 (2021). Article  CAS  PubMed  PubMed Central  Google Scholar  * Zhang, H. et al. Ultrafast relaxation of lattice distortion in two-dimensional perovskites. _Nat. Phys._ 19,

545–550 (2023). Article  CAS  Google Scholar  * Thouin, F. et al. Phonon coherences reveal the polaronic character of excitons in two-dimensional lead halide perovskites. _Nat. Mater._ 18,

349–356 (2019). Article  ADS  CAS  PubMed  Google Scholar  * Sun, Q. et al. Ultrafast and high-yield polaronic exciton dissociation in two-dimensional perovskites. _J. Am. Chem. Soc._ 143,

19128–19136 (2021). Article  CAS  PubMed  Google Scholar  * Srimath Kandada, A. R. & Silva, C. Exciton polarons in two-dimensional hybrid metal-halide perovskites. _J. Phys. Chem. Lett._

11, 3173–3184 (2020). Article  CAS  PubMed  Google Scholar  * Tao, W., Zhang, Y. & Zhu, H. Dynamic exciton polaron in two-dimensional lead halide perovskites and implications for

optoelectronic applications. _Acc. Chem. Res._ 55, 345–353 (2022). Article  CAS  PubMed  Google Scholar  * Simbula, A. et al. Exciton dissociation in 2D layered metal-halide perovskites.

_Nat. Commun._ 14, 4125 (2023). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Buizza, L. R. V. & Herz, L. M. Polarons and charge localization in metal-halide

semiconductors for photovoltaic and light-emitting devices. _Adv. Mater._ 33, e2007057 (2021). Article  PubMed  Google Scholar  * Fu, J., Ramesh, S., Melvin Lim, J. W. & Sum, T. C.

Carriers, quasi-particles, and collective excitations in halide perovskites. _Chem. Rev._ 123, 8154–8231 (2023). Article  CAS  PubMed  PubMed Central  Google Scholar  * Neutzner, S. et al.

Exciton-polaron spectral structures in two-dimensional hybrid lead-halide perovskites. _Phys. Rev. Mater._ 2, 064605 (2018). Article  CAS  Google Scholar  * Kahmann, S. et al. Photophysics

of two‐dimensional perovskites—learning from metal halide substitution. _Adv. Funct. Mater_. 31, 2103778 (2021). * Hansen, K. R. et al. Low exciton binding energies and localized

exciton–polaron states in 2D tin halide perovskites. _Adv. Opt. Mater._ 10, 2102698 (2022). Article  CAS  Google Scholar  * Dyksik, M. et al. Broad tunability of carrier effective masses in

two-dimensional halide perovskites. _ACS Energy Lett._ 5, 3609–3616 (2020). Article  CAS  Google Scholar  * Milot, R. L. et al. The effects of doping density and temperature on the

optoelectronic properties of formamidinium tin triiodide thin films. _Adv. Mater._ 30, 1804506 (2018). Article  Google Scholar  * Umari, P., Mosconi, E. & De Angelis, F. Infrared

dielectric screening determines the low exciton binding energy of metal-halide perovskites. _J. Phys. Chem. Lett._ 9, 620–627 (2018). Article  CAS  PubMed  Google Scholar  * Herz, L. M. How

lattice dynamics moderate the electronic properties of metal-halide perovskites. _J. Phys. Chem. Lett._ 9, 6853–6863 (2018). Article  CAS  PubMed  Google Scholar  * Zhou, H. et al.

Spatiotemporally coupled electron-hole dynamics in two dimensional heterostructures. _Nano Lett._ 22, 2547–2553 (2022). Article  ADS  CAS  PubMed  Google Scholar  * Zhang, T. et al.

Regulation of the luminescence mechanism of two-dimensional tin halide perovskites. _Nat. Commun._ 13, 60 (2022). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Wright, A. D.

et al. Electron–phonon coupling in hybrid lead halide perovskites. _Nat. Commun._ 7, 11755 (2016). Article  ADS  Google Scholar  * Chernikov, A. et al. Exciton binding energy and

nonhydrogenic Rydberg series in monolayer WS2. _Phys. Rev. Lett._ 113, 076802 (2014). Article  ADS  CAS  PubMed  Google Scholar  * Blancon, J. C. et al. Scaling law for excitons in 2D

perovskite quantum wells. _Nat. Commun._ 9, 2254 (2018). Article  ADS  PubMed  PubMed Central  Google Scholar  * Motti, S. G. et al. Exciton formation dynamics and band‐like free

charge‐carrier transport in 2D metal halide perovskite semiconductors. _Adv. Funct. Mater._ 33, 2300363 (2023). Article  CAS  Google Scholar  * Tao, W., Zhou, Q. & Zhu, H. Dynamic

polaronic screening for anomalous exciton spin relaxation in two-dimensional lead halide perovskites. _Sci. Adv._ 6, eabb7132 (2020). Article  ADS  PubMed  PubMed Central  Google Scholar  *

Xing, G. et al. Transcending the slow bimolecular recombination in lead-halide perovskites for electroluminescence. _Nat. Commun._ 8, 14558 (2017). Article  ADS  CAS  PubMed  PubMed Central

  Google Scholar  * Konabe, S. Screening effects due to carrier doping on valley relaxation in transition metal dichalcogenide monolayers. _Appl. Phys. Lett._ 109, 073104 (2016). Article 

ADS  Google Scholar  * Maialle, M. Z., de Andrada e Silva, E. A. & Sham, L. J. Exciton spin dynamics in quantum wells. _Phys. Rev. B_ 47, 15776–15788 (1993). Article  ADS  CAS  Google

Scholar  * Miyauchi, Y. et al. Evidence for line width and carrier screening effects on excitonic valley relaxation in 2D semiconductors. _Nat. Commun._ 9, 2598 (2018). Article  ADS  PubMed

  PubMed Central  Google Scholar  * Mahmood, F., Alpichshev, Z., Lee, Y. H., Kong, J. & Gedik, N. Observation of exciton-exciton interaction mediated valley depolarization in monolayer

MoSe2. _Nano Lett._ 18, 223–228 (2018). Article  ADS  CAS  PubMed  Google Scholar  * Zhu, C. R. et al. Exciton valley dynamics probed by Kerr rotation in WSe2 monolayers. _Phys. Rev. B_ 90,

161302(R) (2014). Article  ADS  Google Scholar  * Giovanni, D. et al. Coherent spin and quasiparticle dynamics in solution-processed layered 2D lead halide perovskites. _Adv. Sci._ 5,

1800664 (2018). Article  Google Scholar  * Zhou, H., Chen, Y. & Zhu, H. Deciphering asymmetric charge transfer at transition metal dichalcogenide-graphene interface by helicity-resolved

ultrafast spectroscopy. _Sci. Adv._ 7, eabg2999 (2021). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Chen, X. et al. Tuning spin-polarized lifetime in two-dimensional

metal-halide perovskite through exciton binding energy. _J. Am. Chem. Soc._ 143, 19438–19445 (2021). Article  CAS  PubMed  Google Scholar  * Giovanni, D. et al. Highly spin-polarized carrier

dynamics and ultralarge photoinduced magnetization in CH3NH3PbI3 perovskite thin films. _Nano Lett._ 15, 1553–1558 (2015). Article  ADS  CAS  PubMed  Google Scholar  * Bourelle, S. A. et

al. How exciton interactions control spin-depolarization in layered hybrid perovskites. _Nano Lett._ 20, 5678–5685 (2020). Article  ADS  CAS  PubMed  Google Scholar  * Liang, W. et al.

Efficient optical orientation and slow spin relaxation in lead-free CsSnBr3 perovskite nanocrystals. _ACS Energy Lett._ 6, 1670–1676 (2021). Article  CAS  Google Scholar  * Huang, Y. et al.

Tuning spin-polarized lifetime at high carrier density through deformation potential in Dion-Jacobson-phase perovskites. _J. Am. Chem. Soc._ 146, 12225–12232 (2024). Article  CAS  PubMed 

Google Scholar  * Lagarde, D. et al. Carrier and polarization dynamics in monolayer MoS2. _Phys. Rev. Lett._ 112, 047401 (2014). Article  ADS  CAS  PubMed  Google Scholar  * Guo, Y. et al.

Dynamic emission Stokes shift and liquid-like dielectric solvation of band edge carriers in lead-halide perovskites. _Nat. Commun._ 10, 1175 (2019). Article  ADS  PubMed  PubMed Central 

Google Scholar  * Rudin, S., Reinecke, T. & Segall, B. Temperature-dependent exciton linewidths in semiconductors. _Phys. Rev. B_ 42, 11218–11231 (1991). Article  ADS  Google Scholar  *

Tao, W., Zhang, C., Zhou, Q., Zhao, Y. & Zhu, H. Momentarily trapped exciton polaron in two-dimensional lead halide perovskites. _Nat. Commun._ 12, 1400 (2021). Article  ADS  CAS  PubMed

  PubMed Central  Google Scholar  * Huang, X. et al. Understanding electron-phonon interactions in 3D lead halide perovskites from the stereochemical expression of 6s(2) lone pairs. _J. Am.

Chem. Soc._ 144, 12247–12260 (2022). Article  CAS  PubMed  Google Scholar  * Handa, T., Aharen, T., Wakamiya, A. & Kanemitsu, Y. Radiative recombination and electron-phonon coupling in

lead-free CH3NH3SnI3 perovskite thin films. _Phys. Rev. Mater._ 2, 075402 (2018). Article  CAS  Google Scholar  * Ledinsky, M. et al. Temperature dependence of the Urbach energy in lead

iodide perovskites. _J. Phys. Chem. Lett._ 10, 1368–1373 (2019). Article  CAS  PubMed  Google Scholar  * Filip, M. R., Qiu, D. Y., Del Ben, M. & Neaton, J. B. Screening of excitons by

organic cations in quasi-two-dimensional organic-inorganic lead-halide perovskites. _Nano Lett._ 22, 4870–4878 (2022). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Iaru, C.

M. et al. Frohlich interaction dominated by a single phonon mode in CsPbBr(3). _Nat. Commun._ 12, 5844 (2021). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Schreiber, M.

& Toyozawa, Y. Numerical experiments on the absorption lineshape of the exciton under lattice vibrations. III. The Urbach rule. _J. Phys. Soc. Jpn._ 51, 1544–1550 (1982). Article  ADS 

CAS  Google Scholar  * Burgos-Caminal, A., Socie, E., Bouduban, M. E. F. & Moser, J.-E. Exciton and carrier dynamics in two-dimensional perovskites. _J. Phys. Chem. Lett._ 11, 7692–7701

(2020). Article  CAS  PubMed  Google Scholar  * Balogun, F. H. et al. Untangling free carrier and exciton dynamics in layered hybrid perovskites using ultrafast optical and terahertz

spectroscopy. _Mater. Res. Express_ 11, 025503 (2024). * Zhang, X., Lu, G., Baer, R., Rabani, E. & Neuhauser, D. Linear-response time-dependent density functional theory with stochastic

range-separated hybrids. _J. Chem. Theory Comput._ 16, 1064–1072 (2020). Article  CAS  PubMed  Google Scholar  * Refaely-Abramson, S., Jain, M., Sharifzadeh, S., Neaton, J. B. & Kronik,

L. Solid-state optical absorption from optimally tuned time-dependent range-separated hybrid density functional theory. _Phys. Rev. B_ 92, 081204(R) (2015). Article  ADS  Google Scholar  *

Han, X. B., Jing, C. Q., Zu, H. Y. & Zhang, W. Structural descriptors to correlate Pb ion displacement and broadband emission in 2D halide perovskites. _J. Am. Chem. Soc._ 144,

18595–18606 (2022). Article  CAS  PubMed  Google Scholar  * Yazdani, N. et al. Coupling to octahedral tilts in halide perovskite nanocrystals induces phonon-mediated attractive interactions

between excitons. _Nat. Phys._ 20, 47–53 (2024). Article  CAS  PubMed  Google Scholar  * Wang, V. & Geng, W. T. Lattice defects and the mechanical anisotropy of borophene. _J. Phys.

Chem. C._ 121, 10224–10232 (2017). Article  CAS  Google Scholar  Download references ACKNOWLEDGEMENTS We thank Zhihao Gong and Hua Wang at ZJU-Hangzhou Global Scientific and Technological

Innovation Center for help with theoretical studies. H. Zhu thanks the financial support from the National Natural Science Foundation of China (22273084, H.Z.) and Department of Science and

Technology of Zhejiang Province (2024C01191, H.Z.). G.Nan acknowledges the National Natural Science Foundation of China (22273088, G.N.), Hefei National Research Center for Physical Sciences

at the Microscale (KF2021004, G.N.) and Scientific Research Fund of Zhejiang Provincial Education Department (Y202250336, G.N.). H. Zhou thanks the financial support from the China

Postdoctoral Science Foundation (Grant No.2022M722727, H.Z.). This study is supported by the open fund of the state key laboratory of molecular reaction dynamics in DICP, CAS. AUTHOR

INFORMATION Author notes * These authors contributed equally: Hongzhi Zhou, Qingjie Feng. AUTHORS AND AFFILIATIONS * Department of Chemistry, State Key Laboratory of Extreme Photonics and

Instrumentation, Zhejiang Key Laboratory of Excited State Energy Conversion and Storage, Zhejiang University, Hangzhou, China Hongzhi Zhou, Cheng Sun, Weijian Tao & Haiming Zhu *

Department of Physics, Zhejiang Normal University, Jinhua, Zhejiang, China Qingjie Feng & Guangjun Nan * ZJU-Hangzhou Global Scientific and Technological Innovation Center, Hangzhou,

Zhejiang, China Cheng Sun & Haiming Zhu * Research Center for Industries of the Future and School of Engineering, Westlake University, Hangzhou, China Yahui Li & Enzheng Shi * State

Key Laboratory of Extreme Photonics and Instrumentation, College of Optical Science and Engineering, Zhejiang University, Hangzhou, China Wei Tang & Linjun Li Authors * Hongzhi Zhou View

author publications You can also search for this author inPubMed Google Scholar * Qingjie Feng View author publications You can also search for this author inPubMed Google Scholar * Cheng

Sun View author publications You can also search for this author inPubMed Google Scholar * Yahui Li View author publications You can also search for this author inPubMed Google Scholar *

Weijian Tao View author publications You can also search for this author inPubMed Google Scholar * Wei Tang View author publications You can also search for this author inPubMed Google

Scholar * Linjun Li View author publications You can also search for this author inPubMed Google Scholar * Enzheng Shi View author publications You can also search for this author inPubMed 

Google Scholar * Guangjun Nan View author publications You can also search for this author inPubMed Google Scholar * Haiming Zhu View author publications You can also search for this author

inPubMed Google Scholar CONTRIBUTIONS H.Zhou and H.Zhu. conceived the project. H.Zhou conducted steady-state PL, TRPL and TA measurements. Q.Feng and G.Nan conducted the theoretical

calculations. Y.L. and E.S. synthesized single-crystal samples. W.Tang and L.Li helped collect the reflectance spectra at 4 K. C.Sun helped collect the PLQY. W.Tao participated in data

analysis. H.Zhou, H.Zhu, Q.Feng, G.Nan wrote the manuscript. All authors read and revised the manuscript. CORRESPONDING AUTHORS Correspondence to Guangjun Nan or Haiming Zhu. ETHICS

DECLARATIONS COMPETING INTERESTS The authors declare no competing interests. PEER REVIEW PEER REVIEW INFORMATION _Nature Communications_ thanks Kameron Hansen, Michele Saba and the other

anonymous reviewer(s) for their contribution to the peer review of this work. A peer review file is available. ADDITIONAL INFORMATION PUBLISHER’S NOTE Springer Nature remains neutral with

regard to jurisdictional claims in published maps and institutional affiliations. SUPPLEMENTARY INFORMATION SUPPLEMENTARY INFORMATION PEER REVIEW FILE RIGHTS AND PERMISSIONS OPEN ACCESS This

article is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License, which permits any non-commercial use, sharing, distribution and reproduction

in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if you modified the

licensed material. You do not have permission under this licence to share adapted material derived from this article or parts of it. The images or other third party material in this article

are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and

your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this

licence, visit http://creativecommons.org/licenses/by-nc-nd/4.0/. Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Zhou, H., Feng, Q., Sun, C. _et al._ Robust excitonic light

emission in 2D tin halide perovskites by weak excited state polaronic effect. _Nat Commun_ 15, 8541 (2024). https://doi.org/10.1038/s41467-024-52952-9 Download citation * Received: 05

February 2024 * Accepted: 26 September 2024 * Published: 02 October 2024 * DOI: https://doi.org/10.1038/s41467-024-52952-9 SHARE THIS ARTICLE Anyone you share the following link with will be

able to read this content: Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing

initiative