ABSTRACT Networked entanglement is an essential component for a plethora of quantum computation and communication protocols. Direct transmission of quantum signals over long distances is

prevented by fibre attenuation and the no-cloning theorem, motivating the development of quantum repeaters, designed to purify entanglement, extending its range. Quantum repeaters have been

demonstrated over short distances, but error-corrected, global repeater networks with high bandwidth require new technology. Here we show that error corrected quantum memories installed in

cargo containers and carried by ship can provide a exible connection between local networks, enabling low-latency, high-fidelity quantum communication across global distances at higher

worldwide Quantum Internet. SIMILAR CONTENT BEING VIEWED BY OTHERS CONTINUOUS ENTANGLEMENT DISTRIBUTION OVER A TRANSNATIONAL 248 KM FIBER LINK Article Open access 17 October 2022 HYBRID

ENTANGLEMENT AND BIT-FLIP ERROR CORRECTION IN A SCALABLE QUANTUM NETWORK NODE Article Open access 03 April 2025 FLEXIBLE ENTANGLEMENT-DISTRIBUTION NETWORK WITH AN ALGAAS CHIP FOR SECURE

COMMUNICATIONS Article Open access 23 July 2021 INTRODUCTION Quantum communication will strengthen the security of cryptographic systems and decision-making algorithms1,2,3,4, support secure

client-server quantum computation5, and improve the sensitivity of scientific instruments6,7,8. An effective global quantum network is ultimately required to support such

applications9,10,11 and significant research has been conducted to realise such networks12,13,14,15. The most common method is the transmission of encoded optical signals along traditional

fibre connections between more localised networks. Photons are traditionally proposed for the establishment of quantum entanglement between stationary quantum systems over moderate

distances. Over longer distances, entanglement purification and entanglement swapping connecting a path comprised of shorter links will mitigate the exponential attenuation loss, the

no-cloning theorem16 and effects of imperfect devices12. The repeat-until-success nature of these techniques allows the rate of entanglement generation to decrease polynomially with

increasing distance, with the fidelity of entanglement limited by the accuracy of the quantum gates operating in the repeaters. While experimental demonstration of short-range quantum

communications has been effective17,18, long range repeater networks require the incorporation of fault-tolerant error correction methods, and numerous designs have been

proposed13,14,15,19,20,21,22. These designs do not offer bandwidth higher than about a MHz and require dense repeater arrays. A global network constructed in this way would require

high-power, low-temperature quantum devices with active control deployed in very hostile environments. While the deployment of satellite technology may mitigate this problem23, a fully error

corrected, global system has still not been developed. Consequently, no known technology meets the stringent requirements for global deployment. In this work we present an alternative

method that could be used to augment networks based on traditional repeaters and satellite technology. This approach can possibly solve crucial issues related to a global network in a way

that remains compatible with other well known networking schemes. Despite the capabilities of modern classical networks, it is still routine to transfer classical information stored in

removable media, an approach known as _sneakernet_. Here we adapt this approach to quantum information, introducing a new network mechanism for the establishment of quantum entanglement over

long distances based on the transport of error-corrected quantum memories24. Long-distance information transport involves significant latency, but establishing entanglement involves no

exchange of users’ data, meaning that this latency is irrelevant to network users. Indeed the fact that entanglement distribution can occur without the exchange of classical information

leads immediately to Peres’ well-known ‘paradox’ of delayed-choice entanglement swapping25,26. The fact that classical information need not be exchanged to distribute entanglement means that

quantum networks can be effectively zero latency, although of course any protocol that uses the distributed entanglement, for example, for quantum teleportation27, will be limited by the

classical one-way information transfer speed. Quantum memories may be transported to locations where entanglement is required or to intermediate locations to facilitate entanglement swapping

between traditional quantum repeater networks, enabling a complete network structure without the full deployment of physical links. Because transoceanic communication presents a particular

challenge for quantum networking, we focus on the establishment of entanglement by ship. This approach of creating a quantum sneakernet through physical transport of actively error corrected

quantum memories will require the fabrication of more physical qubits in the overall network, but has the possibility of significantly mitigating issues related to deployment of a quantum

network. The most effective quantum repeater designs for entanglement distribution (so called 3rd generation repeaters) employ full error correction protocols and consequently require close

separation (within of order 10 km) due to the need for low loss connections13,15. Each repeater unit is effectively a mini-quantum computer, requiring infrastructure technologies such as

cryogenic cooling and vacuum systems, extensive classical control (for both qubit manipulations and error-correction/network operations) and non-trivial power requirements. The deployment of

such devices, every 10 km, across an ocean constitutes an enormous engineering challenge. Conversely, a sneakernet approach allows us to convert this significant hurdle in deployment to the

challenge of making mini-quantum computers amenable to physical transport. This allows us to design memory units that can be serviced (or replaced) at regular intervals with minimal impact

on the overall network or incremental upgrades to the entire system as technology becomes denser and/or cheaper. This sneakernet method of quantum networking provides an alternate pathway

that could augment traditional repeater and satellite based systems where the issue of network deployment and servicing in hostile environments becomes extremely hard or where entanglement

nodes are required on mobile platforms not accessible by a traditional repeater node. Our approach requires quantum memories with an effective coherence time of months, sufficient for

transport along any traditional shipping channel. Because an error-corrected quantum memory is based on the same system architecture as a large-scale quantum computer, technology currently

in development will satisfy our needs28,29,30. In particular, implementations of qubits based on several physical systems—including superconducting circuits and trapped ions—are nearing the

accuracy threshold required for topological error correction to become effective31. Once this threshold is exceeded, it will be possible to arbitrarily lengthen the effective coherence time

of an error-corrected quantum memory with a poly-logarithmic qubit overhead. However, this new mechanism also requires quantum memories that are compatible with storage and transport in a

shipping container, including a stable power source, ultra-high vacuum or refrigeration systems to maintain appropriate operating conditions, and classical-control infrastructure to perform

error correction and decoding32. As yet, there are no implementations designed with this degree of portability in mind. However, keeping in mind these engineering constraints, we will, for

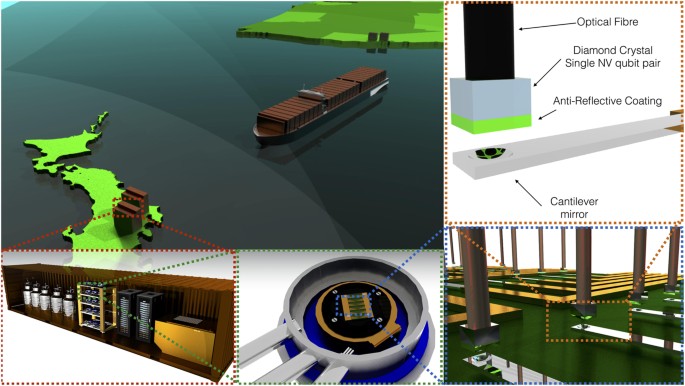
concreteness, consider a potential candidate system: an implementation based on negatively charged nitrogen vacancy (NV−) centres in diamond, which may be integrated in dense arrays and are

optically accessible at a temperature of 4 K30,33. The active nature of the quantum memory is designed to correct traditional errors arising from environmental decoherence and imperfect

control. However, mobile quantum memories may be subject to additional errors arising from physical movement. These additional errors can arise from changes in, for example, the local

magnetic field of the earth or from motional instability of the actual qubits. Most physical systems currently considered for large-scale quantum memories are immune from motional

instability ether because they are etched circuits (superconductors, linear optics) or because they consist of atoms locked into a physical lattice (NV-diamond, silicon). Changes in global

fields are not expected to cause significant problems for physical transport of these devices because global fields will vary on time scales that are far slower than the internal error

correction utilised by each unit. Physical transport does introduce “catastrophic” failure channels (for example, physically losing the memory unit), but these failure channels do not impact

the analysis presented in this work. Each quantum memory consists of a two-dimensional array of optical cavities with a mean linear spacing of 0.66 mm, each containing a single NV− centre

comprising a spin-half N15 nucleus and a spin-one electron30,33. Each nuclear spin represents a single qubit, and all operations (initialisation, readout, and interactions between

neighbouring qubits) are achieved by hyperfine coupling to the electron spins and dipole-induced transparency in an external optical field. We assume that these operations are fixed to a 3.5

 _μ_s clock time and occur with an independent depolarising error rate of 0.1%, per gate30. Coherent control of spins in diamond has already been demonstrated with an error rate below 1%,

indicating that this target may be achievable in the near future34. Each quantum memory stores a single logical qubit encoded in the surface code, although other codes may also be suitable.

The error-correction protocol involves the continuous execution of physical quantum circuits to determine the error syndrome. This information undergoes local classical processing to detect

and correct errors introduced by decoherence, coupling inefficiency, and other sources, thereby preserving the state of the logical qubit. To determine the effective coherence time of the

quantum memories, we undertook numerical simulations of the error-correction protocol for small arrays. (see the supplementary material). Extrapolating to large arrays, we find that in this

system, a quantum memory of approximately 4200 qubits enables storage of a logical qubit for approximately 40 days with a logical error rate of 10−10 [See the methods section]. Quantum

memories are installed in Twenty-foot Equivalent Unit (TEU) containers, the standard shipping unit with an internal volume of 40 m3. We assume that 1 m3 is occupied by quantum memories and

the remaining 39 m3 is reserved for power, refrigeration, and control infrastructure. Each of these units is the quantum equivalent of a memorystick. More efficient protocols may be

achievable under different assumptions, but for simplicity we assume a single, dedicated Very Large Container Ship (VLCS)-class container ship with a capacity of 104 TEU. We consider a

shipping channel between Japan and the United States, with freight terminals acting as primary network nodes for traditional repeater networks deployed in each country, as shown in Fig. 1.

Allowing for local transport and maintenance, this channel requires a one-way transport time of 20 days [http://www.joc.com/sailings, “Journal of Commerce, sailing schedules”, (2016) (Date

of access:01/07/2014)]. Table 1 reports the effective transpacific bandwidth of the network mechanism. Our results compare quantum memories based on NV− centres in diamond to quantum

memories based on a range of other qubit implementations28,29,30,35,36,37,38,39 illustrating the dependence of the bandwidth on the underlying physical parameters. Each implementation

involves a unique set of technological challenges and our results are predicated on the development of portable systems incorporating high-speed external interfaces to facilitate

lattice-surgery operations between logical qubits40. Nevertheless, bandwidth in excess of 1 THz is feasible under realistic physical assumptions, exceeding even the fastest proposals for

traditional repeater networks. Our results assume a single container ship, but the total bandwidth scales linearly with the total freight capacity, allowing for incremental investment in

infrastructure rather than the overhaul of thousands of kilometres of undersea cables. Furthermore, adding network nodes involves transport to additional locations rather than investment in

wider area infrastructure, and can be done with minimal planning and only a few weeks lead time. In addition to expanding the reach of traditional repeater networks, our mechanism may be

used to hybridise networks with different operating regimes. For example, quantum memories may be used to interconvert between repeater networks with different networking protocols, qubit

implementations, or operating rates40. Quantum memories embedded in portable devices may allow mobile nodes to connect to static networks. Our assumptions regarding the amount of space

within each unit reserved for the actual qubits and necessary control infrastructure are somewhat arbitrary due to the unknown nature of how classical control technology will develop. An

underlying assumption of this work is that a high speed, high fidelity quantum network is only describable in a future where large-scale quantum technology exists. While research related to

the classical control of active quantum memories is in its infancy32,42,43, initial efforts in commercialising quantum technology suggests that our estimates are not overly optimistic.

D-WAVE provides an example of a commercial package that requires cryogenic cooling, power and classical control [The DWAVE two system (www.dwavesys.com/d-wave-two-system) utilises a closed

cycle dilution refrigerator, magnetic shielding, vacuum system and classical control in a size approximately the same as a TEU container]. As quantum technology advances, a network

architecture based on the transport of reliable quantum memories could enable fundamental tests of quantum mechanics over long distances and then increasingly sophisticated applications

ranging from quantum cryptography to distributed quantum computing. Our mechanism has the flexibility to service these applications as they develop, in addition to complementing traditional

repeater networks as they are deployed. Eventually, once quantum computers are commonplace, entanglement will be the fungible resource that enables a vast range of distributed applications.

The quantum sneakernet is a mechanism that could feasibly underpin an entanglement-based economy of this kind, connecting users of local quantum networks to a global Quantum Internet.

METHODS CAPACITY OF A MEMORY UNIT Illustrated in Fig. 2 is the structure and performance of a memory unit. The device encodes a single logical qubit of memory [Fig. 2a]. The logical Pauli

operators are chains of physical _X_ and _Z_ operations that connect the top and bottom (logical _X_) or the left and right (logical _Z_) edges of the lattice. Through simulation, we

numerically determine both the fault-tolerant threshold for the memory unit [Fig. 2b] and the expected failure rate as a function of QEC strength at a fixed physical error rate, _p_ [Fig.

2d]. From the behaviour of the code for low values of the physical error rate, _p_, we can estimate the probability that a memory unit fails during one error correction cycle, _P__L_, as a

function of the distance of the topological planar code, _d_ (an error correction cycle requires _d_ rounds of error correction). For an operational device, we assume the error rate for each

physical gate in the quantum memory is, _p_. The functional form for the failure of the code is given by, We use the data from Fig. 2, which simulates a full _d_ rounds of error correction

to estimate _α_ ≈ 0.3 and _β_ ≈ 70. The total number of physical qubits in the memory unit is _N_ = (2_d_ − 1)2 and the total time of a memory correction cycle is _T_corr = 6_td_, where _t_

is the operational time of a _physical_ quantum gate (initialisation, measurement or CNOT), the factor of 6 comes from the six elementary gates necessary to perform syndrome extraction in

the topological code, and we require _d_ rounds of error correction to correct for measurement errors. The total memory time of the unit, _T_mem, is related to the per-error correction cycle

failure probability, _P__L_, and the chosen permissible infidelity of the final entangled link, _P_link = 1 − _F_, where _F_ is the link fidelity (between memory units), Figure 2b shows the

memory time for a device that has a _t_ = 3.5 _μ_s physical gate time (appropriate for optically coupled NV− 30), as a function of total number of physical qubits, _N_, and desired final

link infidelity, _P_link. The contour where the memory unit can maintain coherence for one year and achieve the desired link fidelity is plotted with a heavy line. Similar plots can be

easily obtained from Eqn. 2 for different physical gate times, _t_. As it is assumed that the physical system is at a fixed physical error rate _p_ = 0.1% (or _p_ = 0.001%) regardless of the

intrinsic gate speed of the system, _t_, memory times will increase with slower systems. Ion trap computers will have a _longer_ memory time than donor-based systems as we assume _both_

technologies can achieve a _p_ = 0.1% (0.001%) error rate on all fundamental gates. For a _N_ = 4225 qubit memory unit for the optically coupled NV− system. Taking days as our target memory

time, we find the link infidelity achievable is approximately _P_link ≈ 10−10 (We assume a 40 day storage time for a 20 day transit time to account for preparing and consuming the Bell

states at the source or destination. This in practice can be reduced to 20 days by strategic choices of which Bell states to prepare and consume at given points in time, but this does not

significantly change the size of each memorystick). For all other technologies we recalculate the size of the memory unit to achieve the same infidelity and memory time given the physical

gate time, _t_, and the physical error rate, _p_. LATTICE SURGERY OPERATIONS The planar code (and all toric code derivatives) allows a logical two-qubit CNOT gate to be executed as a

transversal operation using individual CNOT gates applied between corresponding qubits in each memory unit. While fault tolerant, this method may be difficult to implement due to the

difficulty of ensuring that each qubit in the 2D memory cell can interact with the corresponding qubit in another cell. A different approach, called _lattice surgery_, partially solves this

problem by realising a fault-tolerant CNOT gate between two memory units by only using interaction between qubits along an edge of each memory unit. Lattice surgery works by merging two

separate lattices, each containing a single logical qubit encoded in the planar code, into a single oblong lattice, then splitting up this single planar code again. The merging operation is

done by matching the edges of two distinct logical qubits and measuring code stabilisers spanning the lattice cells. This effectively reduces a two qubit encoded system to a single encoded

qubit. This merging takes the state to , where . The measurement of the stabilisers to perform a merge must occur _d_ times, where _d_ is the effective code distance of each planar code.

This protects against faulty qubit measurements for each stabiliser measurement. Given that the quantum circuit required to measure the stabilisers for the planar code requires 6 physical

gates, the merge operation requires a time of _T_ = 6_td_, for physical gate times _t_. The splitting operation is executed by physically measuring the qubits along the merged edge to divide

the single lattice back into two individual lattices. The effect of a split operation is to take the single logical state encoded in the joint lattice, to the two-qubit state, . Once again

to protect against measurement errors, error correction of both lattices must be run for a total of _d_ cycles, requiring a total time of _T_ = 6_td_ for the split operation. Given these

transformations, we can construct a Bell state between two encoded memory units by initialising a _d_ × _d_ lattice holding a logical qubit in one memory unit in the state and a logical

qubit in the other memory unit in the state, merge the edges of the lattices across the optical interface between units to form a single state in a 2_d_ × _d_ distance lattice, and then

split them again to create the state , with one logical qubit held in each memory unit. This state can be manipulated through transversal Hadamard operations on each memory cell and/or

_X__L_ and _Z__L_ to any of the three other Bell states in either the _X_- or _Z_-basis. The total time for the split/merge operation will be for a physical gate time of _t_ and a memory

cell containing _N_ qubits. For the NV− design described above, _t_ = 3.5 _μ_s and for _N_ = 4225, _T_ ≈ 1.4 ms. NETWORK OPERATIONAL PROCEDURES Our network protocol ensures that the

effective transoceanic bandwidth is limited only by the freight capacity and the transport time. A total of seven shipping containers are utilised for each “online” pair. Two units are

permanently located at each shipping terminal. Three mobile units rotate locations; at any point in time, one is at each terminal (_A_ or _B_) and the third is aboard ship. The protocol is

separated into three phases, which operate sequentially in each direction. In the first phase, each mobile logical qubit is entangled with a stationary logical qubit at the origin to

establish logical Bell pairs. In the second phase, one logical qubit in each logical Bell pair is transported from the origin to the terminus, undergoing continuous error correction. In the

third phase, the logical qubits in the logical Bell pairs are entangled with additional logical qubits at the origin and the terminus and then measured to provide end-to-end entanglement

swapping at the logical level. For example, consider the case in which a stationary unit sitting at terminal _A_ is entangled with a mobile memory unit at terminal _B_, and this pair is used

as the online pair for supplying terminal-to-terminal entanglement to other parts of the network. After the remote entanglement supply in the mobile unit is exhausted, it will be

re-entangled with another stationary memory unit at its current location. A second mobile memory unit is aboard ship, entangled with a stationary memory unit at the shipping terminal from

which it departed, carrying entanglement from _B_ to _A_. A third mobile memory unit at _A_ is creating entanglement with a stationary local partner in preparation for shipping. This ensures

that ships are never transporting inactive (unentangled) memory units. The fixed constraints on the bandwidth of a link are latency of the ship, the capacity of a memory unit, and the

physical gate time, from which we can derive additional operational procedures and hardware development goals. The fixed 20-day transit time for the Japan-U.S. link serves as an upper bound

for completing the entanglement of a mobile memory unit with a stationary memory unit, and for consuming the entanglement after shipping. The _T_ = 1.4 ms logical Bell pair creation time

above arises from a surface code distance _d_ = 33 and gate operation time _t_ = 3.5 _μ_s, for an NV− optical implementation30, and assumes that inter-container operations can be executed at

the same rate as operations local to each memory unit. (For the optical NV− system, this is a reasonable assumption, because the intra-container operations use the same physical mechanism

as the inter-container operations.) At this operation rate, the entanglement of a memorystick containing approximately 12.7 KEb (Kilo-Entangled-bit) in the NV− optical approach is created or

consumed in 18 s, and at full rate an entire shipload of entanglement would be consumed in a little over two days. Thus, inter-container operations may be 10× slower without impacting the

performance even if only one container at a time out of an entire shipload is used online. To avoid long periods of unavailability of the network, it may be desirable to limit the rate at

which entanglement is provided to applications to one-tenth of the achievable physical rate. The denser memory subsystems, differing physical gate times, and varying code distances for other

options in Table 1 will result in different demands on the inter-container interfaces. Because of the generic nature of the created entanglement, slow inter-container interfaces can be

compensated for by having more than a single container online. To achieve the performance in the right hand column of Table 1, the containers on board ship must collectively provide that

much bandwidth. For the first three entries in the table, we expect that having a single container online with a simple serial interface will be sufficient. For the higher data rates,

parallelism can be used both at the container interface and by having all 104 containers online at the same time. Each container must provide 106–108 logical entanglements/second. These

entanglement operations may be multiplexed through a single connection, or more likely carried in parallel through a parallel waveguide bundle. Even so, achieving this level of performance

will require advances in optical entanglement methods for the physical technologies. Thus, this table serves as a performance target for experimental work. ADDITIONAL INFORMATION HOW TO CITE

THIS ARTICLE: Devitt, S. J. _et al._ High-speed quantum networking by ship. _Sci. Rep._ 6, 36163; doi: 10.1038/srep36163 (2016). PUBLISHER’S NOTE: Springer Nature remains neutral with

regard to jurisdictional claims in published maps and institutional affiliations. REFERENCES * Bennett, C. H. & Brassard, G. quantum cryptography: Public key distribution and coin

tossing. _Proc. IEEE International Conference on Computers, Systems and Signal Processing_, Bangalore, India: IEEE 175, 8 (December 10–12 1984). * Ekert, A. K. Quantum cryptography based on

Bell’s theorem. Phys. Rev. Lett. 67, 661 (1991). Article  CAS  ADS  MathSciNet  Google Scholar  * Ben-Or, M. & Hassidim, A. Fast quantum Byzantine agreement. _Proc. thirty-seventh annual

ACM symposium on Theory of computing_, Baltimore, USA: ACM. pages 481–485 (2005, May 22–24). * Buhrman, H. & Rohrig, H. Distributed Quantum Computing. Mathematical Foundations of

Computer Science pages 1–20 (2003). * Broadbent, A., Fitzsimons, J. & E. Kashefi . Universal blind quantum computation. _Proc. 50th Annual IEEE Symposium on Foundations of Computer

Science (FOCS2009_), Atlanta, USA: IEEE. pages 517–526 (October 27–29 2009). * Gottesman, D., Jennewein, T. & Croke, S. Longer-Baseline Telescopes Using Quantum Repeaters. Phys. Rev.

Lett., 109, 070503 (2012). Article  ADS  Google Scholar  * Bartlett, S. D., Rudolph, T. & Spekkens, R. W. Reference frames, superselection rules, and quantum information. Rev. Mod. Phys.

79, 555 (2007). Article  CAS  ADS  MathSciNet  Google Scholar  * Jozsa, R., Abrams, D. S., Dowling, J. P. & Williams, C. P. Quantum Clock Synchronization Based on Shared Prior

Entanglement. Phys. Rev. Lett. 85, 2010 (2000). Article  CAS  ADS  Google Scholar  * Kimble, H. J. The Quantum Internet. Nature (London), 453 1023–1030 (2008). Article  CAS  ADS  Google

Scholar  * Lloyd, S. et al. Infrastructure for the quantum internet. ACM SIGCOMM Computer Communication Review 34, 9–20 (2004). Article  Google Scholar  * Van Meter, R. Quantum Networking.

Wiley-ISTE (2014). * Briegel, H. J., Dür, W., Cirac, J. I. & Zoller, P. Quantum Repeaters: The role of imperfect local operations in quantum communication. Phys. Rev. Lett. 81, 5932

(1998). Article  CAS  ADS  Google Scholar  * Fowler, A. G. et al. Surface Code Quantum Communication. Phys. Rev. Lett., 104, 180503 (2010). Article  ADS  Google Scholar  * Jiang, L. et al.

Quantum repeater with encoding. Phys. Rev. A. 79, 032325 (2009). Article  ADS  Google Scholar  * Li, Y., Barrett, S. D., Stace, T. M. & Benjamin, S. C. Long range failure-tolerant

entanglement distribution. New. J. Phys. 15, 023012 (2013). Article  ADS  Google Scholar  * Wooters, W. K. & Zurek, W. H. A Single Quantum Cannot be Cloned. Nature (London) 299, 802

(1982). Article  ADS  Google Scholar  * Ritter, S. et al. An Elementary Quantum Network of Single Atoms in Optical Cavities. Nature (London) 484, 195–200 (2012). Article  CAS  ADS  Google

Scholar  * Hucul, D. et al. Modular Entanglement of Atomic Qubits using Both Photons and Phonons. Nature (Physics). 11, 37–42 (2015). CAS  ADS  Google Scholar  * Muralidharan, S., Kim, J.,

Lutkenhaus, N., Lukin, M. D. & Jiang, L. Ultrafast and Fault-Tolerant Quantum Communication across Long Distances. Phys. Rev. Lett. 112, 250501 (2014). Article  ADS  Google Scholar  *

Azuma, K., Tamaki, K. & Lo, H.-K. All photonic quantum repeaters. Nature Communcations. 6, 6787 (2013). ADS  Google Scholar  * Munro, W. J., Harrison, K. A., Stephens, A. M., Devitt, S.

J. & Nemoto, K. From quantum multiplexing to high-performance quantum networking. Nature Photonics 4, 792–796 (2010). Article  CAS  ADS  Google Scholar  * Devitt, S. J., Munro, W. J.

& Nemoto, K. High Performance Quantum Computing Prog. Informatics. 8, 49–55 (2011). Article  Google Scholar  * Boone, K. et al. Entanglement over global distances via quantum repeaters

with satellite links. Phys. Rev. A. 91, 052325 (2014). Article  ADS  Google Scholar  * Dennis, E., Kitaev, A., Landahl, A. & Preskill, J. Topological Quantum Memory. J. Math. Phys. 43,

4452 (2002). Article  ADS  MathSciNet  Google Scholar  * Peres, A. Delayed choice for entanglement swapping. J. Mod. Opt. 47, 139–143 (2000). Article  ADS  MathSciNet  Google Scholar  * Ma,

X.-S. et al. Experimental delayed-choice entanglement swapping. Nature Physics 8, 479–484 (2012). Article  ADS  Google Scholar  * Bennett, C. H. et al. Teleporting an unknown quantum state

via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 70, 1895 (1993). Article  CAS  ADS  MathSciNet  Google Scholar  * Cody Jones, N. et al. A Layered Architecture for

Quantum Computing Using Quantum Dots. Phys. Rev. X. 2, 031007 (2012). Google Scholar  * Yao, N. Y. et al. Scalable Architecture for a Room Temperature Solid-State Quantum Information

Processor. Nature Communications, 3, 800 (2012). Article  CAS  ADS  Google Scholar  * Nemoto, K. et al. Photonic architecture for scalable quantum information processing in NV-diamond. Phys.

Rev. X. 4, 031022 (2014). Google Scholar  * Barends, R. et al. Logic gates at the surface code threshold: Superconducting qubits poised for fault-tolerant quantum computing. Nature (London)

508 500–503 (2014). Article  CAS  ADS  Google Scholar  * Devitt, S. J. Classical Control of Large-Scale Quantum Computers. RC2014, Springer Lecture Notes on Computer Science (LNCS) 8507,

26–39 (2014). Article  MathSciNet  Google Scholar  * Derntl, C. et al. Arrays of open, independently tunable microcavities. Optics Express 22, 22111–22120 (2014). Article  ADS  Google

Scholar  * Dolde, F. et al. High-Fidelity spin entanglement using optimal control. Nature Communications 5, 3371 (2014). Article  ADS  Google Scholar  * Benhelm, J., Kirchmair, G., Roos, C.

F. & Blatt, R. Towards fault-tolerant quantum computing with trapped ions. Nature Physics 4, 463 (2008). Article  CAS  ADS  Google Scholar  * Kumph, M. et al. Operation of a planar

electrode ion trap array with adjustable RF electrodes. New. J. Phys. 18, 023047 (2014). Article  Google Scholar  * Hollenberg, L. C. L., Greentree, A. D., Fowler, A. G. & Wellard, C. J.

Two-Dimensional Architectures for Donor-Based Quantum Computing. Phys. Rev. B. 74, 045311 (2006). Article  ADS  Google Scholar  * Morton, J. J. L. A Silicon-based cluster state quantum

computer. _arXiv:0905.4008_ (2009). * DiVincenzo, D. P. Fault-tolerant architectures for superconducting qubits. Phys. Scr. T137 (2009). * Nagayama, S., Choi, B.-S., Devitt, S. J., Suzuki,

S. & Van Meter, R. Interoperability in encoded quantum repeater networks Phys. Rev. A. 93, 042338 (2016). Article  ADS  Google Scholar  * Fowler, A. G. Minimum weight perfect matching of

fault-tolerant topological quantum error correction in average _O_(1) parallel time Quant. Inf. Comp. 15, 0145–0158 (2015). MathSciNet  Google Scholar  * Devitt, S. J., Fowler, A. G.,

Tilma, T., Munro, W. J. & Nemoto, K. Classical Processing Requirements for a Topological Quantum Computing System Int. J. Quant. Inf. 8, 1–27 (2010). Article  Google Scholar  * Horsman,

C., Fowler, A. G., Devitt, S. J. & Van Meter, R. Surface code quantum computing by lattice surgery. New. J. Phys. 14, 123011 (2012). Article  ADS  MathSciNet  Google Scholar  Download

references ACKNOWLEDGEMENTS S.J.D. acknowledges support from the JSPS Grant-in-aid for Challenging Exploratory Research and NICT, Japan. R.V. and S.J.D. acknowledge support from JSPS KAKENHI

Kiban B 25280034. R.V. acknowledges that this project has been made possible in part by a gift from the Cisco University Research Program Fund, a corporate advised fund of Silicon Valley

Community Foundation. A.D.G. acknowledges the A.R.C. for financial support (DP130104381). A.M.S. acknowledges support from NICT, Japan. AUTHOR INFORMATION AUTHORS AND AFFILIATIONS * Center

for Emergent Matter Science, RIKEN, Wakoshi, 315-0198, Saitama, Japan Simon J. Devitt * Australian Research Council Centre of Excellence for Nanoscale BioPhotonics, and Chemical and Quantum

Physics, School of Science, RMIT University, Melbourne 3001, Australia Andrew D. Greentree * National Institute of Informatics, 2-1-2 Hitotsubashi, Chiyoda-ku, 101-8430, Tokyo, Japan Ashley

M. Stephens * Faculty of Environment and Information Studies, Keio University, Fujisawa, 252-0882, Kanagawa, Japan Rodney Van Meter Authors * Simon J. Devitt View author publications You can

also search for this author inPubMed Google Scholar * Andrew D. Greentree View author publications You can also search for this author inPubMed Google Scholar * Ashley M. Stephens View

author publications You can also search for this author inPubMed Google Scholar * Rodney Van Meter View author publications You can also search for this author inPubMed Google Scholar

CONTRIBUTIONS S.J.D. and A.D.G. conceived the idea. A.M.S. undertook the numerical simulations. R.V. devised the network protocol. All authors contributed to the writing of the manuscript.

ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing financial interests. RIGHTS AND PERMISSIONS This work is licensed under a Creative Commons Attribution 4.0

International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the

material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit

http://creativecommons.org/licenses/by/4.0/ Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Devitt, S., Greentree, A., Stephens, A. _et al._ High-speed quantum networking by

ship. _Sci Rep_ 6, 36163 (2016). https://doi.org/10.1038/srep36163 Download citation * Received: 06 June 2016 * Accepted: 11 October 2016 * Published: 02 November 2016 * DOI:

https://doi.org/10.1038/srep36163 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry, a shareable link is not currently

available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative