ABSTRACT Spectrally resolved photoacoustic imaging is promising for label-free imaging in optically scattering materials. However, this technique often requires acquisition of a separate

image at each wavelength of interest. This reduces imaging speeds and causes errors if the sample changes in time between images acquired at different wavelengths. We demonstrate a solution

to this problem by using dual-comb spectroscopy for photoacoustic measurements. This approach enables a photoacoustic measurement at thousands of wavelengths simultaneously. In this

technique, two optical-frequency combs are interfered on a sample and the resulting pressure wave is measured with an ultrasound transducer. This acoustic signal is processed in the

resolved photoacoustic imaging. SIMILAR CONTENT BEING VIEWED BY OTHERS MID-INFRARED CROSS-COMB SPECTROSCOPY Article Open access 24 February 2023 PHOTO-ACOUSTIC DUAL-FREQUENCY COMB

SPECTROSCOPY Article Open access 20 August 2020 DUAL-COMB PHOTOTHERMAL SPECTROSCOPY Article Open access 21 April 2022 INTRODUCTION In vivo and in vitro medical imaging and spectroscopy face

the fundamental challenge of strong optical scattering in biological tissues. This challenge has led researchers to use the photoacoustic (PA) effect, where localized light absorption

generates acoustic waves that can be detected from deep within tissue1,2,3,4. PA measurements have an advantage over pure optical methods because light only has to travel through the sample

to the absorber to create an acoustic wave, but the light does not need to travel back out of the sample to a detector. Other advantages of PA measurements are that scattered optical

illumination can still induce a PA signal, and the generated acoustic waves are only weakly scattered by tissue5. In addition, by measuring the PA response versus excitation wavelength, it

is possible to identify and quantify substances based on their unique absorption features. For example, researchers have used this method, called PA spectroscopy, to quantify glucose levels

in vivo6,7,8. A more advanced technique for medical diagnostics and functional imaging, called multispectral optoacoustic tomography (MSOT) or photoacoustic tomography (PAT), has also been

demonstrated. This technique provides images of multiple exogenous and endogenous species at multiple wavelengths and uses spectral unmixing algorithms to determine the spatial distribution

of each species2,9,10. This technique has been used to differentiate adipose tissue from cholesterol11,12, measure intramuscular fat distributions13, map tumor size and shape14,15, identify

white matter loss in spinal cord injuries16, quantify blood oxygen saturation17,18, and even to construct a label-free molecular map of a mouse fibroblast cell19. One challenge of PA

spectroscopy, or PAS, measurements is that they require a broadband optical excitation source with a spectrally resolved detection or illumination method. Traditionally, PA researchers have

used optical parametric oscillators or other tunable lasers, which require sequential image acquisition over narrow optical-frequency bands. Although it is possible in simple ratiometric PAS

to use only a couple of optical-frequency bands, measuring in more bands allows the identification of additional species and improves detection sensitivity20. However, sequentially scanning

the optical frequency can be time consuming and can lead to potential issues with sample drift or damage between images at different optical frequencies9,21. Broadband thermal sources using

a Fourier transform spectrometer (FTS)22,23,24,25 for optical-frequency resolution have been used to overcome sequential scanning, but the low optical power and slow scanning delay arm have

limited the application of these systems. Alternatively, broadband supercontinuum coherent laser sources may provide higher power than a thermal source, but still require either sequential

frequency scanning or physical scanning of an FTS delay arm and can suffer from high laser intensity noise. Optical frequency combs26,27,28 are an alternative light source for broadband PAS

and multispectral PAT because they simultaneously generate thousands of discrete optical-frequency bands. These frequency bands are called comb teeth because, like the teeth of a comb, they

are evenly spaced and very narrow, with absolute linewidths below 120 kHz29. The comb teeth of a frequency comb are temporally and spatially coherent and propagate in a single spatial mode

with high brightness. Recent work has shown that frequency combs are a promising light source for PAS. Researchers have demonstrated highly sensitive and precise measurements of methane

absorption spectra with frequency-comb-based PAS30,31. These demonstrations relied on a FTS for spectral selectivity and thus required physical scanning of the optical pathlength. It is

possible, however, to eliminate the need for the FTS altogether by using a second comb like a local oscillator. By exploiting the coherence of optical-frequency combs, one can capture an

entire absorption spectrum on a single photodetector without wavelength or delay arm scanning, as is done in dual-comb spectroscopy (DCS)32. DCS provides broad spectral coverage, high

sensitivity, and high spectral resolution for identification of multiple chemical species33,34,35,36,37. It also can provide rapid spectral measurements for monitoring dynamic biological

processes38, as well as for coherent Raman imaging39,40. These advantages should be applicable to PAS using a technique analogous to DCS. This method of dual-comb PAS (DCPAS), which was

suggested in ref. 31, can be used across broad spectral windows without sequential wavelength scanning and can be implemented with a compact, stable, and robust dual-comb spectrometer. In

this article, we report an experimental demonstration of DCPAS. We use two self-referenced fiber-laser frequency combs to measure the PA spectra of thick polymer films over an ≈15 THz

spectral bandwidth centered at 173 THz. With coherent averaging we obtain spectrally resolved PA responses of polydimethylsiloxane (PDMS) and paraffin samples immersed in water. These

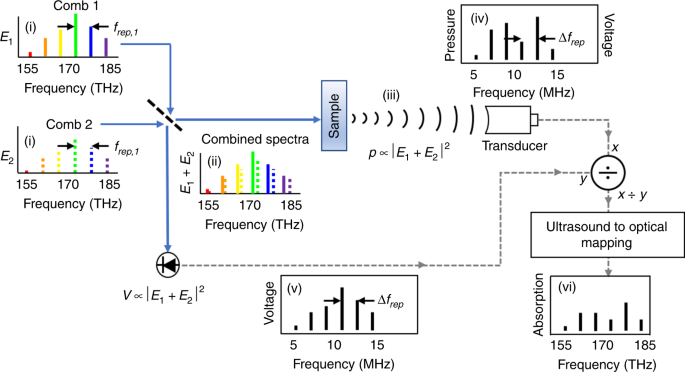
experiments reveal that dual-comb optical excitation can generate PA signals and that these signals can be used to detect and identify organic materials. RESULTS DCPAS CONCEPT Figure 1

describes the concept of DCPAS. The DCPAS system is based on laser frequency combs whose frequency-domain output is tens of thousands of evenly spaced narrow lines or comb “teeth”. These

teeth are spaced at the frequency-comb’s pulse repetition rate, _f_rep, ((i) in Fig. 1)27. In the dual-comb approach, we use two such frequency combs where the repetition rate of comb 2,

_f_rep,2, is slightly larger than the repetition rate of comb 1, _f_rep,1, such that _f_rep,2 − _f_rep,1 = Δ_f_rep. As a result, the frequencies of subsequent pairs of comb teeth are offset

by increasing increments of Δ_f_rep, as illustrated in Fig. 1. The optical intensity of the combined combs is given by \(I_0 = \left| {E_1 + E_2} \right|^2\), where _E_1 is the electric

field of the light from comb 1 and _E_2 is the electric field of the light from comb 2. This intensity contains a multi-heterodyne term corresponding to the beat notes of each, increasingly

offset, pair of comb teeth. Assuming the zeroth tooth of comb 1, at frequency _v_1,0, overlaps with the zeroth tooth of comb 2, at frequency _v_2,0 = _v_1,0, then the beat notes occur at

radio frequencies, _f__i_, given by $$\begin{array}{*{20}{c}} {f_1} & = & {\nu _{2,1} - \nu _{1,1}} & = & {\left[ {\nu _{2,0} + 1 \times f_{{\mathrm{rep}},2}} \right] - [\nu

_{1,0} + 1 \times f_{{\mathrm{rep}},1}]} & = & {1 \times \Delta f_{{\mathrm{rep}}}} \\ {f_2} & = & {\nu _{2,2} - \nu _{1,2}} & = & {\left[ {\nu _{2,0} + 2 \times

f_{{\mathrm{rep}},2}} \right] - \left[ {\nu _{1,0} + 2 \times f_{{\mathrm{rep}},1}} \right]} & = & {2 \times \Delta f_{{\mathrm{rep}}}} \\ {f_3} & = & {\nu _{2,3} - \nu

_{1,3}} & = & {\left[ {\nu _{2,0} + 3 \times f_{{\mathrm{rep}},2}} \right] - \left[ {\nu _{1,0} + 3 \times f_{{\mathrm{rep}},1}} \right]} & = & {3 \times \Delta

f_{{\mathrm{rep}}}} \\ \vdots & = & \vdots & = & \vdots & = & \vdots \\ {f_n} & = & {\nu _{2,n} - \nu _{1,n}} & = & {\left[ {\nu _{2,0} + n \times

f_{{\mathrm{rep}},2}} \right] - \left[ {\nu _{1,0} + n \times f_{{\mathrm{rep}},1}} \right]} & = & {n \times \Delta f_{{\mathrm{rep}}}} \end{array}$$ (1) As the optical frequencies

_v__i,j_ are typically 10 to 12 orders of magnitude larger than Δ_f_rep, _v_1,_i_ is approximately the same as _v_2,_i_, and it is quite accurate to say that light at the optical-frequency

_v__i_ = _v_1,_i_ ≈ _v_2,_i_ is amplitude modulated at the radio frequency _f__i_. When the sample absorbs light from a pair of comb teeth at optical-frequency _v__i_, it produces a PA

pressure wave with an amplitude, _p_, proportional to the amount of light absorbed by the sample ((iii) in Fig. 1)41,42,43. This proportionality is given by \(p \propto I_0\left( {\nu _i}

\right) \times \left[ {1 - \exp \left( { - \mu _{\mathrm{A}}\left( {\nu _i} \right) \times L} \right)} \right] \times \beta\), where _I_0(_v__i_) is the intensity of the dual-comb excitation

at optical-frequency _v__i_, _μ_A(_v__i_) is the sample’s base-e absorption coefficient at optical-frequency _v__i_, _L_ is the sample thickness that contributes to the PA signal, and _β_

is a proportionality constant that depends on sample material and geometry. The amplitude of this pressure wave is modulated at the multi-heterodyne frequency _f__i_, which can be several

orders of magnitude less than the comb repetition frequencies, _f_rep. When the sample absorbs light from many pairs of comb teeth, at optical frequencies _v_0,_v_1._v_2,…,_v__n_, it

produces a PA pressure wave with frequency components centered at the corresponding modulation frequencies _f_0,_f_1,_f_2,…,_f__n_. As there is a one-to-one correlation between the frequency

_v__i_ of the absorbed light and the resulting acoustic modulation frequency, _f__i_, one can infer the sample’s optical absorption spectrum by analyzing the frequency-domain PA signal. As

every comb tooth is simultaneously incident on the sample and every PA frequency is simultaneously recorded, this technique allows parallel acquisition of thousands of spectral elements and

multiplexed measurement of the sample’s absorption spectrum. It is also useful to consider the time-domain picture. In the time-domain, the DCPAS signal is a series of interferograms, just

as in DCS. Each interferogram reflects the time-dependent optical intensity caused by the interference between successive pulses from the two offset frequency combs. In DCPAS, this

time-dependent optical intensity leads to a time-dependent amplitude modulation of the PA pressure wave, which we detect via an ultrasound transducer. The pressure waves are detected by the

transducer to generate a corresponding time-domain voltage interferogram. We then convert these interferograms to frequency-domain spectra by a Fourier transform to generate the

multi-heterodyne acoustic signal discussed above ((iv) in Fig. 1). This acoustic signal is normalized by the optical excitation spectrum recorded by a photodetector ((v) in Fig. 1). Then the

acoustic frequencies are mapped to optical frequencies using the known parameters of the combs32. This yields the sample’s optical absorption spectrum ((vi) in Fig. 1). One advantage of

DCPAS is that the bandwidth and center frequency of the generated acoustic signal, corresponding to the amplitude modulation on the optical signal, can be adjusted independently of the

optical bandwidth and center frequency. This adjustment is made in a controlled fashion by tuning the frequency locking of the two combs to adjust _v_2,0, _v_1,0, and Δ_f_rep, and therefore,

the multi-heterodyne frequencies _f__i_. This allows any optical spectrum to generate a PA signal that can be detected by an ultrasound transducer despite the transducer’s limited

bandwidth. In this demonstration, we use lasers with ≈160 MHz repetition rates and set the difference in repetition rates (tooth spacings), Δ_f_rep = _f_rep,2−_f_rep,1, to be 66.81 Hz. This

allows us to map the roughly 15 THz optical bandwidth (full width at –10 dB) into an acoustic bandwidth of (15 THz) × (Δ_f_rep/_f_rep) ≈6.3 MHz32 while centering this modulation signal at

the peak of the transducer responsivity, 7.5 MHz. In order to maintain this mapping, as well as the optical coherence required for long-term averaging of the signals, both combs are fully

stabilized in the manner described in ref. 44. EXPERIMENTAL SETUP Figure 2 shows the experimental setup for DCPAS. We use two self-referenced erbium fiber frequency combs at 160 MHz

repetition rate. The output of each comb is amplified and filtered to generate light in a 15-THz-wide band centered at ≈173 THz, selected to coincide with low water absorption and the first

overtone absorption of C–H bonds. This wavelength region has previously been used to image arterial plaques (fatty tissue) in the presence of blood, to distinguish protein and fat, and to

image white matter in a spinal cord4,13. A total of about 24 mW of comb light is incident on the samples. The PA signals generated by the samples then propagate through a water bath to a

polytetrafluoride ultrasound transducer with a frequency response that is peaked at 7.5 MHz with an ≈7.5 MHz bandwidth (see Supplementary Fig. 3). The PA and photodetected signals are

coherently summed in a data acquisition system previously used for mid-infrared DCS34,45. The methods section provides more detail of the experimental setup. DCPAS EXPERIMENTAL RESULTS

Figure 3 shows the DCPAS measurement results from a sample consisting of vertically aligned carbon nanotubes (VACNTs) embedded in PDMS (polydimethylsiloxane). This sample was chosen because

VACNTs have strong optical absorption at ≈170 THz46 and therefore generate a strong PA response when illuminated. This provides a clear demonstration that dual-comb optical excitation (Fig. 

3a) induces a PA response (Fig. 3b). Furthermore, the shape of the PA response reveals that the PA pressure wave is an interferogram that corresponds to the optical interferogram incident on

the sample. The correlation between the optical excitation and the PA response can be probed further by examining the frequency-domain representation of these signals (Fig. 3c). To obtain

the frequency-domain signals, the time-domain signals are apodized with a 4.8 μs window, zero-padded outside of the apodization window, and Fourier transformed. The Fourier transform yields

the spectrally resolved optical excitation and PA response at acoustic frequencies corresponding to the beat frequencies between the teeth of the two frequency combs (top axis of Fig. 3c).

To convert these acoustic or ultrasound frequencies, _f_US, to the optical frequencies, _v_optical, of the comb teeth that generated the signal, we linearly scale the frequency according to:

$$\nu _{{\mathrm{optical}}} = \nu _0 + \frac{{f_{{\mathrm{rep}},1} + f_{{\mathrm{rep}},2}}}{{2 \times \left( {f_{{\mathrm{rep}},2} - f_{{\mathrm{rep}},1}} \right)}} \times

f_{{\mathrm{US}}},$$ (2) where _f_rep,1 is the repetition rate of comb 1, _f_rep,2 is the repetition rate of comb 2, and _v_0 is a constant offset calculated from the comb repetition rates

and carrier-envelope offset frequencies. With the 4.8 μs apodization window used here, the spectral resolution of the measurement is ≈500 GHz or ≈5 nm (due to the zero-padding, the spectrum

is effectively smoothed to 500 GHz). The spectrally resolved PA response shows how a sample’s absorption coefficient depends on optical frequency. At frequencies where the sample absorbs

strongly, there is a strong PA response; whereas, at frequencies where the sample does not absorb, there is no PA response. Therefore, if a sample absorbs uniformly at all frequencies

contained in the optical excitation, the spectrally resolved PA response should have the same shape as the optical excitation spectrum. The spectrally resolved PA response of the VACNT

sample (Fig. 3c, red line) has a frequency dependence that is similar to the optical excitation spectrum (Fig. 3c, black line). This similarity shows that the PA response is dependent on the

optical excitation spectrum. The relatively weaker amplitude of the PA response for optical frequencies \(\nu _{{\mathrm{optical}}} \, \lesssim \, 166\,{\mathrm{THz}}\) and \(\nu

_{{\mathrm{optical}}} \, \gtrsim \, 176\,{\mathrm{THz}}\) is due to a roll-off in the transducer’s responsivity away from its peak responsivity at _f_US = 7.5 MHz (which corresponds to

_v_optical = 171.2 THz). There are small (around 20%) variations in the PA response relative to the optical excitation spectrum for optical frequencies between 166 THz and 176 THz whose

origins are unknown, but could be caused by sample inhomogeneities. Despite these variations, the VACNT target was useful for characterizing the DCPAS system and for demonstrating that

dual-comb excitation can induce a PA interferogram. However, the VACNT’s absorption spectrum is not representative of a biological material because it lacks a characteristic C-H overtone in

the 175-THz band. To observe the C-H overtone absorption, we measured two different polymer thin films: polydimethylsiloxane (PDMS) and paraffin, both of which are much more weakly absorbing

in this spectral region than the VACNT sample. The PA response of PDMS and paraffin samples are shown in Fig. 4a, b, respectively, for several different averaging times. As the polymers

absorb more weakly than the VACNTs and are less efficient at generating acoustic waves, they produce a DCPAS signal that is 50× and 100× weaker than the VACNTs, as can be seen from comparing

Fig. 3c to Fig. 4a, b, respectively. The spectral features of the PDMS response are somewhat distinguishable over the noise with as little as 4 min of signal averaging and are easily

distinguishable with 20 min of averaging. On the other hand, the spectral features of the paraffin response are readily apparent even at 4 min of averaging due to the approximately two times

stronger signal from the paraffin film. Figure 4c, d compare these PA responses to the samples’ optical absorption spectra measured with a spectrophotometer. In order to make this

comparison, we need to account for several effects inherent to the DCPAS measurement. As mentioned in the introduction, the amplitude of the PA pressure wave is proportional to the sample’s

optical absorbance, the intensity of the optical excitation at the sample, and other things such as acoustic impedance mismatch and the sample’s acoustic response. The measured PA response

is proportional to the pressure wave’s amplitude multiplied by the transfer function of the transducer47,48. For weakly absorbing samples like the paraffin and PDMS in these experiments, the

exponential in Beer’s law can be linearized, and we can write the measured PA response as $$V_{{\mathrm{PA}}}\left( {\nu _{{\mathrm{optical}}}} \right) \approx \beta \times \mu

_{\mathrm{A}}\left( {\nu _{{\mathrm{optical}}}} \right) \times I_0\left( {\nu _{{\mathrm{optical}}}} \right) \times H_{{\mathrm{transducer}}}\left( {\nu _{{\mathrm{optical}}}} \right),$$ (3)

where _V_PA is the measured PA response as a function of optical frequency, _v_optical, _β_ is a proportionality factor to convert from sample absorption to PA pressure, which may be

material and geometry dependent, _μ_A(_v_optical) is the sample’s optical absorption coefficient at optical frequency _v_optical, _I_0(_v_optical) is the intensity of the optical excitation

at optical frequency _v_optical, and _H_transducer (_v_optical) is the ultrasound transducer’s frequency-dependent responsivity after scaling to optical frequencies according to Eq. (2). An

apparent optical-frequency dependence of _β_ could arise from acoustic-frequency-dependent pressure generation or attenuation; however, here we assume that _β_ is constant over the acoustic

frequencies used for the amplitude modulation in these experiments. _H_transducer is obtained from calibration curves provided by the manufacturer (Supplementary Fig. 3), and

_I_0(_v_optical) is measured via the photodetected DCS spectrum. Thus, the normalized PA response of a sample is $$\widehat {V_{{\mathrm{PA}}}}\left( {\nu _{{\mathrm{optical}}}} \right) =

\frac{{V_{{\mathrm{PA}}}\left( {\nu _{{\mathrm{optical}}}} \right)}}{{I_0\left( {\nu _{{\mathrm{optical}}}} \right) \times H_{{\mathrm{transducer}}}\left( {\nu _{{\mathrm{optical}}}}

\right)}} = \beta \times \mu _{\mathrm{A}}\left( {\nu _{{\mathrm{optical}}}} \right).$$ (4) Figure 4c, d show the result of normalizing the polymers’ PA responses according to Eq. (4). For

comparison, these figures also show the optical absorbance spectra, ∝_μ_A(_v_optical), of PDMS and paraffin measured in a transmission-mode spectrophotometer. The normalized PA responses

reflect the absorbance spectra from the spectrophotometer measurements. The DCPAS measurement reveals prominent PDMS absorbance peaks at 171.6 THz, 176.0 THz, 177.4 THz. The location of

these peaks are in good agreement with the spectrophotometer absorbance measurement and previously published results49,50. The normalized PA response of paraffin contains well-defined

absorbance peaks at 169.8 THz and 173.1 THz. Although we could not find literature showing paraffin absorbance spectra in the 165 THz to 180 THz window, the absorbance peaks from the DCPAS

measurement closely match the peaks from our spectrophotometer measurement, and the peak at 173.1 THz agrees well with the location of a reported absorbance peak51. The results shown in Fig.

 4 prove that DCPAS is sensitive to the absorption spectra of polymer samples. However, the signal-to-noise ratio (SNR) of this proof-of-concept DCPAS system is low for weakly absorbing

features such as the C-H overtone bands in these polymer films. Thus, long averaging times are currently required to obtain spectrally resolved responses like those in Fig. 4. Figure 5a

shows the time-domain SNR versus averaging time for both VACNTs and the polymer samples. (The time-domain SNR is defined in the Methods section and Supplementary Note 1.) In all cases the

SNR is proportional to the square root of averaging time, as expected for white noise. The VACNT sample requires 4 orders of magnitude less averaging time than the polymer samples to reach

the same SNR because the VACNTs produce an ≈100× stronger PA signal than the polymers. Figure 5b shows that the SNR is linearly proportional to the power of the optical excitation. This

proportionality indicates that the SNR of the system is limited by detector noise and not subject to saturation effects even for the strongly absorbing VACNT sample. This lack of saturation

suggests that higher SNR measurements of organic films are feasible with a higher-power dual-comb source. DISCUSSION The reported results highlight the potential of DCPAS for label-free

imaging without spectral scanning. As shown in Fig. 5, a high SNR ( >300) can be reached through coherent averaging. However, the averaging times required for high spectral SNR are likely

too long to be useful, especially for medical imaging applications, and it is useful to consider potential routes to reduce the acquisition time. First, the signal strength could be

improved by operating at a wavelength range with larger molecular absorption cross-sections. For example, the PA signal from the VACNT sample has a much higher SNR than that of the PDMS

sample because the VACNTs absorb light much more strongly than the PDMS in this wavelength region. In this proof-of-principle experiment, we focused on the optical window at ≈173 THz because

it was easily accessible with our laser system, contains first overtone absorption by C–H bonds, and has lower water absorption than adjacent frequency bands13. However, mid-infrared

frequency combs34,35,38,52 could measure much stronger fundamental C-H rovibrational transitions. These stronger absorption features (up to several orders of magnitude) could provide a

proportional increase in the PA signal strength and a corresponding quadratic decrease in the required averaging time. However, strong water absorption would limit mid-infrared DCPAS to

<100 μm penetration depths in biological tissue because the 1/e penetration depth is only 32 μm for excitation at _v_optical = 60 THz53. Nevertheless, mid-infrared DCPAS could be useful

for measuring thin samples in the same way that pulsed PAS can measure thin samples at mid-infrared wavelengths8. Moving to visible/near-infrared wavelengths would enable measurements of

oxygenated and deoxygenated hemoglobin. This visible/near-infrared hemoglobin absorption is about ten times stronger than lipid absorption at around 173 THz without any competing absorption

from water4. Additionally, other exogenous agents and dyes with strong absorption features could be measured with visible/near-infrared wavelengths9. The acquisition time could also be

reduced by use of a higher comb repetition frequency. In DCPAS, the fundamental sample point spacing of the measured spectrum is set by the comb repetition rate, _f_rep. In this

demonstration, the spectral sample spacing is _f_rep = 160 MHz, much finer than any absorption feature of interest. For this reason, we apply a 4.8-μs apodization window before the Fourier

transformation. This apodization improves the spectral SNR by a factor of \(\sqrt {500\,{\mathrm{GHz/}}160\,{\mathrm{MHz}}} \approx 56\) and smooths the frequency-domain response to a

spectral resolution of ≈500 GHz. This 500-GHz resolution is acceptable for the envisioned applications because the absorption features in tissue samples are typically 100 s of GHz to 1000 s

of GHz wide. For instance, the full-widths at half-maximum of lipid absorption spectra at 172 THz are ≈6000 GHz, and the absorption peaks of plaque lipids and adipose lipids are separated by

about 1200 GHz12. However, rather than smoothing the frequency-domain response via apodization, it would be better if the fundamental sample spacing, _f_rep, matched the desired resolution.

In other words, there is an SNR penalty because the fundamental spectral sample spacing is not matched to the desired resolution. This SNR penalty can be understood by recognizing that a

wider tooth-spacing, with a fixed total power, results in a greater power per comb tooth. Taking this into account, one finds that the required averaging time should scale linearly with

1/_f_rep for a fixed output power and fixed SNR up until the repetition rate matches the desired resolution54. It is possible to generate frequency combs with 10 GHz repetition rates from a

mode locked laser55,56, quantum cascade laser-based combs38, or the use of electro-optic frequency combs57,58, while microresonator-based frequency combs could provide 500 GHz repetition

rates59,60. A 500 GHz repetition rate would result in a ≈3000-fold reduction in averaging time, or, for a given averaging time a \(\sqrt {3000} = 55 \times\) improvement in SNR. However,

there are challenges to increasing the repetition rate while simultaneously maintaining the average comb output power and spectral coverage. Nonetheless, recent reports of dark-pulse

microresonators and dissipative Kerr soliton combs have shown >200 GHz repetition rate and on-chip powers exceeding 10 mW61,62. With further improvements in efficiency and output

coupling, these comb sources could be well-suited for DCPAS. If we assume a 500-GHz repetition rate, the same excitation power used here, and a tenfold stronger absorbance by illuminating in

the visible or mid-infrared, the acquisition time is reduced by a factor of 300,000 so that a 2-h acquisition time drops to 24 milliseconds. Depending on the application, this could be

further reduced by increasing the incident optical power. As shown in Fig. 5b, a linear increase in optical power leads to a linear improvement in SNR and to a corresponding quadratic

decrease in the required averaging time. In some cases, additional considerations will limit the incident power for a given application. For example maximum permissible exposure limits for

in vivo samples63 and potential thermal damage for in vitro samples must be avoided. In addition to increasing the incident optical power, the 24-millisecond acquisition time could also be

decreased by decreasing the measurement noise. As the PA noise floor is limited by the transducer noise, improved transducer readout electronics or lower-noise transducing elements would

directly improve the SNR43 and lead to a quadratic reduction in acquisition time. In this initial demonstration of DCPAS, we did not choose an optimal transducer, but rather the 7.5 MHz

transducer used is similar to transducers typically employed for conventional PAS experiments. The ability to adjust the multi-heterodyne frequencies of the optical excitation in DCPAS would

allow the use of transducers with center frequencies and bandwidths both ranging from ≈1 MHz to ≈100 MHz. The arguments mentioned above suggest that DCPAS could be useful for applications

such as spectrally resolved medical imaging. In addition to considering these arguments, we also evaluated the potential of DCPAS by directly comparing DCPAS with a PAS system using a

tunable pulsed optical parametric oscillator (OPO). As shown in Supplementary Fig. 1 and Supplementary Fig. 2, the current DCPAS implementation achieves a similar SNR to that obtained with

pulsed PAS with 20 nJ pulse energies after accounting for wavelength multiplexing. Existing fiber-coupled OPO-based PAS systems can provide up to 20× more pulse energy and a correspondingly

higher SNR. However, given the scaling arguments above, the use of higher repetition rate combs could improve DCPAS SNR by a factor of 55×. Therefore, an improved DCPAS implementation would

be competitive with PAS systems that use tunable pulsed lasers while enabling many spectral elements to be acquired simultaneously without the extra time and potential systematics introduced

by spectral scanning. Finally, DCPAS would also benefit from a more accurate normalization procedure to remove systematic wavelength-dependent variations in the PA response. Our

normalization procedure assumes that optical scattering, sample heating, pressure wave generation, and pressure wave propagation are independent of the multi-heterodyne frequencies in the

optical intensity. Any frequency-dependent effects in these processes would skew the inferred absorption spectrum because of the coupling between ultrasonic and optical frequencies

delineated by Eq. (2). One could address this problem by developing a model for the frequency dependence of PA signal generation under dual-comb excitation. This frequency dependence would

be included in the _β_ proportionality factor in Eq. (4). Alternatively, one could use a reference sample with a known (and ideally flat) absorption spectrum to characterize the frequency

response of the DCPAS system23. In applications where it is not necessary to determine quantitative absorption spectra, these issues might be completely circumvented by applying principal

component analysis to detect specific substances in DCPAS measurements8. Another limitation of the normalization procedure used here is that the optical excitation spectrum is only measured

before the light hits the sample. This could be improved by using optical inversion calculations to obtain the optical spectrum as a function of depth. In that case, one could use a

depth-resolved version of Eq. (4) to normalize the PA response64. Overall, the results demonstrated here show that dual-comb excitation can generate PA signals. These PA signals, in turn,

reflect the absorption spectra of sample materials and can be used to detect and identify different organic solids. Furthermore, we propose several strategies to increase DCPAS acquisition

speeds and to develop more accurate normalization procedures. With these improvements, DCPAS could become a useful new technology for high-speed, label-free spectroscopy and possibly

imaging. The authors would like to acknowledge a recent preprint demonstrating DCPAS for detection of gaseous acetylene65. This shows yet another potential application of DCPAS. METHODS

SAMPLES We used three different samples for DCPAS in this work. One sample consists of a ≈1 mm × 2 mm film of vertically aligned carbon nanotubes (VACNTs) embedded in an ≈240 μm thick film

of poly(dimethylsiloxane) (PDMS). The VACNTs are multi-walled CNTs with an estimated tube diameter of ≈10 nm to ≈20 nm based on measurements of similarly prepared samples. The thickness of

the VACNT layer varies from ≈15 μm to ≈40 μm (see Supplementary Fig. 4). The PDMS film is ≈2.5 mm wide and ≈5 mm long, with several millimeter-wide regions that contain no VACNTs (see

Supplementary Fig. 5). One of these regions is used as a second PA sample that consists of only PDMS. The third sample is a piece of paraffin-based paper (Parafilm “M”, ≈120 μm thick) cut

into a ≈5 mm × 5 mm square (the use of trade names is necessary to specify the experimental results and cannot imply endorsement by the National Institute of Standards and Technology.). Each

of these samples is laminated onto the top of a N-BK7 wedge by gently applying pressure with a pair of tweezers. The wedge is sealed against an opening in the bottom of a water bath so that

the sample is immersed in the water (location (v) in Fig. 2) and oriented toward the transducer. The distance from the sample to the transducer is equal to the transducer focal length of

approximately 2 cm. We also measured the optical absorption spectra of the PDMS and paraffin in transmission-mode with a NIR spectrophotometer (Perkin-Elmer Lambda 1050 equipped with a

3-detector module [The use of trade names is necessary to specify the experimental results and cannot imply endorsement by the National Institute of Standards and Technology]) for comparison

with the spectra obtained in the DCPAS system. The same PDMS sample was used for DCPAS and spectrophotometer measurements. The spectrophotometer illuminated a portion of the PDMS sample

that contained no VACNTs; however, this illumination was not in the exact same location as the dual-comb excitation. The paraffin sample used for DCPAS could not be used for

spectrophotometer measurements because it displayed too much optical scattering for transmission measurements. For transmission measurements, we reduced the optical scattering by placing the

paraffin between two glass microscope slides, heating the glass to 70° C, and applying pressure. Spectrophotometer measurements of this sample were made after allowing it to cool to room

temperature. DCPAS SYSTEM DESIGN Our DCPAS system uses two self-referenced fiber frequency combs with repetition rates, _f_rep, of ≈160 MHz, described in more detail in refs. 44 and 66. Each

comb emits ≈40 mW of light centered at 192 THz (1560 nm) with a 3-dB bandwidth of ≈45 nm. This output is filtered with a 1550 nm ± 5 nm bandpass filter and then amplified to ≈400 mW with a

triple-pumped erbium-doped fiber amplifier. After amplification, the light from each comb is spectrally broadened in ≈3 cm of highly nonlinear fiber with a dispersion of ≈2.2 ps nm−1 km−1.

This yields ≈60 mW of light from each comb spanning 158 THz to 187 THz (location i in Fig. 2). This 158 THz to 187 THz light from each comb is combined on a beam-splitter, transmitted

through a 187 THz low-pass filter and a linear polarizer, and coupled into single-mode PM1550 fiber (location ii in Fig. 2) for transport to the PA setup. The light is then launched into

free space by a _f_ = 50.8 mm reflective collimator. About 88% of the light is transmitted through a CaF2 wedge and ≈6% is reflected by both the front and back faces. The back reflection is

coupled into single-mode fiber and detected by an extended InGaAs photodiode (location (iii) in Fig. 2) to record the optical excitation spectrum. The front reflection is coupled into a

single-mode fiber delay line, for ≈100 μs of delay. Then it is detected by a standard InGaAs photodiode to provide a trigger for the FPGA data acquisition system. The ≈25 mW of light

transmitted through the CaF2 wedge is focused by a _f_ = 45 mm achromatic lens to a spot size of 4 μm on the sample to generate the PA signal. Absorption by the sample generates pressure

waves that are detected by a polytetrafluoride ultrasound transducer with a frequency response that is peaked at 7.5 MHz with a ≈7.5 MHz bandwidth (Supplementary Note 2) and a focal length

of 2 cm (Olympus Panametrics A320S [the use of trade names is necessary to specify the experimental results and cannot imply endorsement by the National Institute of Standards and

Technology]). This transducer is mounted on a 3-D translation stage and positioned to maximize the PA signal, which occurs when the transducer focus overlaps the optical focus of the

incident comb light. In addition, the sample is translated vertically to place it at the common focal plane of the comb light and transducer. As there is 2 cm (transducer focal length) of

water between the sample and the transducer and because the absorption coefficient of water is _μ_A > 6.5 cm−1 for the optical frequencies in this experiment, no light reaches the face of

the transducer. We have also verified that when the sample is translated out of the common focus of the illumination and transducer, no signal is measured. After the ultrasound transducer,

the PA signal is amplified by ≈80 dB and filtered with a 15 MHz low-pass filter before it is input to the data acquisition system, as shown in Fig. 2. The optical excitation signal is

filtered with a 50 MHz low-pass filter after the photodetector and before the data acquisition system. The PA signal and the optical excitation signal are digitized on separate channels of

the data acquisition system, and the resulting time-domain signals are interferograms, as shown in Fig. 3a, b. For the SNR calculations used in Fig. 5, the time-domain SNR is defined as the

peak height of the interferogram divided by the standard deviation of the noise outside of the interferogram centerburst (see Supplementary Fig. 2 and Supplementary Note 1). As the SNR is

low for the weakly absorbing polymer samples, multiple interferograms are coherently averaged in real-time as is common in DCS34,45. We implement this signal averaging on a data acquisition

system that consists of a multi-channel 14-bit analog-digital converter and a field-programmable gate array (FPGA). The firmware in the FPGA removes residual phase noise from the

interferograms before co-adding them for long-term averaging34,45. To measure slow phase drifts, the phase correction firmware requires a stronger signal than is present in a single PA

interferogram. We resolve this issue by adding the optical DCS voltage to the DCPAS voltage as a delayed trigger signal as shown in Fig. 2. DATA AVAILABILITY Data and processing scripts are

available on request from the authors. REFERENCES * Beard, P. Biomedical photoacoustic imaging. _Interface Focus_ 1, 602–631 (2011). Article  PubMed  PubMed Central  Google Scholar  * Wang,

L. V. & Hu, S. Photoacoustic tomography: in vivo imaging from organelles to organs. _Science_ 335, 1458–1462 (2012). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Drexler,

W. et al. Optical coherence tomography today: speed, contrast, and multimodality. _J. Biomed. Opt._ 19, 071412 (2014). Article  ADS  PubMed  Google Scholar  * Hui, J. et al. Bond-selective

photoacoustic imaging by converting molecular vibration into acoustic waves. _Photoacoustics_ 4, 11–21 (2016). Article  PubMed  PubMed Central  Google Scholar  * Zhang, H. F., Maslov, K.,

Stoica, G. & Wang, L. V. Functional photoacoustic microscopy for high-resolution and noninvasive in vivo imaging. _Nat. Biotechnol._ 24, 848–851 (2006). Article  CAS  PubMed  Google

Scholar  * Dasa, M. K., Markos, C., Janting, J. & Bang, O. Multispectral photoacoustic sensing for accurate glucose monitoring using a supercontinuum laser. _J. Opt. Soc. Am. B_ 36,

A61–A65 (2019). Article  ADS  CAS  Google Scholar  * Ghazaryan, A., Ovsepian, S. V. & Ntziachristos, V. Extended near-infrared optoacoustic spectrometry for sensing physiological

concentrations of glucose. _Front. Endocrinol._ 9, 112 (2018). Article  Google Scholar  * Pleitez, M. A. et al. In vivo Noninvasive monitoring of glucose concentration in human epidermis by

mid-infrared pulsed photoacoustic spectroscopy. _Anal. Chem._ 85, 1013–1020 (2013). Article  CAS  PubMed  Google Scholar  * Taruttis, A. & Ntziachristos, V. Advances in real-time

multispectral optoacoustic imaging and its applications. _Nat. Photonics_ 9, 219–227 (2015). Article  ADS  CAS  Google Scholar  * Wang, L. V. & Yao, J. A practical guide to photoacoustic

tomography in the life sciences. _Nat. Methods_ 13, 627–638 (2016). Article  CAS  PubMed  PubMed Central  Google Scholar  * Dasa, M. K. et al. High-pulse energy supercontinuum laser for

high-resolution spectroscopic photoacoustic imaging of lipids in the 1650-1850 nm region. _Biomed. Opt. Express_ 9, 1762–1770 (2018). Article  CAS  PubMed  PubMed Central  Google Scholar  *

Wu, M., Jansen, K., van der Steen, A. F. W. & van Soest, G. Specific imaging of atherosclerotic plaque lipids with two-wavelength intravascular photoacoustics. _Biomed. Opt. Express_ 6,

3276–3286 (2015). Article  CAS  PubMed  PubMed Central  Google Scholar  * Wang, P., Wang, H.-W., Sturek, M. & Cheng, J.-X. Bond-selective imaging of deep tissue through the optical

window between 1600 and 1850 nm. _J. Biophotonics_ 5, 25–32 (2012). Article  PubMed  Google Scholar  * Hudson, S. V. et al. Targeted noninvasive imaging of EGFR-expressing orthotopic

pancreatic cancer using multispectral optoacoustic tomography. _Cancer Res._ 74, 6271–6279 (2014). Article  CAS  PubMed  PubMed Central  Google Scholar  * Li, R. et al. Assessing breast

tumor margin by multispectral photoacoustic tomography. _Biomed. Opt. Express_ 6, 1273–1281 (2015). Article  PubMed  PubMed Central  Google Scholar  * Wu, W., Wang, P., Cheng, J.-X. &

Xu, X.-M. Assessment of white matter loss using bond-selective photoacoustic imaging in a rat model of contusive spinal cord injury. _J. Neurotrauma_ 31, 1998–2002 (2014). Article  PubMed 

PubMed Central  Google Scholar  * Wang, X., Xie, X., Ku, G., Wang, L. V. & Stoica, G. Noninvasive imaging of hemoglobin concentration and oxygenation in the rat brain using

high-resolution photoacoustic tomography. _J. Biomed. Opt._ 11, 024015 (2006). Article  ADS  PubMed  CAS  Google Scholar  * Fainchtein, R., Stoyanov, B. J., Murphy, J. C., Wilson, D. A.

& Hanley, D. F. Local determination of hemoglobin concentration and degree of oxygenation in tissue by pulsed photoacoustic spectroscopy. _Biomed. Optoacoust._ 3916, 19–33 (2000).

Article  ADS  CAS  Google Scholar  * Shi, J. et al. High-resolution, high-contrast mid-infrared imaging of fresh biological samples with ultraviolet-localized photoacoustic microscopy. _Nat.

Photonics_ 13, 609–615 (2019). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar  * Tzoumas, S., Nunes, A., Deliolanis, N. C. & Ntziachristos, V. Effects of multispectral

excitation on the sensitivity of molecular optoacoustic imaging. _J. Biophotonics_ 8, 629–637 (2015). Article  PubMed  Google Scholar  * Taruttis, A., Claussen, J., Razansky, D. &

Ntziachristos, V. Motion clustering for deblurring multispectral optoacoustic tomography images of the mouse heart. _J. Biomed. Opt._ 17, 016009 (2012). Article  ADS  PubMed  Google Scholar

  * Lloyd, L. B., Riseman, S. M., Burnham, R. K., Eyring, E. M. & Farrow, M. M. Fourier transform photoacoustic spectrometer. _Rev. Sci. Instrum._ 51, 1488–1492 (1980). Article  ADS  CAS

  Google Scholar  * Teng, Y. C. & Royce, B. S. H. Quantitative Fourier transform IR photoacoustic spectroscopy of condensed phases. _Appl. Opt._ 21, 77–80 (1982). Article  ADS  CAS 

PubMed  Google Scholar  * Busse, G. & Bullemer, B. Use of the opto-acoustic effect for rapid scan Fourier spectroscopy. _Infrared Phys._ 18, 255–256 (1978). Article  ADS  CAS  Google

Scholar  * Farrow, M. M., Burnham, R. K. & Eyring, E. M. Fourier‐transform photoacoustic spectroscopy. _Appl. Phys. Lett._ 33, 735–737 (1978). Article  ADS  Google Scholar  * Diddams, S.

A. The evolving optical frequency comb [Invited]. _J. Opt. Soc. Am. B_ 27, B51–B62 (2010). Article  CAS  Google Scholar  * Ye, J. & Cundiff, S. T. (eds). _Femtosecond Optical Frequency

Comb: Principle, Operation, and Applications_. (Springer Science & Business Media, Boston, 2005). * Newbury, N. R. Searching for applications with a fine-tooth comb. _Nat. Photonics_ 5,

186–188 (2011). Article  ADS  CAS  Google Scholar  * Truong, G.-W. et al. Accurate frequency referencing for fieldable dual-comb spectroscopy. _Opt. Express_ 24, 30495–30504 (2016). Article

  ADS  CAS  PubMed  Google Scholar  * Karhu, J. et al. Broadband photoacoustic spectroscopy of 14CH4 with a high-power mid-infrared optical frequency comb. _Opt. Lett._ 44, 1142–1145 (2019).

Article  ADS  CAS  PubMed  Google Scholar  * Sadiek, I., Mikkonen, T., Vainio, M., Toivonen, J. & Foltynowicz, A. Optical frequency comb photoacoustic spectroscopy. _Phys. Chem. Chem.

Phys._ 20, 27849–27855 (2018). Article  CAS  PubMed  Google Scholar  * Coddington, I., Newbury, N. & Swann, W. Dual-comb spectroscopy. _Optica_ 3, 414–426 (2016). Article  ADS  Google

Scholar  * Okubo, S. et al. Ultra-broadband dual-comb spectroscopy across 1.0–1.9 µm. _Appl. Phys. Express_ 8, 082402 (2015). Article  ADS  CAS  Google Scholar  * Ycas, G. et al.

High-coherence mid-infrared dual-comb spectroscopy spanning 2.6 to 5.2 μm. _Nat. Photonics_ 12, 202–208 (2018). Article  ADS  CAS  Google Scholar  * Muraviev, A. V., Smolski, V. O., Loparo,

Z. E. & Vodopyanov, K. L. Massively parallel sensing of trace molecules and their isotopologues with broadband subharmonic mid-infrared frequency combs. _Nat. Photonics_ 12, 209–214

(2018). Article  ADS  CAS  Google Scholar  * Waxman, E. M. et al. Intercomparison of open-path trace gas measurements with two dual frequency comb spectrometers. _Atmos. Meas. Tech._ 10,

3295–3311 (2017). Article  ADS  PubMed  PubMed Central  Google Scholar  * Ideguchi, T., Poisson, A., Guelachvili, G., Picqué, N. & Hänsch, T. W. Adaptive real-time dual-comb

spectroscopy. _Nat. Commun._ 5, 3375 (2014). Article  ADS  PubMed  PubMed Central  CAS  Google Scholar  * Klocke, J. L. et al. Single-shot sub-microsecond mid-infrared spectroscopy on

protein reactions with quantum cascade laser frequency combs. _Anal. Chem._ 90, 10494–10500 (2018). Article  CAS  PubMed  Google Scholar  * Ideguchi, T. et al. Coherent Raman spectro-imaging

with laser frequency combs. _Nature_ 502, 355–358 (2013). Article  ADS  CAS  PubMed  Google Scholar  * Ideguchi, T. et al. Microfluidic single-particle chemical analyzer with dual-comb

coherent Raman spectroscopy. _Opt. Lett._ 43, 4057–4060 (2018). Article  ADS  CAS  PubMed  Google Scholar  * Schmid, T. Photoacoustic spectroscopy for process analysis. _Anal. Bioanal.

Chem._ 384, 1071–1086 (2006). Article  CAS  PubMed  Google Scholar  * Kuthirummal, N. Listening to nanomaterials: photoacoustic spectroscopy. _J. Chem. Educ._ 86, 1238–1240 (2009). Article 

CAS  Google Scholar  * Yao, J. & Wang, L. V. Sensitivity of photoacoustic microscopy. _Photoacoustics_ 2, 87–101 (2014). Article  PubMed  PubMed Central  Google Scholar  * Sinclair, L.

C. et al. Invited article: a compact optically coherent fiber frequency comb. _Rev. Sci. Instrum._ 86, 081301 (2015). Article  ADS  CAS  PubMed  Google Scholar  * Roy, J., Deschênes, J.-D.,

Potvin, S. & Genest, J. Continuous real-time correction and averaging for frequency comb interferometry. _Opt. Express_ 20, 21932–21939 (2012). Article  ADS  PubMed  Google Scholar  *

Lehman, J., Yung, C., Tomlin, N., Conklin, D. & Stephens, M. Carbon nanotube-based black coatings. _Appl. Phys. Rev._ 5, 011103 (2018). Article  ADS  CAS  Google Scholar  * Maslov, K.

& Wang, L. V. Photoacoustic imaging of biological tissue with intensity-modulated continuous-wave laser. _J. Biomed. Opt._ 13, 024006 (2008). Article  ADS  PubMed  Google Scholar  *

Sivaramakrishnan, M., Maslov, K., Zhang, H. F., Stoica, G. & Wang, L. V. Limitations of quantitative photoacoustic measurements of blood oxygenation in small vessels. _Phys. Med. Biol._

52, 1349–1361 (2007). Article  PubMed  Google Scholar  * Cai, D. K., Neyer, A., Kuckuk, R. & Heise, H. M. Optical absorption in transparent PDMS materials applied for multimode

waveguides fabrication. _Opt. Mater._ 30, 1157–1161 (2008). Article  ADS  CAS  Google Scholar  * Turek, I., Tarjányi, N., Martinček, I. & Káčik, D. Effect of mechanical stress on optical

properties of polydimethylsiloxane. _Opt. Mater._ 36, 965–970 (2014). Article  ADS  CAS  Google Scholar  * McGoverin, C. M., Lewis, K., Yang, X., Bostrom, M. P. G. & Pleshko, N. The

contribution of bone and cartilage to the near-infrared spectrum of osteochondral tissue. _Appl. Spectrosc._ 68, 1168–1175 (2014). Article  ADS  CAS  PubMed  PubMed Central  Google Scholar 

* Schliesser, A., Picqué, N. & Hänsch, T. W. Mid-infrared frequency combs. _Nat. Photonics_ 6, 440–449 (2012). Article  ADS  CAS  Google Scholar  * Robertson, C. W. & Williams, D.

Lambert Absorption coefficients of water in the infrared. _J. Opt. Soc. Am._ 61, 1316–1320 (1971). Article  ADS  CAS  Google Scholar  * Newbury, N. R., Coddington, I. & Swann, W. C.

Sensitivity of coherent dual-comb spectroscopy. _Opt. Express_ 18, 7929–7945 (2010). Article  ADS  CAS  PubMed  Google Scholar  * Krainer, L. et al. Tunable picosecond pulse-generating laser

with repetition rate exceeding 10 GHz. _Electron. Lett._ 38, 225–227 (2002). Article  ADS  CAS  Google Scholar  * Bartels, A., Heinecke, D. & Diddams, S. A. Passively mode-locked 10 GHz

femtosecond Ti:sapphire laser. _Opt. Lett._ 33, 1905–1907 (2008). Article  ADS  CAS  PubMed  Google Scholar  * Torres-Company, V. & Weiner, A. M. Optical frequency comb technology for

ultra-broadband radio-frequency photonics. _Laser Photonics Rev._ 8, 368–393 (2014). Article  ADS  Google Scholar  * Carlson, D. R. et al. Ultrafast electro-optic light with subcycle

control. _Science_ 361, 1358–1363 (2018). Article  ADS  CAS  PubMed  Google Scholar  * Pasquazi, A. et al. Micro-combs: a novel generation of optical sources. _Phys. Rep._ 729, 1–81 (2018).

Article  ADS  MathSciNet  CAS  MATH  Google Scholar  * Kippenberg, T. J., Holzwarth, R. & Diddams, S. A. Microresonator-based optical frequency combs. _Science_ 332, 555–559 (2011).

Article  ADS  CAS  PubMed  Google Scholar  * Xue, X., Wang, P.-H., Xuan, Y., Qi, M. & Weiner, A. M. Microresonator Kerr frequency combs with high conversion efficiency: high-efficiency

microcombs. _Laser Photonics Rev._ 11, 1600276 (2017). Article  ADS  Google Scholar  * Karpov, M., Pfeiffer, M. H. P., Liu, J., Lukashchuk, A. & Kippenberg, T. J. Photonic chip-based

soliton frequency combs covering the biological imaging window. _Nat. Commun._ 9, 1146 (2018). Article  ADS  PubMed  PubMed Central  CAS  Google Scholar  * Laser Institute of America.

_American National Standard for Safe Use of Lasers_. (Laser Institute of America, 2014). * Cox, B., Laufer, J. G., Arridge, S. R. & Beard, P. C. Quantitative spectroscopic photoacoustic

imaging: a review. _J. Biomed. Opt._ 17, 061202 (2012). Article  ADS  PubMed  Google Scholar  * Wildi, T., Voumard, T., Brasch, V., Yilmaz, G., & Herr, T. Photo-acoustic dual-frequency

comb spectroscopy. arXiv:2004.04691 (2020). * Herman, D. et al. Femtosecond timekeeping: slip-free clockwork for optical timescales. _Phys. Rev. Appl_ 9, 044002 (2018). Article  ADS  CAS 

Google Scholar  Download references ACKNOWLEDGEMENTS We would like to acknowledge G. Ycas for fruitful discussion and development of FPGA code. We acknowledge helpful comments from E.M.

Waxman and A.S. Kowligy. J.T.F. and A.M.G. both conducted their research while the authors held NRC Research Associateship awards at NIST. AUTHOR INFORMATION Author notes * Gabriel M.

Colacion Present address: Optical Science and Engineering, University of New Mexico, 1313 Goddard, SE, Albuquerque, NM, 87106, USA * Eli V. Hoenig Present address: Pritzker School of

Molecular Engineering, University of Chicago, 5640 South Ellis Avenue, ERC 387, Chicago, IL, 60637, USA * Edgar F. Perez Present address: Institute for Research in Electronics and Applied

Physics, University of Maryland, 8279 Paint Branch Drive, College Park, MD, 20742-3511, USA AUTHORS AND AFFILIATIONS * National Institute of Standards and Technology, Applied Physics

Division, 325 Broadway, Boulder, CO, 80305, USA Jacob T. Friedlein, Esther Baumann, Kimberly A. Briggman, Gabriel M. Colacion, Fabrizio R. Giorgetta, Aaron M. Goldfain, Daniel I. Herman, Eli

V. Hoenig, Jeeseong Hwang, Nathan R. Newbury, Edgar F. Perez, Christopher S. Yung, Ian Coddington & Kevin C. Cossel * Department of Physics, University of Colorado, Boulder, CO, 80309,

USA Esther Baumann, Gabriel M. Colacion, Fabrizio R. Giorgetta, Daniel I. Herman, Eli V. Hoenig & Edgar F. Perez Authors * Jacob T. Friedlein View author publications You can also search

for this author inPubMed Google Scholar * Esther Baumann View author publications You can also search for this author inPubMed Google Scholar * Kimberly A. Briggman View author publications

You can also search for this author inPubMed Google Scholar * Gabriel M. Colacion View author publications You can also search for this author inPubMed Google Scholar * Fabrizio R.

Giorgetta View author publications You can also search for this author inPubMed Google Scholar * Aaron M. Goldfain View author publications You can also search for this author inPubMed 

Google Scholar * Daniel I. Herman View author publications You can also search for this author inPubMed Google Scholar * Eli V. Hoenig View author publications You can also search for this

author inPubMed Google Scholar * Jeeseong Hwang View author publications You can also search for this author inPubMed Google Scholar * Nathan R. Newbury View author publications You can also

search for this author inPubMed Google Scholar * Edgar F. Perez View author publications You can also search for this author inPubMed Google Scholar * Christopher S. Yung View author

publications You can also search for this author inPubMed Google Scholar * Ian Coddington View author publications You can also search for this author inPubMed Google Scholar * Kevin C.

Cossel View author publications You can also search for this author inPubMed Google Scholar CONTRIBUTIONS J.T.F helped design and build the DCPAS system. He also acquired and analyzed the

data and wrote the manuscript. E.B., G.M.C., D.H., E.V.H., E.F.P., and helped design and build the DCPAS system. E.B., K.A.B., F.R.G., J.H., N.R.N., I.C., and K.C.C. provided guidance for

experimental design and interpretation of data. F.R.G. and K.C.C. helped with data analysis. C.S.Y. and J.H. fabricated the PDMS/VACNT sample. A.M.G., K.A.B., and J.T.F. performed the PAS

measurements with the optical parametric oscillator. All authors approve of the submitted version of the manuscript and agree to be personally accountable for his/her own contributions and

to ensure that questions related to the accuracy or integrity of any part of the work, even ones in which he/she was not personally involved, are appropriately investigated, resolved, and

the resolution documented in the literature. CORRESPONDING AUTHOR Correspondence to Kevin C. Cossel. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing interests.

ADDITIONAL INFORMATION PEER REVIEW INFORMATION _Nature Communications_ thanks Frans Harren, Markku Vainio and the other, anonymous, reviewer(s) for their contribution to the peer review of

this work. Peer reviewer reports are available. PUBLISHER’S NOTE Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

SUPPLEMENTARY INFORMATION SUPPLEMENTARY INFORMATION PEER REVIEW FILE RIGHTS AND PERMISSIONS OPEN ACCESS This article is licensed under a Creative Commons Attribution 4.0 International

License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source,

provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons

license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by

statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit

http://creativecommons.org/licenses/by/4.0/. Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Friedlein, J.T., Baumann, E., Briggman, K.A. _et al._ Dual-comb photoacoustic

spectroscopy. _Nat Commun_ 11, 3152 (2020). https://doi.org/10.1038/s41467-020-16917-y Download citation * Received: 27 November 2019 * Accepted: 29 May 2020 * Published: 19 June 2020 * DOI:

https://doi.org/10.1038/s41467-020-16917-y SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry, a shareable link is not

currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative